Methyl acetate was subjected to hydrolyiss in N−HCl at 298K. 5mL of the mixture is withdraw at different intervals and titrated with constant with about N/8NaOH. The following results were obtained:
∣∣∣Time (min)0254061∞Vol of alkali used (mL)19.2424.2026.6029.3242.03∣∣∣
Show that the reaction is of first order.
To determine whether the hydrolysis of methyl acetate in N-HCl is a first-order reaction, we will use the data provided and the first-order rate equation. Here’s how we can approach the problem step by step:
Step 1: Understand the First-Order Rate Equation
The first-order rate equation can be expressed as:
k=2.303tlog(V∞−V0V∞−Vt)
Where:
- k = rate constant
- t = time (in minutes)
- V∞ = volume of alkali used at infinite time
- V0 = initial volume of alkali used (at time 0)
- Vt = volume of alkali used at time t
Step 2: Identify the Values from the Data
From the provided data:
- V∞=42.03mL (volume at infinite time)
- V0=19.24mL (volume at time 0)
- Volumes at different times:
- At t=25min, Vt=24.20mL
- At t=40min, Vt=26.60mL
- At t=61min, Vt=29.32mL
Step 3: Calculate the Rate Constant k for Each Time Interval
We will calculate k for each time interval using the first-order rate equation.
For t=25min:
k=2.30325log(42.03−19.2442.03−24.20)
Calculating:
k=2.30325log(22.7917.83)
=2.30325log(1.278)
=2.30325×0.107
=0.0098min−1
For t=40min:
k=2.30340log(42.03−19.2442.03−26.60)
Calculating:
k=2.30340log(22.7915.43)
=2.30340log(1.477)
=2.30340×0.169
=0.0098min−1
For t=61min:
k=2.30361log(42.03−19.2442.03−29.32)
Calculating:
k=2.30361log(22.7912.71)
=2.30361log(1.794)
=2.30361×0.253
=0.0098min−1
Step 4: Conclusion
Since the calculated rate constants k for all time intervals are approximately equal (0.0098 min−1), we can conclude that the reaction is a first-order reaction.
The first-order rate equation can be expressed as:
k=2.303tlog(V∞−V0V∞−Vt)
Where:
- k = rate constant
- t = time (in minutes)
- V∞ = volume of alkali used at infinite time
- V0 = initial volume of alkali used (at time 0)
- Vt = volume of alkali used at time t
From the provided data:
- V∞=42.03mL (volume at infinite time)
- V0=19.24mL (volume at time 0)
- Volumes at different times:
- At t=25min, Vt=24.20mL
- At t=40min, Vt=26.60mL
- At t=61min, Vt=29.32mL
We will calculate k for each time interval using the first-order rate equation.
k=2.30325log(42.03−19.2442.03−24.20)
Calculating:
k=2.30325log(22.7917.83)
=2.30325log(1.278)
=2.30325×0.107
=0.0098min−1
k=2.30340log(42.03−19.2442.03−26.60)
Calculating:
k=2.30340log(22.7915.43)
=2.30340log(1.477)
=2.30340×0.169
=0.0098min−1
k=2.30361log(42.03−19.2442.03−29.32)
Calculating:
k=2.30361log(22.7912.71)
=2.30361log(1.794)
=2.30361×0.253
=0.0098min−1
Since the calculated rate constants k for all time intervals are approximately equal (0.0098 min−1), we can conclude that the reaction is a first-order reaction.
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Solved Example
52 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Ex 4.1 (Objective)
9 Videos
- CARBOXYLIC ACIDS AND THEIR DERIVATIVESBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CARBOXYLIC ACIDS AND THEIR DERIVATIVESEXERCISE - Exercises Archives (Analytical And Descriptive)
34 Videos
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Archives Subjective
18 Videos
Similar Questions
In the dehydration of oxalic acid by conc H2SO4
(COOH)2→CO+CO2+H2O
2mL of the solution was withdraw at different times and titrated against 0.1NKMnO4 solution. Show that the reaction is of first order.
∣∣∣Time (mins)0300450600Vol ofKMnO4used (mL)22.017.015.013.4∣∣∣
Note: This is an example of indirect estimation of reactant, i.e., titrating of oxalic acid by KMnO4.
View SolutionIn an experiment on the decomposition of an aqueous NH4NO2 at a certain temperature, the volume of nitrogen gas collected at different intervals of time was as follows:
∣∣∣Time (s)11902300∞Vol ofN2(mL)5.007.309.50∣∣∣
Show how the above data illustrate that the reaction is of first order.
Note: This is an example of direct-estimation of Product, i.e., N2.
View SolutionThe kinetics of hydrolysis of methyl acetate in excess of hydrochloric acid solution at 298K were followed by withdrawing 2 mL of the reaction mixture at intervals of time (t), adding 50 mL of water and titrating against baryta-water. The following results were obtained :
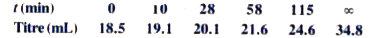
Determine the velocity constant of the hydrolysis.
View SolutionThe kinetics of hydrolysis of methyl acetate in excess of hydrochloric acid solution at 298K were followed by withdrawing 2 mL of the reaction mixture at intervals of time (t), adding 50 mL of water and titrating against baryta-water. The following results were obtained :
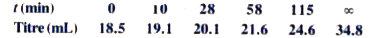
Determine the velocity constant of the hydrolysis.
View SolutionThe kinetics of hydrolysis of methyl acetate in excess of hydrochloric acid solution at 298K were followed by withdrawing 2 mL of the reaction mixture at intervals of time (t), adding 50 mL of water and titrating against baryta-water. The following results were obtained :
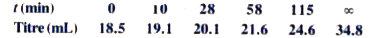
Determine the velocity constant of the hydrolysis.
View SolutionThe hydrolyiss of methyl acetate in aqueous solution is has been studied by titrating the liberated acetic acid against NaOH. The concentration of ester at different times is given below:
∣∣∣t (min)0306090C(MolL−1)0.85000.80040.75380.7096∣∣∣
Show that it follows a pseudo first order reaction, as the concentration of water remains nearly constant (55molL−1) during the course of the reaction . What is the value of k' in the equation ?
Rate =k'[CH3COOCH3][H2O]
View Solution1.0mL of ethyl acetate was added to 25mL of N/2HCl,2mL of the mixture were withdraw form time to time during the progress of the hydrolyiss of the ester and titrated against standard NaOH solution. The amount of NaOH required for titration at various intervals is given below:
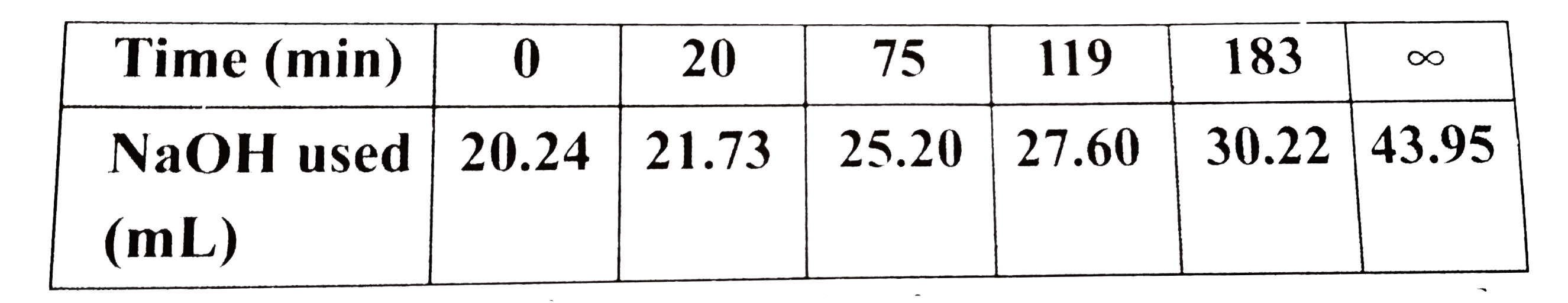
The value at ∞ time was obtained by completing the hydrolyiss on boiling. Show that it is a reaction of the first order and find the average value of the velocity constant.
View SolutionThe following data were reported for the decompoistion of N2O5 in CCl4 at 303K:
∣∣∣Time (min)120160200240∞Vol ofO2(mL)37.7045.8552.6758.3484.35∣∣∣
Show that the reaction is the first order and calculate the rate constant.
Note: This is an example of direct-estimation of Product, i.e., O2.
View SolutionThe first order reaction:
Sucrose → Glucose + Fructose takes place at 308K in 0.5NHCl. At time zero the initial total rotation of the mixture is 32.4∘. After 10min, the total rotation is 28.8∘. If the rotation of sucrose per mole is 85∘, that of glucose is 7.4∘, and of fructose is −86.04∘, calculate the half life of the reaction.
View SolutionFor the hydrolysis of methyl acetate in aqueous solution, the following results were obtained :
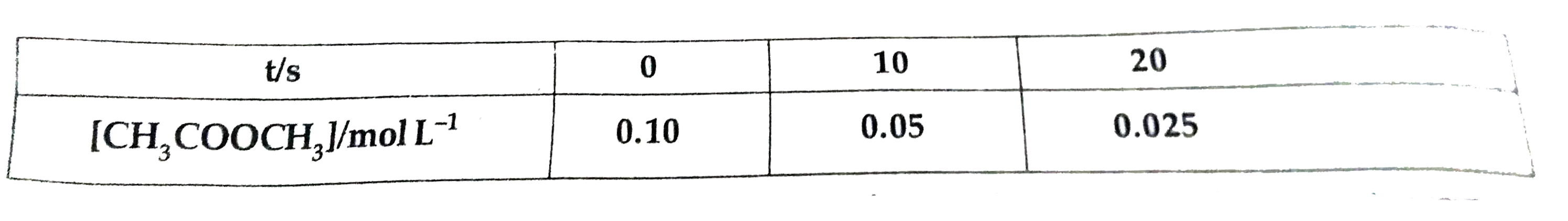
(a) Show that it follows pseudo first order reaction, as the concentration of water remains constant.
(b) Calculate the average rate of reaction between the time interval 10 to 20 seconds. (Given : log 2 = 0.3010, log 4 = 0.6021)
View Solution
