For the reaction: aA+bB→cC+dD
Rate =dxdt=−1ad[A]dt=−1bd[B]dt=1cd[C]dt=1dd[D]dt
The rate of formation of SO3 in the following reaction 2SO2+O2→2SO3 is 100g−1min. Hence the rate of disappearance of O2 is
2g−1min
20g−1min
200g−1min
50g−1min
The correct Answer is:B
To solve the problem, we need to analyze the given reaction and the rate of formation of the product SO3. The reaction is:
2SO2+O2→2SO3
1. Identify the Reaction Stoichiometry:
The balanced chemical equation shows that 2 moles of SO2 react with 1 mole of O2 to produce 2 moles of SO3.
2. Write the Rate Expressions:
From the stoichiometry of the reaction, we can write the rate expressions as follows:
Rate=−12d(SO2)dt=−11d(O2)dt=12d(SO3)dt
3. Given Rate of Formation of SO3:
We are given that the rate of formation of SO3 is 100g/min. This can be expressed as:
d(SO3)dt=100g/min
4. Relate the Rate of Formation of SO3 to the Rate of Disappearance of O2:
Using the rate expressions, we can relate the rate of disappearance of O2 to the rate of formation of SO3:
−d(O2)dt=12d(SO3)dt
5. Substitute the Given Rate:
Substitute the known rate of formation of SO3 into the equation:
−d(O2)dt=12×100g/min
6. Calculate the Rate of Disappearance of O2:
−d(O2)dt=50g/min
Therefore, the rate of disappearance of O2 is:
d(O2)dt=−50g/min
Final Answer:
The rate of disappearance of O2 is 50g/min.
---
The balanced chemical equation shows that 2 moles of SO2 react with 1 mole of O2 to produce 2 moles of SO3.
From the stoichiometry of the reaction, we can write the rate expressions as follows:
Rate=−12d(SO2)dt=−11d(O2)dt=12d(SO3)dt
We are given that the rate of formation of SO3 is 100g/min. This can be expressed as:
d(SO3)dt=100g/min
Using the rate expressions, we can relate the rate of disappearance of O2 to the rate of formation of SO3:
−d(O2)dt=12d(SO3)dt
Substitute the known rate of formation of SO3 into the equation:
−d(O2)dt=12×100g/min
−d(O2)dt=50g/min
Therefore, the rate of disappearance of O2 is:
d(O2)dt=−50g/min
The rate of disappearance of O2 is 50g/min.
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Multiple Correct
33 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Single Correct
177 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Ex4.4 Objective
10 Videos
- CARBOXYLIC ACIDS AND THEIR DERIVATIVESBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CARBOXYLIC ACIDS AND THEIR DERIVATIVESEXERCISE - Exercises Archives (Analytical And Descriptive)
34 Videos
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Archives Subjective
18 Videos
Similar Questions
The rate of formation of SO3 in the following reaction: 2SO2+O2→2SO3 is 10gsec−1. The rate of disappearance of O2 will be
View SolutionFor the reaction: aA+bB→cC+dD
Rate =dxdt=−1ad[A]dt=−1bd[B]dt=1cd[C]dt=1dd[D]dt
A reaction follows the given concentration-time graph. The rate for this reaction at 20s will be
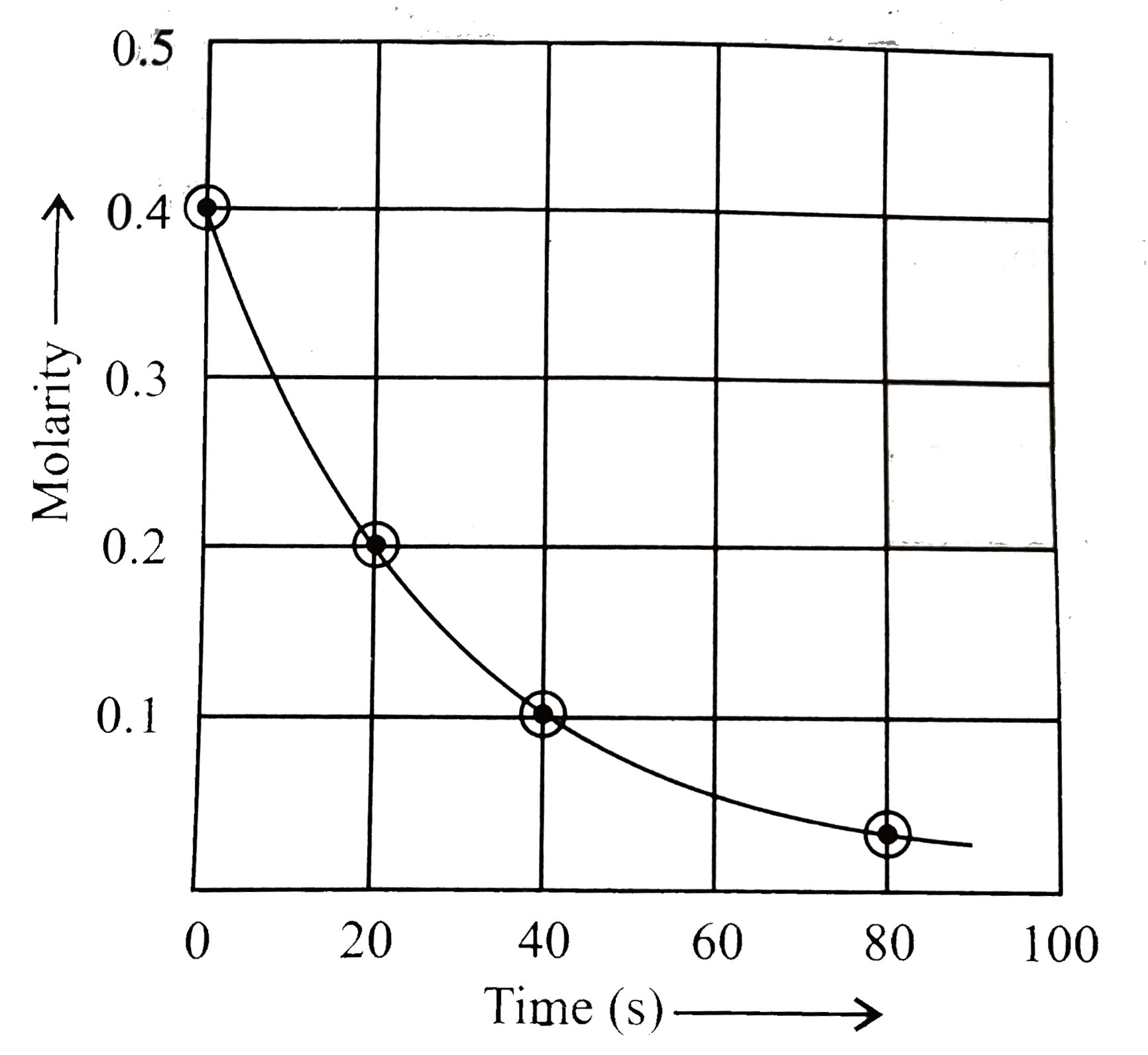
View Solution
Knowledge Check
The rate of disappearance of SO2 in the reaction 2SO2+O2→2SO3 is 1.28×10−3g/sec then the rate of formation of SO3 is
A0.64×10−5gs−1B0.32×10−5gs−1C2.56×10−5gs−1D1.28×10−5gs−1
For the reaction: aA+bB→cC+dD
Rate =dxdt=−1ad[A]dt=−1bd[B]dt=1cd[C]dt=1dd[D]dt
In the following reaction,
xA→yB
log.[−d[A]dt]=log.[d[B]dt]+0.3
where negative isgn indicates rate of disappearance of the reactant. Thus, x:y is:
View SolutionFor the reaction: aA+bB→cC+dD
Rate =dxdt=−1ad[A]dt=−1bd[B]dt=1cd[C]dt=1dd[D]dt
For reaction 3BrOɵ→BrOɵ3+2Brɵ, the value of rate constant at 80∘C in the rate law for −d[BrOɵ]dt was found to be 0.054Lmol−1s−1. The rate constant (k) for the reaction in terms of d[BrOɵ3]dt is
View SolutionThe rate of a reaction is expressed in different ways as follows:
+12d[C]dt=−12d[D]dt=+13d[A]dt=−d[B]dt
the reaction is
View SolutionThe rate of a reaction is expressed in different ways as follows:
+12d[C]dt=−13d[D]dt=+14d[A]dt=−d[B]dt
the reaction is
View SolutionIn a reaction,
aA+bB→cC+dD
rate =−1ad[A]dt , the - ve sign in it represents
View SolutionFor the reaction , 2SO2+O2⇔2SO3, the rate of disappearance of O2 is 2×10−4molL−1s−1 . The rate of appearance of SO3 is
View SolutionHow will the rate of reaction
2SO2(g)+O2(g)→2SO3(g) change if the volume of the reaction vessel is halved?
View Solution
