The reaction 2AX(g)+2B2(g)→A2(g)+2B2X(g) has been studied kinetically and on the baiss of the rate law following mechanism has been proposed.
I. 2AX⇔A2X2 (fast and reverse)
II. A2X2+B2→A2X+B2X
III. A2X+B2→A2+B2X
where all the reaction intermediates are gases under ordinary condition.
form the above mechanism in which the steps (elementary) differ conisderably in their rates, the rate law is derived uisng the principle that the slowest step is the rate-determining step (RDS) and the rate of any step varies as the Product of the molar concentrations of each reacting speacting species raised to the power equal to their respective stoichiometric coefficients (law of mass action). If a reacting species is solid or pure liquid, its active mass, i.e., molar concentration is taken to be unity, the standard state. In qrder to find out the final rate law of the reaction, the concentration of any intermediate appearing in the rate law of the RDS is substituted in terms of the concentration of the reactant(s) by means of the law of mass action applied on equilibrium step.
How many times the rate of formation of A2 will increase if concentrations of AX is doubled and that of B2 is increased theee fold?
36
12
6
8
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Write the Rate Law for the Rate Determining Step (RDS)
The rate law for the second step (which is the slowest step and thus the RDS) can be expressed as:
Rate=k2(A2X2)(B2)
where k2 is the rate constant for the second step.
Step 2: Substitute the Equilibrium Expression from Step 1
From the first step, which is an equilibrium reaction:
2AX⇌A2X2
The equilibrium constant Keq for this step can be expressed as:
Keq=(A2X2)(AX)2
Thus, we can express (A2X2) in terms of (AX):
(A2X2)=Keq(AX)2
Step 3: Substitute into the Rate Law
Now, substituting (A2X2) into the rate law:
Rate=k2⋅Keq(AX)2(B2)
Let’s denote k=k2⋅Keq for simplicity:
Rate=k(AX)2(B2)
Step 4: Calculate the New Rate with Changed Concentrations
If the concentration of AX is doubled and the concentration of B2 is increased threefold, we have:
- New concentration of AX=2(AX)
- New concentration of B2=3(B2)
Substituting these into the rate equation:
New Rate=k(2(AX))2(3(B2))
=k⋅4(AX)2⋅3(B2)
=12k(AX)2(B2)
Step 5: Relate New Rate to Original Rate
The original rate was:
Original Rate=k(AX)2(B2)
Thus, the new rate can be expressed as:
New Rate=12×Original Rate
Conclusion
The rate of formation of A2 will increase by a factor of 12 when the concentration of AX is doubled and the concentration of B2 is increased threefold.
Final Answer
The rate of formation of A2 will increase by 12 times.
---
The rate law for the second step (which is the slowest step and thus the RDS) can be expressed as:
Rate=k2(A2X2)(B2)
where k2 is the rate constant for the second step.
From the first step, which is an equilibrium reaction:
2AX⇌A2X2
The equilibrium constant Keq for this step can be expressed as:
Keq=(A2X2)(AX)2
Thus, we can express (A2X2) in terms of (AX):
(A2X2)=Keq(AX)2
Now, substituting (A2X2) into the rate law:
Rate=k2⋅Keq(AX)2(B2)
Let’s denote k=k2⋅Keq for simplicity:
Rate=k(AX)2(B2)
If the concentration of AX is doubled and the concentration of B2 is increased threefold, we have:
- New concentration of AX=2(AX)
- New concentration of B2=3(B2)
New Rate=k(2(AX))2(3(B2))
=k⋅4(AX)2⋅3(B2)
=12k(AX)2(B2)
The original rate was:
Original Rate=k(AX)2(B2)
Thus, the new rate can be expressed as:
New Rate=12×Original Rate
The rate of formation of A2 will increase by a factor of 12 when the concentration of AX is doubled and the concentration of B2 is increased threefold.
The rate of formation of A2 will increase by 12 times.
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Multiple Correct
33 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Single Correct
177 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Ex4.4 Objective
10 Videos
- CARBOXYLIC ACIDS AND THEIR DERIVATIVESBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CARBOXYLIC ACIDS AND THEIR DERIVATIVESEXERCISE - Exercises Archives (Analytical And Descriptive)
34 Videos
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Archives Subjective
18 Videos
Similar Questions
The reaction 2AX(g)+2B2(g)→A2(g)+2B2X(g) has been studied kinetically and on the baiss of the rate law following mechanism has been proposed.
I. 2AX⇔A2X2 (fast and reverse)
II. A2X2+B2→A2X+B2X
III. A2X+B2→A2+B2X
where all the reaction intermediates are gases under ordinary condition.
form the above mechanism in which the steps (elementary) differ conisderably in their rates, the rate law is derived uisng the principle that the slowest step is the rate-determining step (RDS) and the rate of any step varies as the Product of the molar concentrations of each reacting speacting species raised to the power equal to their respective stoichiometric coefficients (law of mass action). If a reacting species is solid or pure liquid, its active mass, i.e., molar concentration is taken to be unity, the standard state. In qrder to find out the final rate law of the reaction, the concentration of any intermediate appearing in the rate law of the RDS is substituted in terms of the concentration of the reactant(s) by means of the law of mass action applied on equilibrium step.
Let the equilibrium constant of Step I be 2×10−3mol−1L and the rate constants for the formation of A2X and A2 in Step II and III are 3.0×10−2mol−1L−1min and 1×103mol−1L−1min (all data at 25∘C), then what is the overall rate constant (mol−2L2−1min) of the consumption of B2?
View SolutionThe gaseous reaction A(g)+B(g)⇔2C(g)+D(g)+q kJ is most favoured at :
View Solution
Knowledge Check
The reaction ,2AB(g)+2C(g)→A2(g)+2BC(g) proceeds according to the mechanism .
I. 2AB⇔A2B2 (fast)
II. A2B2+C→A2B+BC (slow )
III. A2B+C→A2+BC (fast)
what will be the initial rate taking [AB] = 0.2 M and [C] = 0.5 M ? The Kc for the step I is 102M−1 and rate constant for the step II is 3.0×10−3mol−1−1minA0.0716M−1minB0.0891M−1minC0.006M−1minD0.0257M−1min
In a reaction A2(g)+4B2(g)⇔2AB4(g),ΔH<0. The formation of AB4 is not favoured by
View SolutionFor the Chemical reaction A2(g)+B2(g)⇔2AB(g) the amount of AB at equilibrium is affected by
View Solution2 is the equilibrium constant for the reaction A2+B2⇔2AB at a given temperature. What is the degree of dissociation for A2 or B2
View SolutionConisder the reaction mechanism:
A2keq⇐⇒2A(fast) (where A is the intermediate.)
A+B−−→k1P(slow)
The rate law for the reaction is
View Solution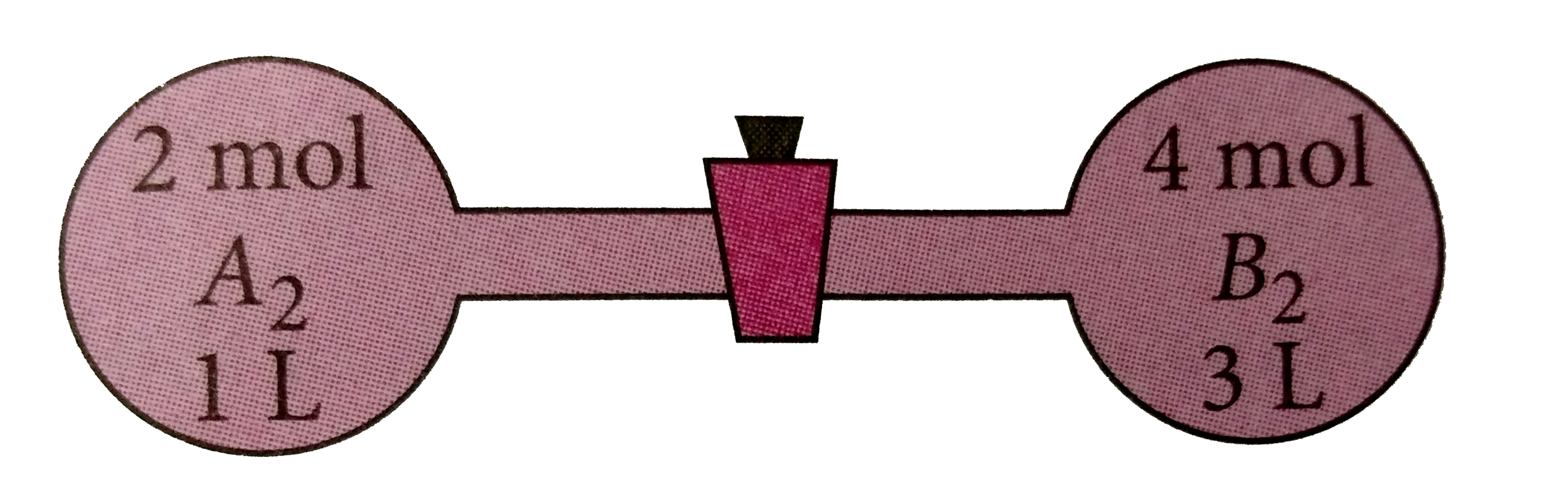
When A2andB2 are allowed to react, the equilibrium constant of the reaction at 27∘C is found (Kc=4).
A2(g)+B2(g)⇔2AB(g)
what will be the equilibrium concentration of AB?
View SolutionIf for 2A2B(g)⇔2A2(g)+B2(g),KP=TOTAL PRESSURE(at equilibrium) and starting the dissociation from" 4 "mol of" A_(2)B then:
View SolutionThe mechanism for the overall reaction is
A2+B→C
A2→2A (slow)
2A+B→X (fast)
If a catalyst D changes the mechanism to
A2+D→A2D (slow)
A2D⇒2A+D (fast)
2A+B→C (fast)
Which is the rate expresison for the reaction with and without a catalyst ?
View Solution
