A first order reaction: A→ Products and a second order reaction: 2R→ Products both have half time of 20min when they are carried out taking 4molL−1 of their respective reactants. The number of mole per litre of A and R remaining unreacted after60min form the start of the reaction, respectively, will be
1 and 0.5M
0.5M and negligible
0.5 and 1M
1 and 0.25M
The correct Answer is:C
To solve the problem, we need to calculate the concentration of reactants A and R after 60 minutes for a first-order and a second-order reaction, respectively.
Step 1: Calculate the concentration of A after 60 minutes (First-order reaction)
1. Identify the half-life of the first-order reaction: The half-life (t₁/₂) is given as 20 minutes.
2. Determine how many half-lives fit into 60 minutes:
- 60 minutes / 20 minutes = 3 half-lives.
3. Calculate the concentration remaining after each half-life:
- Initial concentration of A = 4 mol/L.
- After 1st half-life (20 min): 4 mol/L → 2 mol/L.
- After 2nd half-life (40 min): 2 mol/L → 1 mol/L.
- After 3rd half-life (60 min): 1 mol/L → 0.5 mol/L.
Thus, the concentration of A remaining after 60 minutes is 0.5 mol/L.
Step 2: Calculate the concentration of R after 60 minutes (Second-order reaction)
1. Identify the half-life of the second-order reaction: The half-life (t₁/₂) is also given as 20 minutes.
2. Understand the relationship of half-life in second-order reactions: The half-life for a second-order reaction is inversely proportional to the initial concentration.
- The formula for half-life of a second-order reaction is:
t1/2=1k(A)0
- Since t₁/₂ is constant at 20 minutes for an initial concentration of 4 mol/L, we can calculate the concentration after each half-life.
3. Calculate the concentration remaining after each half-life:
- After the first half-life (20 min):
- Initial concentration = 4 mol/L → After 20 min: 2 mol/L.
- After the second half-life (40 min):
- New t₁/₂ = 40 min (since concentration is now 2 mol/L).
- After 40 min: 2 mol/L → 1 mol/L.
- After the third half-life (60 min):
- New t₁/₂ = 60 min (since concentration is now 1 mol/L).
- After 60 min: 1 mol/L → 0.5 mol/L.
Thus, the concentration of R remaining after 60 minutes is 1 mol/L.
Final Answer:
- Concentration of A after 60 minutes: 0.5 mol/L
- Concentration of R after 60 minutes: 1 mol/L
2. Determine how many half-lives fit into 60 minutes:
- 60 minutes / 20 minutes = 3 half-lives.
3. Calculate the concentration remaining after each half-life:
- Initial concentration of A = 4 mol/L.
- After 1st half-life (20 min): 4 mol/L → 2 mol/L.
- After 2nd half-life (40 min): 2 mol/L → 1 mol/L.
- After 3rd half-life (60 min): 1 mol/L → 0.5 mol/L.
2. Understand the relationship of half-life in second-order reactions: The half-life for a second-order reaction is inversely proportional to the initial concentration.
- The formula for half-life of a second-order reaction is:
t1/2=1k(A)0
- Since t₁/₂ is constant at 20 minutes for an initial concentration of 4 mol/L, we can calculate the concentration after each half-life.
3. Calculate the concentration remaining after each half-life:
- After the first half-life (20 min):
- Initial concentration = 4 mol/L → After 20 min: 2 mol/L.
- After the second half-life (40 min):
- New t₁/₂ = 40 min (since concentration is now 2 mol/L).
- After 40 min: 2 mol/L → 1 mol/L.
- After the third half-life (60 min):
- New t₁/₂ = 60 min (since concentration is now 1 mol/L).
- After 60 min: 1 mol/L → 0.5 mol/L.
- Concentration of A after 60 minutes: 0.5 mol/L
- Concentration of R after 60 minutes: 1 mol/L
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Assertion-Reasoning
22 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Integer
15 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Multiple Correct
33 Videos
- CARBOXYLIC ACIDS AND THEIR DERIVATIVESBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CARBOXYLIC ACIDS AND THEIR DERIVATIVESEXERCISE - Exercises Archives (Analytical And Descriptive)
34 Videos
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Archives Subjective
18 Videos
Similar Questions
For a first order reaction A→ Products, the half life is 100 seconds. The rate constant of the reaction is
View SolutionWhat fraction of a reactant remains unreacted after 40 min if t1/2 is of the reaction is 20 min? consider the reaction is first order
View SolutionTaking the reaction, A+2B→ Products to be of second order, which of the following is/are the correct rate law expresisons (s)?
View SolutionFor a first order for the reaction A→ products : the rate of reaction at [A]=0.2M is 1.0×10−3molL−1s−1. The reaction will occur to 75% completion in .
View SolutionRate of reaction, A+B→ products is given below as a function of different initial concentration of
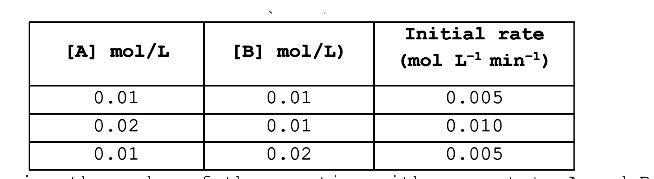
Determine the order of the reaction with respect to A and B. What is the half-life of A in the reaction?
View SolutionFor the reaction A→ Products, it is found that the rate of reaction increases by a factor of 6.25 when concentration of A increases by a factor of 2.5. Calculate the order of reaction with respect to A.
View SolutionThe specific rate (in mol L^(-1) S^(-1)) of a first order reaction having half life of 6.93 seconds is
View SolutionIn a reaction A→ products , when start is made from 8.0×10−2M of A, half life is found to be 120 minute. For the initial concentration 4.0×10−2M, the half life of the reaction becomes 240 minute. The order of the reaction is :
View Solution60% of a first order reaction was completed in 60min. The time taken for reactants to decompose to half of their original amount will be
View SolutionThe half life of a first order reaction is 60min. How long will is take to consume 90% of the reactant?
View Solution
