When the rate determined by the change in concentration of two different reactants, then the kinetic equation may be expressed as
k2=2.303(a−b)tlog.(a−x)b(b−x)a
k2=2.303(a−b)tlog.(a−x)(b+x)
k2=2.303(a−b)tlog.(a−x)(b−x)
k2=1t×x(a−x)
The correct Answer is:A
To solve the question regarding the kinetic equation when the rate is determined by the change in concentration of two different reactants, we can follow these steps:
1. Identify the Reaction:
Consider the reaction:
A+B→Products
Here, A and B are the two different reactants.
2. Define Initial Concentrations:
At time t=0:
- Concentration of A = (A)0=A
- Concentration of B = (B)0=B
- Concentration of products = 0
3. Define Concentrations at Time t:
At time t:
- Concentration of A = (A)=A−x
- Concentration of B = (B)=B−x
- Concentration of products = x
4. Write the Rate Equation:
The rate of the reaction can be expressed as:
Rate=−d(A)dt=−d(B)dt=k(A)(B)
Substituting the expressions for (A) and (B):
Rate=k(A−x)(B−x)
5. Integrate the Rate Equation:
We can express the rate as:
dxdt=k(A−x)(B−x)
Rearranging gives:
dx(A−x)(B−x)=kdt
6. Set Up the Integral:
Integrate both sides:
∫x0dx(A−x)(B−x)=∫t0kdt
7. Perform the Integration:
The left side can be integrated using partial fractions:
1(A−x)(B−x)=1B−A(1A−x−1B−x)
Thus, the integral becomes:
1B−A(ln|A−x|−ln|B−x|)∣∣∣x0=kt
8. Evaluate the Limits:
Evaluating the limits gives:
1B−A(ln|A−x|−ln|B−x|−(ln|A|−ln|B|))=kt
9. Rearranging the Equation:
This can be rearranged to find:
kt=1B−A(ln((A−x)(B−x))+ln(BA))
10. Final Expression:
The final expression can be simplified to:
k=2.303B−A⋅tlog((A−x)(B−x))
Consider the reaction:
A+B→Products
Here, A and B are the two different reactants.
At time t=0:
- Concentration of A = (A)0=A
- Concentration of B = (B)0=B
- Concentration of products = 0
At time t:
- Concentration of A = (A)=A−x
- Concentration of B = (B)=B−x
- Concentration of products = x
The rate of the reaction can be expressed as:
Rate=−d(A)dt=−d(B)dt=k(A)(B)
Substituting the expressions for (A) and (B):
Rate=k(A−x)(B−x)
We can express the rate as:
dxdt=k(A−x)(B−x)
Rearranging gives:
dx(A−x)(B−x)=kdt
Integrate both sides:
∫x0dx(A−x)(B−x)=∫t0kdt
The left side can be integrated using partial fractions:
1(A−x)(B−x)=1B−A(1A−x−1B−x)
Thus, the integral becomes:
1B−A(ln|A−x|−ln|B−x|)∣∣∣x0=kt
Evaluating the limits gives:
1B−A(ln|A−x|−ln|B−x|−(ln|A|−ln|B|))=kt
This can be rearranged to find:
kt=1B−A(ln((A−x)(B−x))+ln(BA))
The final expression can be simplified to:
k=2.303B−A⋅tlog((A−x)(B−x))
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Assertion-Reasoning
22 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Integer
15 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Multiple Correct
33 Videos
- CARBOXYLIC ACIDS AND THEIR DERIVATIVESBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CARBOXYLIC ACIDS AND THEIR DERIVATIVESEXERCISE - Exercises Archives (Analytical And Descriptive)
34 Videos
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Archives Subjective
18 Videos
Similar Questions
Knowledge Check
The increase in concentration of the reactants lead changes in
AΔHBcollision frequencyCactivation energyDequilibrium constant.Assertion : The rate of reaction is the rate of change of concentration of a reactant or a product.
Reason : Rate of reaction remians constant during the complete reaction.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.Compounds 'A' and 'B' react according to the following chemical equation.
A(g)→2B(g)→2C(g)
Concentration of either 'A' or 'B' were changed Keeping the concentration of one of the reactants constant and rates were measured as a function of initial concentration. Following result were obtained.
Choose the correct option for the rate equations for this reaction.
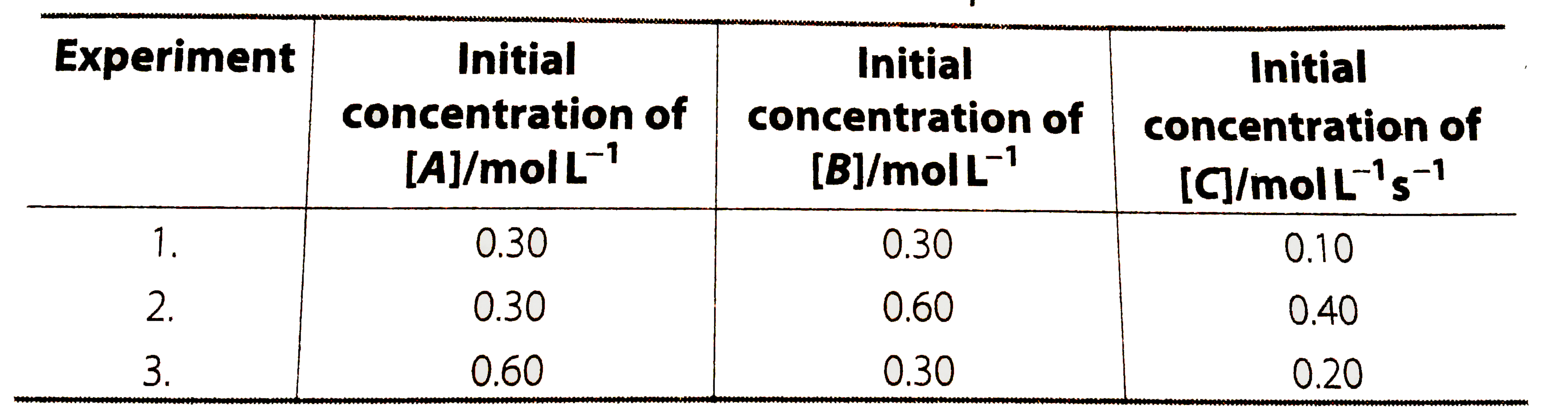 ARate =k[A]2[B]BRate =k[A][B]2CRate =k[A][B]DRate =k[A]2[B]∘
ARate =k[A]2[B]BRate =k[A][B]2CRate =k[A][B]DRate =k[A]2[B]∘
Greater the concentration of reactants, slower the rate of reaction––––––––––––––––––––––––––––.
View SolutionGreater the concentration of reactants, slower the rate of reaction––––––––––––––––––––––––––––.
View SolutionGreater the concentration of reactants, slower the rate of reaction––––––––––––––––––––––––––––.
View SolutionHow concentration of reactants influence the rate of a reaction .
View SolutionIf the concentration of the reactants is increased, the rate of reaction for first order-
View Solution
