A reaction takes place in theee steps: the rate constant are k1,k2,andk3. The overall rate constant k=k1k3k2. If E1,E2, and E3 (energy of activation) are 60,30 and 10kJ, respectively, the overall energy of activation is
40
30
400
60
The correct Answer is:A
To find the overall energy of activation for the reaction that takes place in three steps, we can follow these steps:
Step 1: Understand the given information
We have three steps in the reaction with activation energies:
- E1=60kJ
- E2=30kJ
- E3=10kJ
The overall rate constant is given by the equation:
k=k1k3k2
Step 2: Write the expression for the natural logarithm of the rate constant
Taking the natural logarithm of both sides, we have:
lnk=lnk1+lnk3−lnk2
Step 3: Substitute the Arrhenius equation
According to the Arrhenius equation, we can express the natural logarithm of the rate constants in terms of activation energy:
lnk=−EaRT+lnA
where Ea is the activation energy, R is the gas constant, and T is the temperature.
Step 4: Write the expressions for each rate constant
For each rate constant, we can write:
- For k1:
lnk1=−E1RT+lnA
- For k2:
lnk2=−E2RT+lnA
- For k3:
lnk3=−E3RT+lnA
Step 5: Substitute these expressions into the equation for lnk
Substituting these into the expression for lnk, we get:
lnk=(−E1RT+lnA)+(−E3RT+lnA)−(−E2RT+lnA)
Step 6: Simplify the equation
Combining the terms, we have:
lnk=−E1+E3−E2RT+3lnA
The lnA terms will cancel out when we equate both sides.
Step 7: Rearranging to find overall activation energy
From this, we can see that the overall activation energy Ea can be expressed as:
Ea=E1+E3−E2
Step 8: Substitute the values of activation energies
Now substituting the values:
Ea=60kJ+10kJ−30kJ
Ea=40kJ
Conclusion
The overall energy of activation for the reaction is:
40kJ
We have three steps in the reaction with activation energies:
- E1=60kJ
- E2=30kJ
- E3=10kJ
k=k1k3k2
Taking the natural logarithm of both sides, we have:
lnk=lnk1+lnk3−lnk2
According to the Arrhenius equation, we can express the natural logarithm of the rate constants in terms of activation energy:
lnk=−EaRT+lnA
where Ea is the activation energy, R is the gas constant, and T is the temperature.
For each rate constant, we can write:
- For k1:
lnk1=−E1RT+lnA
- For k2:
lnk2=−E2RT+lnA
- For k3:
lnk3=−E3RT+lnA
Substituting these into the expression for lnk, we get:
lnk=(−E1RT+lnA)+(−E3RT+lnA)−(−E2RT+lnA)
Combining the terms, we have:
lnk=−E1+E3−E2RT+3lnA
The lnA terms will cancel out when we equate both sides.
From this, we can see that the overall activation energy Ea can be expressed as:
Ea=E1+E3−E2
Now substituting the values:
Ea=60kJ+10kJ−30kJ
Ea=40kJ
The overall energy of activation for the reaction is:
40kJ
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Assertion-Reasoning
22 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Integer
15 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Exercises Multiple Correct
33 Videos
- CARBOXYLIC ACIDS AND THEIR DERIVATIVESBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CARBOXYLIC ACIDS AND THEIR DERIVATIVESEXERCISE - Exercises Archives (Analytical And Descriptive)
34 Videos
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Archives Subjective
18 Videos
Similar Questions
A reaction takes place in theee steps: the rate constant are k1,k2,andk3. The overall rate constant k=k1k3/k2. If the energies of activation are 40,30,and20KJmol−1, the overall energy of activation is (assuming A to be constant for all)
View SolutionA reaction takes place in theee steps: the rate constant are k1,k2,andk3. The overall rate constant k=k1k3/k2. If the energies of activation are 40,30,and20KJmol−1, the overall energy of activation is (assuming A to be constant for all)
View SolutionA reaction takes place in various steps. The rate constatn for first, second, third and fifth steps are k1,k2,k3 and k5 respectively The overall rate constant is given by
k=k2k3(k1k5)1/2
If activation energy are 40, 60, 50, and 10 kJ/mol respectively, the overall energy of activation (kJ/mol) is :
View SolutionRate of two reaction whose rate constants arek1&k2 are equal at 300 k such that: So caculate ln(A2)A1=? Ea2−Ea1=2RT.
View SolutionFor a reaction, the rate constant is expressed as k=Ae−40000/T. The energy of the activation is
View SolutionThe rate constant k1 and k2 for two different reactions are 1016e−2000/T and 1015e−1000/T, respectively. The temperature at which k1=k2 is
View SolutionFor reaction A → B , the rate constant K1=A1(e−Ea1/RT) and the reaction X→Y , the rate constant K2=A2(e−Ea2/RT) . If A1=109, A2=1010 and Ea1=1200 cal/mol and Ea2 =1800 cal/mol , then the temperature at which K1=K2 is : (Given , R=2 cal/K-mol)
View SolutionA chemical reaction was carried out at 300 K and 280 K. The rate constants were found to be k1andk2 respectively. Then
View SolutionFor the following elementary first order reaction:
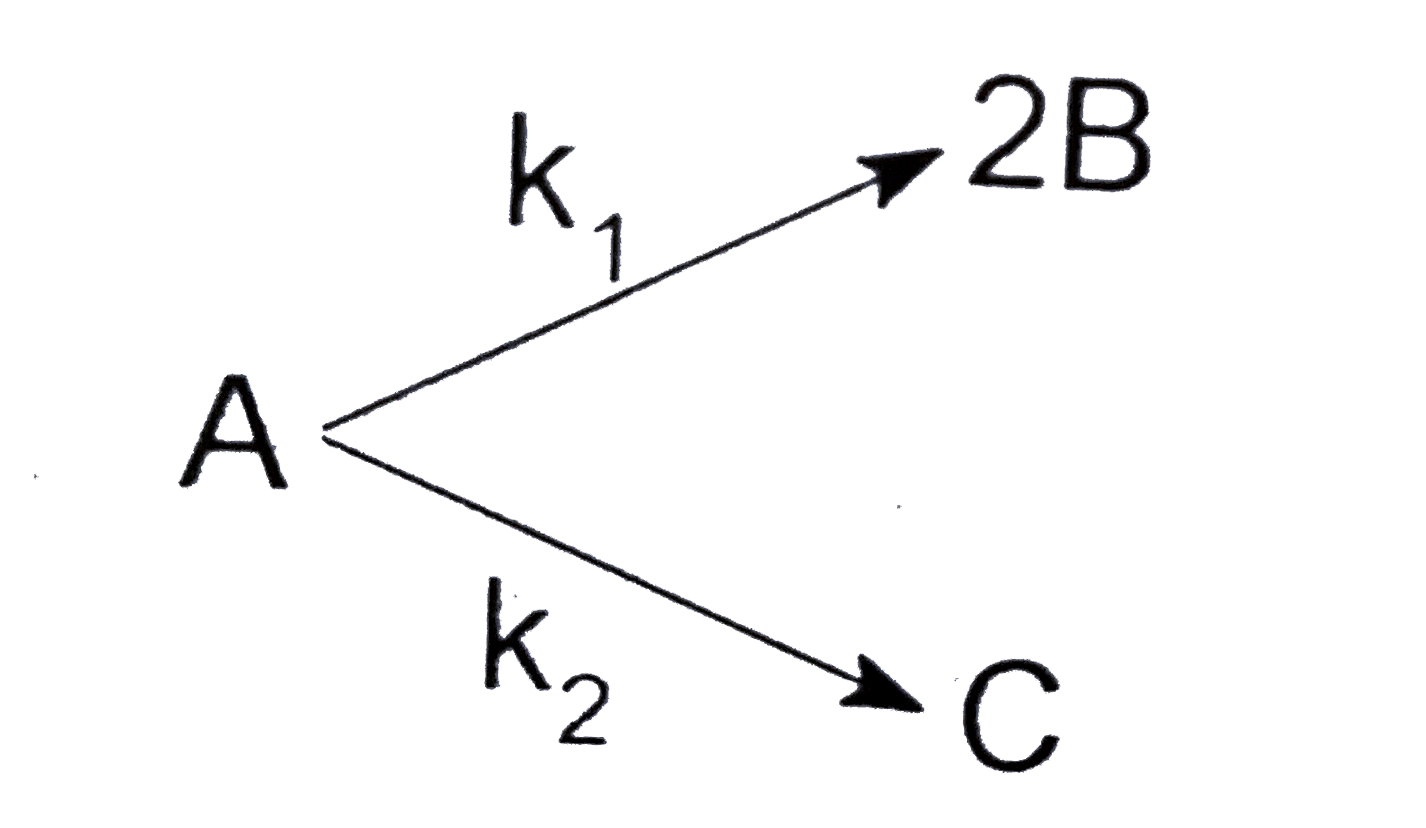
If k2=2k1, then % of B in overall product is:
View SolutionFor a reaction, activation energy (E_a ) =0 and rate constant, k=1.5x104s−1 at 300 K. What is the value of rate constant at 320 K'?
View Solution