The radioactive decay follows zero− order kinetics.
The correct Answer is:F
To solve the question regarding whether the radioactive decay follows zero-order kinetics, we can break down the reasoning step by step.
1. Understanding Radioactive Decay:
- Radioactive decay is a process where an unstable nucleus transforms into a more stable nucleus by emitting radiation (alpha, beta, or gamma particles).
2. Identifying the Rate of Decay:
- In radioactive decay, the rate of disintegration (decay) is dependent on the number of undecayed (unstable) nuclei present.
3. Formulating the Rate Equation:
- The rate of decay can be expressed mathematically as:
−dNdt∝N
where N is the number of undecayed atoms.
4. Understanding the Proportionality:
- This proportionality indicates that the rate of decay is directly proportional to the number of undecayed atoms.
5. Converting Proportionality to an Equation:
- By removing the proportionality, we can write the rate equation as:
−dNdt=kN
where k is the rate constant.
6. Determining the Order of the Reaction:
- The equation −dNdt=kN indicates that the rate of decay is first-order because the rate is proportional to the concentration of the reactant raised to the power of 1.
7. Conclusion:
- Since the rate of radioactive decay is directly proportional to the number of undecayed atoms and follows first-order kinetics, the statement that radioactive decay follows zero-order kinetics is false.
Final Answer:
The statement that the radioactive decay follows zero-order kinetics is false; it actually follows first-order kinetics.
---
- Radioactive decay is a process where an unstable nucleus transforms into a more stable nucleus by emitting radiation (alpha, beta, or gamma particles).
- In radioactive decay, the rate of disintegration (decay) is dependent on the number of undecayed (unstable) nuclei present.
- The rate of decay can be expressed mathematically as:
−dNdt∝N
where N is the number of undecayed atoms.
- This proportionality indicates that the rate of decay is directly proportional to the number of undecayed atoms.
- By removing the proportionality, we can write the rate equation as:
−dNdt=kN
where k is the rate constant.
- The equation −dNdt=kN indicates that the rate of decay is first-order because the rate is proportional to the concentration of the reactant raised to the power of 1.
- Since the rate of radioactive decay is directly proportional to the number of undecayed atoms and follows first-order kinetics, the statement that radioactive decay follows zero-order kinetics is false.
The statement that the radioactive decay follows zero-order kinetics is false; it actually follows first-order kinetics.
Topper's Solved these Questions
- NUCLEAR CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Archives Linked Comprehension
3 Videos
- NUCLEAR CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Archives Multiple Correct
3 Videos
- NUCLEAR CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Exercise Fill In The Blanks
30 Videos
- NCERT BASED EXERCISEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NCERT BASED EXERCISEEXERCISE - Nuclear Chemistry (NCERT Exercise)
29 Videos
- ORGANIC COMPOUNDS WITH FUNCTIONAL GROUPBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ORGANIC COMPOUNDS WITH FUNCTIONAL GROUPEXERCISE - Archives Analytical And Descriptive
24 Videos
Similar Questions
The decay of a radioactive element follows first order kinetic. Thus,
View SolutionFirst Order Kinetics
View SolutionA substance with initial concentration 'a' follows zero order kinetics. In how much time, will the reactions go to completion ?
View SolutionWhich order does radioactive decay follow?
View SolutionThe radioactive decay rate of a radioactive element is found to be 103 disintegration per second at a certain time . If the half life of the element is one second , the decay rate after one second is?
View SolutionThe unit of radioactivity decay rate is
View SolutionReactant 'A' (initial concentration, a) reacts according to zero order kinetics, the time taken for the completion of the reaction is
View SolutionWhy do radioactive element decay?
View SolutionConsider radioactive decay of A to B with which further decays either to X or Y, λ1,λ2 and λ3 are decay constant for A to B decay, B to X decay and Bto Y decay respectively. At t=0, the number of nuclei of A,B,X and Y are N0,N0 zero and zero respectively.
N1,N2,N3 and N4 are the number of nuclei of A,B,X and Y at any instant t.
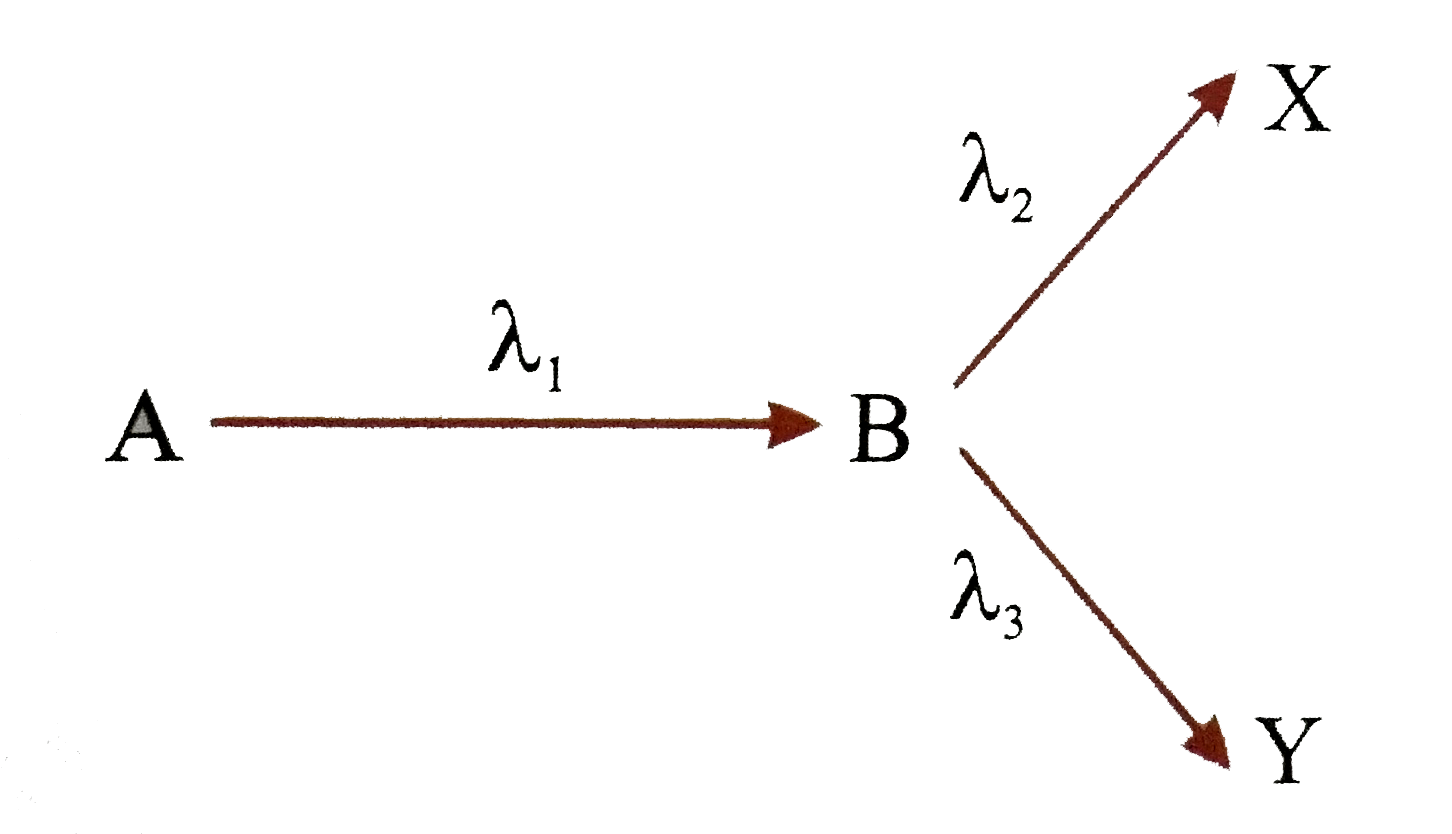
The net rate of accumulation of B at any instant is
View SolutionA radioactive element X decays to give two inert gasesX is
View Solution
