Vector →A of magnitude 4 units is directed along the positive x-axis. Another vector →B of magnitude 3 units lies in x-y plane and is directed along 30∘ with the positive x-axis in anticlockwise direction. Then →A×→B is
6ˆj
6ˆk
2ˆi+4ˆj
None of these
The correct Answer is:B
Topper's Solved these Questions
Similar Questions
Vector →A of magnitude 4 units is directed along the positive x-axis. Another vector →B of magnitude 3 units lies in the x-y plane and is directed along 30∘ with the positive x-axis is as shown in figure. The magnitude of dot product →A.→B is:
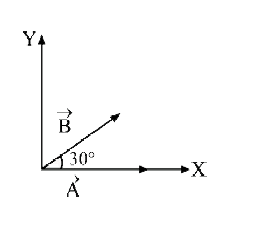
View SolutionWrite down a unit vector in XY-plane, making an angle of 30° with the positive direction of x-axis.
View Solution
Knowledge Check
A vector of magnitude 14 lies in the xy-plane and makes an angle of 60∘ with x -axis . The components of the vector in the direction of x -axis and y-axis are
A7,7√3B7√3,7C14√3,14/√3D14/√3,14√3If →A is a vector of magnitude 4 units due east. What is the magnitude and direction of a vector −4→A ?
A4 units due eastB8 units due eastC16 units due eastD16 units due west
Write down a unit vector in XY-plane, making an angle of 30° with the positive direction of x-axis.
View SolutionWrite down a unit vector in XY-plane, making an angle of 30° with the positive direction of x-axis.
View SolutionA vector of magnitude 13 makes an angle 65.37∘ with the x-axis . What is its component along positive y-axis ?
View SolutionA vector of magnitude 13 makes an angle 65.37∘ with the x-axis . What is its component along positive y-axis ?
View SolutionA vector →A makes an angle 30∘ with the Y-axis in anticlockwise direction. Another vector →B makes an angle 30∘ with the x-axis in clockwise direction. Find angle between vectors →A and →B.
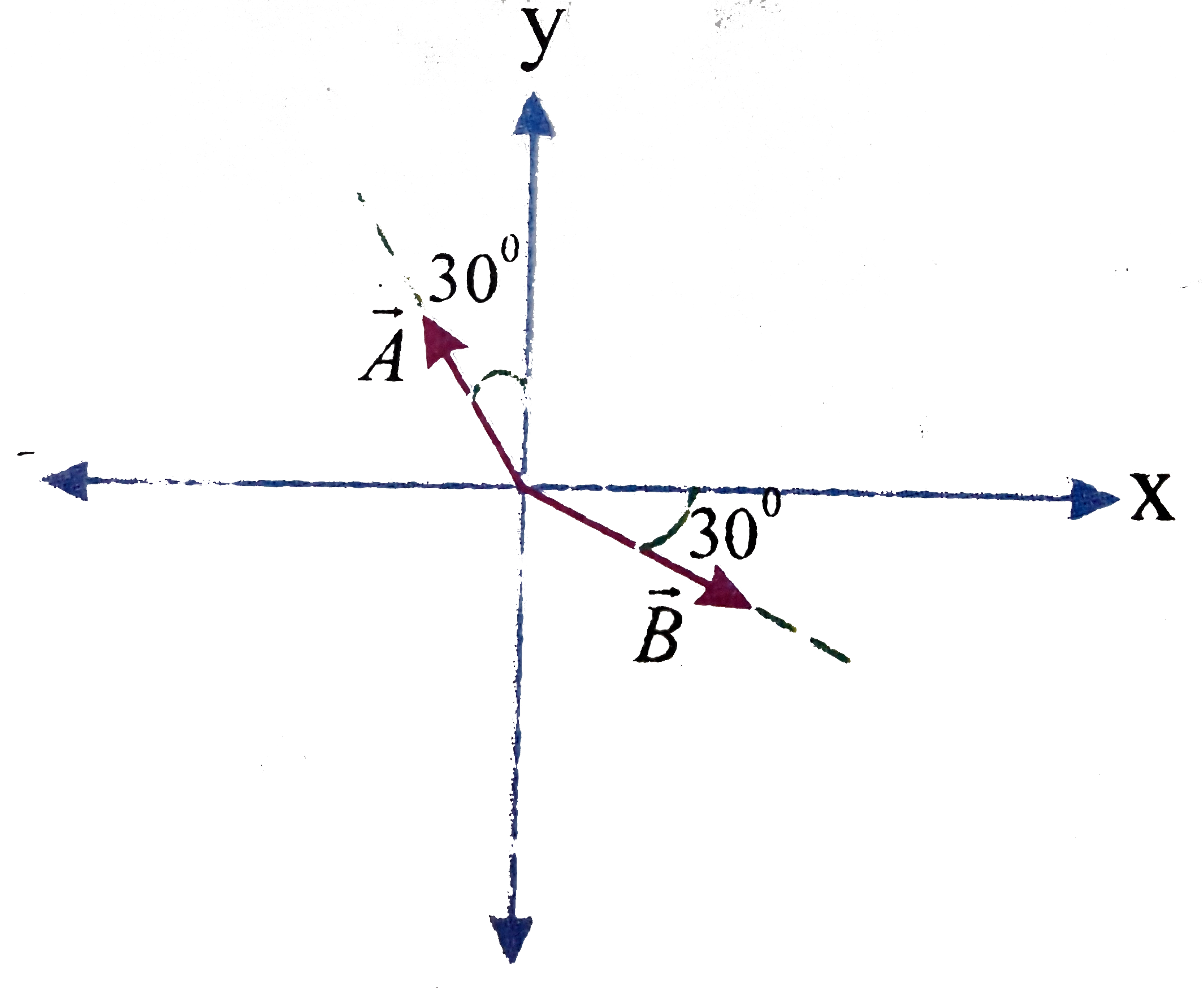
View SolutionWrite down a unit vector in XY plane, making an angle of 30∘ with positive direction of x-axis.
View Solution
