Three masses of 1 kg, 2 kg and 3 kg are placed at the vertices of an equilateral triangle of side 1m Take G=6.67×10−11Nm2kg−2, The gravitational potential energy of this system is −n×10−10J The integral value of n is nearly , Solution in Bengali
Step by step video & image solution for Three masses of 1 kg, 2 kg and 3 kg are placed at the vertices of an equilateral triangle of side 1m Take G = 6.67 xx 10^(-11)Nm^2 kg^-2, The gravitational potential energy of this system is -n xx 10^(-10) J The integral value of n is nearly , by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The Young's modulus of iron and steel are 5×1010N/m2 and 2×1011N/m2 respectively. An iron wire and steel wire of same length are extended by 2 mm under the same force. If the radii of iron and steel wires are RI and RS respectively, then what will be the relation between RI and RS?
View SolutionExplain why ?
The blood pressure in humans is greater at the feet than at the brain.
View SolutionThe value of gravitation is G=6.67×10−11N−m2kg2 in SL units . Convert it into CGS system of units .
View SolutionThree masses of 1kg , 2kg , and 3kg , are placed at the vertices of an equilateral traingle of side 1m . Find the gravitational potential energy of this system. Take G= 6.67xx10^(-11) N-m^(2)//kg^(2) .
View SolutionThe distance between the moon and earth is 3.8×108m. Find the gravitional potential at the mid point of the joining them. Given that the mass of the earth is 6×1024kg, mass of moon =7.4×1022kg and G=6.67×1011Nm2kg−2.
View SolutionThe distance between earth and moon is 3.8×108m. Determine the gravitational potential energy of earth-moon system. Given, mass of the earth =6×1024kg, mass of moon =7.4×1022kg and G=6.67×10−11Nm2kg−2
View SolutionCalculate the gravitational potential energy of a body of mass 30 kg , at a height of 4 R from the surface of Earth, where R (= 6.4 xx 10^(6) m) is the radius of the Earth. Mass of the Earth = 6.0 xx 10^(24) kg, G = 6.67 xx 10^(-11) Nm^(2) kg^(-2) .
View SolutionThree masses of 1 kg, 2kg, and 3 kg are placed at the vertices of an equilateral triangle of side 1m. Find the gravitational potential energy of this system (Take, G = 6.67 xx 10^(-11) "N-m"^(-2)kg^(-2) )
View SolutionThe mass of the earth is 6 xx 10^(24) kg . The constant of gravitation G = 6.67 xx 10^(-11) "N-m"^(-2) kg^(-2) . The potential energy of the earth and moon system is -7.79 xx 10^(28) J . Determine the mean distance between earth and moon.
View SolutionThe position vector of the objects of masses 25 kg and 10 kg are (4,7,5)m and (1,3,5) m respectively. Obtain the vector representing the gravitational force on 25 kg object by 10 kg object. (Take G=6.67×10−11Nm2kg−2 )
View SolutionThe mass of the earth is 6.00×1024 kg and that of the moon is 7.40×1022kg. The constant of gravitation G=6.67×10−11N−m2kg−2. The potential energy of the system is −7.79×1028J. The mean distance between the earth and moon is
View SolutionThe mass of the earth is 6.00×1024kg and that of the moon is 7.40×1022kg. The constant of gravitational G=6.67×10−11N−m2kg−2. The potential energy of the system is −7.79×1028J. The mean distance between the earth and moon is
View SolutionThe mass of the earth is 6.00 xx 10^(24) kg and that of the moon is 7.40 xx 10^(22) kg . The constant of gravitation G= 6.67xx 10^(-11) N-m^(2)kg^(-2) . The potential energy of the system is -7.79 xx 10^(28) J . The mean distance between the earth and moon is
View SolutionThe mass of the earth is 6.00×1024kg and that of the moon is 7.40×1022kg. The constant of gravitational G=6.67×10−11N−m2kg−2. The potential energy of the system is −7.79×1028J. The mean distance between the earth and moon is
View SolutionThe mass of the earth is 6.00×1024kg and that of the moon is 7.40×1022kg. The constant of gravitation G=6.67×10−11N−m2kg−2. The potential energy of the system is −7.79×1028J. The mean distance between the earth and moon is
View Solution1 किग्रा, 2 किग्रा तथा 3 किग्रा के तीन द्रव्यमान 1मी भुजा के समबाहु त्रिभुज के शीर्षों पर रखे हैं। निकाय की गुरुत्वीय स्थितिज ऊर्जा ज्ञात कीजिए।
(G=6.67×10−11 न्यूटन−मी2किग्रा−2)
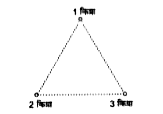
View Solution
