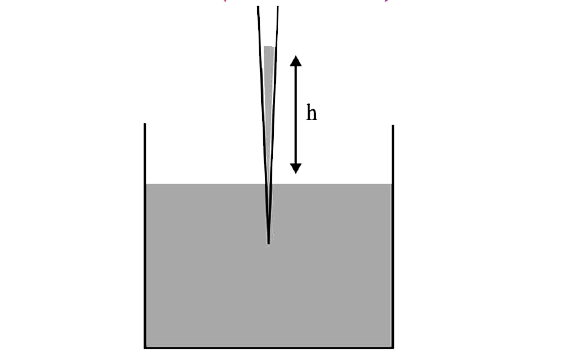
A glass capillary tube is of the shape of a truncated cone with an apex angle α so that its two ends have cross-sections of different radii. When dipped in water vertically, water rises in it to a height h, where radius of its cross- sections is b. If the surface tension of water is s, its density is ρ, and its angle of contact with glass is θ, the value of h will be (g is the acceleration due to gravity)
2Sbρgcos(θ−α)
2Sbρgcos(θ+α)
2Sbρgcos(θ−α2)
2Sbρgcos(θ+α2)
The correct Answer is:D
Topper's Solved these Questions
Similar Questions
A glass capillary tube is of the shape of a truncated cone with an apex angle α so that its two ends have cross sections of different radii. When dipped in water vertically, water rises in it to a high h, where the radius of its cross section is b. If the surface tension of water is S, its density if ρ, and its contact angle with glass is θ, the value of h will be (g is the acceleration due to gravity)
View SolutionIn a capillary tube , water rises to a height of 4 cm If its cross section area were one forth , the water would have to rises a height of
View Solution
Knowledge Check
A glass capillary tube is of the shape of a truncated cone with an apex angle α so that its two ends have cross sections of different radii. When dipped in water vertically, water rises in it to a high h, where the radius of its cross section is b. If the surface tension of water is S, its density if ρ, and its contact angle with glass is θ, the value of h will be (g is the acceleration due to gravity)
 A(a) 2Sbρgcos(θ−α)B(b) 2Sbρgcos(θ+α)C(c) 2Sbρgcos(θ−α/2)D(d) 2Sbρgcos(θ+a/2)
A(a) 2Sbρgcos(θ−α)B(b) 2Sbρgcos(θ+α)C(c) 2Sbρgcos(θ−α/2)D(d) 2Sbρgcos(θ+a/2)A glass capillary tube is of the shape of a truncated cone with an apex angle α so that its two ends have cross sections of different radii. When dipped in water vertically, water rises in it to a high h, where the radius of its cross section is b. If the surface tension of water is S, its density if ρ, and its contact angle with glass is θ, the value of h will be (g is the acceleration due to gravity)
 A2Sbρgcos(θ−α)B2Sbρgcos(θ+α)C2Sbρgcos(θ−α/2)D2Sbρgcos(θ+α)
A2Sbρgcos(θ−α)B2Sbρgcos(θ+α)C2Sbρgcos(θ−α/2)D2Sbρgcos(θ+α)When liquid medicine ρ is to be put in the eye, it is done with the help of a dropper. As the bulb on the top of the dropper is pressed, a drop froms at the opening of the dropper. We wish to estimate the size of the drop. We first assume that the drop formed at the opening is osperical becaus ethat requires a minimum increase in its surface energy. To datermine the size., we calculate the net vertical force due to the surface tension T when the radius of the drop is R. When this force become smaller than the wight of the drop, the drop gets detached from the droppr.
A glass capillary tube is of the shape of a truncated cone with an apex angle α so that its two ends have cross sections of different raddi. When dipped in water verically, water rises in it to a height h, where the radius of its cross section is b. If the surface tension of water is S, its density is ρ, and its contact angle with glass is θ, the value of h will be (g is the acceleration due to gravity)
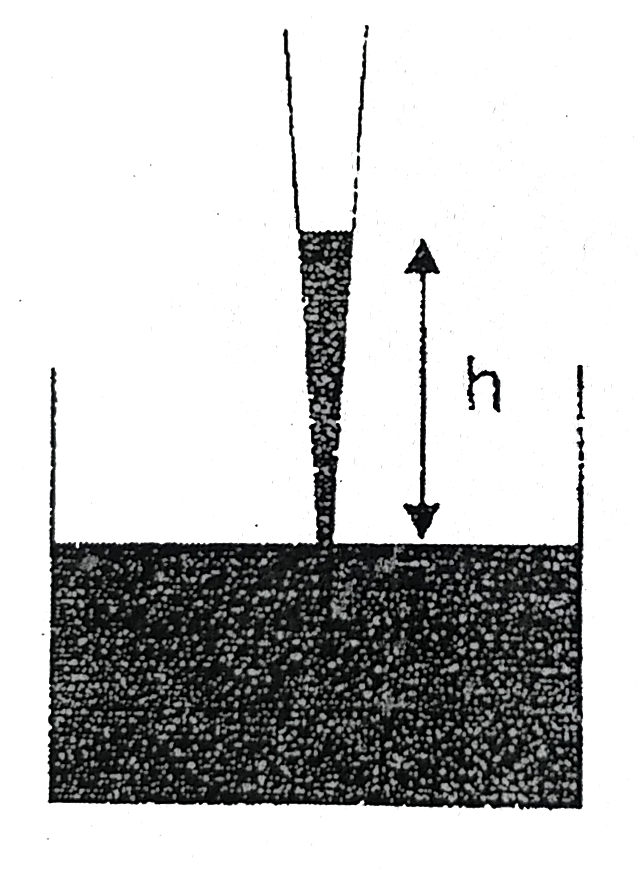 A2Sbρgcos(θ−α)B2Sbρgcos(θ+α)C2Sbρgcos(θ−α/2)D2Sbρgcos(θ+α/2)
A2Sbρgcos(θ−α)B2Sbρgcos(θ+α)C2Sbρgcos(θ−α/2)D2Sbρgcos(θ+α/2)
Water rises to a height h in a capillary tube of cross-sectional area A. the height to which water will rise in a capillary tube of cross-sectional area 4A will be
View SolutionWater rises to a height h in a capillary tube of cross-sectional area A. the height to which water will rise in a capillary tube of cross-sectional area 4A will be
View SolutionThe surface tension of water is 7×10−2N/m2 and its angle of contact with glass is zero . The rise of water in a capillary tube of diameter 0.5 mm is ………..
View SolutionIn a capillary tube , water rises to a height of 4 cm If its cross section area were one forth , the water would have to rises a height of
View SolutionWater rises to a height h in a capillary tube of cross-sectional area A. the height to which water will rise in a capillary tube of cross-sectional area 4A will be
View Solution
