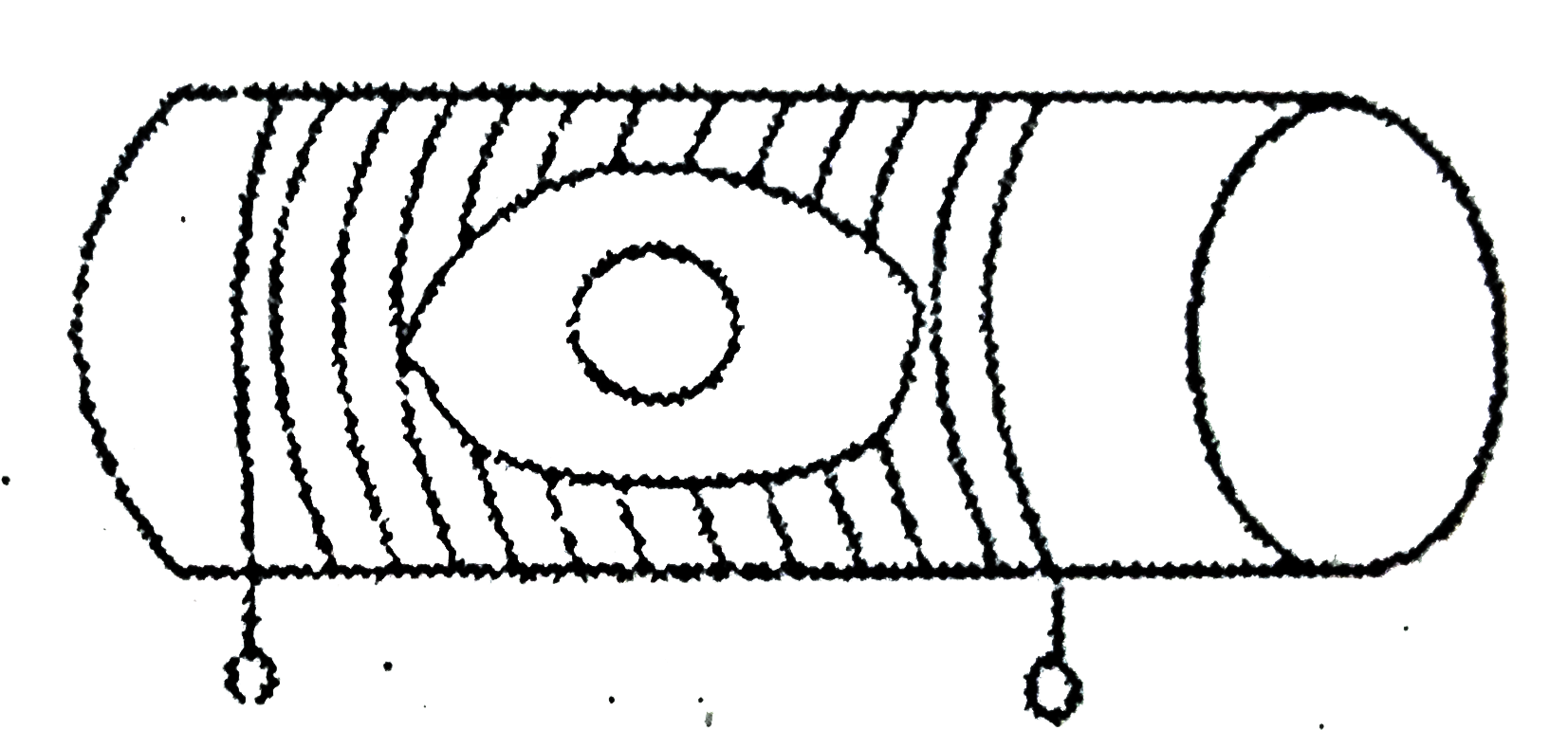
A long solenoid of length 50 cm and cross sectional area 10cm2 is closely wound with 1000 turns of wire. A small coil of 10 turns surrounds it at its centre. Calculate the mutual inductance of the two coils. Solution in Bengali
L =50cm=0.50m.A=10×10−4m2, N2=10 turns, N1=1000turns.
M=μ0N1N2Al=4π×10−7×10×1000×10×10−40.50=0.025mH
Topper's Solved these Questions
Similar Questions
Can 8.6×10−19 Coulomb of charge be given to a conductor. Explain.
03:15View SolutionCan two equipotential surfaces intersect?
03:47View SolutionA 2 m long solenoid with diameter 4 cm and 2000 turns has a secondary of 1000 turns wound closely near its mid point. Calculate the mutual inductance between the two coils.
04:22View SolutionA solenoid is of length 50cm and has a radius of 2cm. It has 500 turns. Around its central section a coil of 50 turns is wound. Calculate the mutual inductance of the system.
01:44View SolutionA long solcnoid of length 1m, cross-sectional area 10cm2, having 1000 turns has wound about its centre a small coil of 20 turns. Compute the mutual inductance of the pair of solenoid and the coil . What is the induced EMF in the coil when the current in the solenoid changes at the rate of 10A/s?
04:19View SolutionThe self inductance of a solenoid that has a cross-sectional area of 1 cm^(2) , a length of 10 cm and 1000 turns of wire is
03:05View SolutionA circular coil with a cross-sectional area of 4cm^(2) has 10 turns. It is placed at the center of a long solenoid that has 15 turns/cm and a cross sectional area of 10cm^(2) , shown in the figure. The axis of the coil conicides with the axis of the solenoid. What is their mutual inductance?
04:30View SolutionA solenoidal coil has 50 turns per centimeter along its length and a cross sectional area of 4×10−4m2. 200 turns of another wire is wound round the first solenoid co-axially. The two coils are electrically insulated from each other. Calculate the mutual inductance between the two coils.
04:30View SolutionA circular coil with a cross-sectional area of 4cm2 has 10 turns/cm. It is placed at the centre of a long solenoid that has 15 turns/cm and a cross-sectional area of 10cm2. The axis of the coil coincides with the axis of the solenoid. What is their mutual inductance ?
02:29View SolutionA circular coil with a cross-sectional area of 4cm2 has 10 turns/cm. It is placed at the centre of a long solenoid that has 15 turns/cm and a cross-sectional area of 10cm2. The axis of the coil coincides with the axis of the solenoid. What is their mutual inductance ?
02:07View SolutionA solenoid is of length 50 cm and has a radius of 2cm. It has 500 turns. Around its central section a coil of 50 turns is wound. Calculate the mutual inductance of the system.
View SolutionA solenoidal coil has 50 turns per centimetre along its length and a cross-sectional area of 4xx10^(-4)m^(2) . 200 turns of another wire is wound round the first solenoid co-axially. The two coils are electrically insulated from each other. Calculate the mutual inductance between the two coils.
02:52View SolutionA solenoid is of length 50 cm and has a radius of 2cm. It has 500 turns. Around its central section a coil of 50 turns is wound. Calculate the mutual inductance of the system.
03:42View SolutionThe self inductance of a solenoid that has a cross-sectional area of 1cm2, a length of 10 cm and 1000 turns of wire is
04:50View SolutionA circular coil with a cross-sectional area of 4cm2 has 10 turns. It is placed at the center of a long solenoid that has 15 turns/cm and a cross sectional area of 10cm2, shown in the figure. The axis of the coil conicides with the axis of the solenoid. What is their mutual inductance?
 02:48View Solution
02:48View Solution

