A particle starts moving from rest from a fixed point in a fixed direction. The distance s from the fixed point at a time t is given by s=t2+at−b+17, where a, b are real numbers. If the particle comes to rest after 5 sec at a distance of s = 25 units from the fixed point, then values of a and b are respeetivelySolution in Bengali
10,−33
−10,−33
−8,33
−10,33
The correct Answer is:B
Step by step video, text & image solution for A particle starts moving from rest from a fixed point in a fixed direction. The distance s from the fixed point at a time t is given by s = t^2+at-b + 17, where a, b are real numbers. If the particle comes to rest after 5 sec at a distance of s = 25 units from the fixed point, then values of a and b are respeetively by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The distance S metres moved by a particle traveling in a straight line in t second is given by S = 45t+11t2−t3. Find the time when the particle comes to rest.
View SolutionThe speed-time graph of a particle moving along a fixed direction as shown in the figure. The distance transversed by the particle between t = 0 s to t = 10 s is
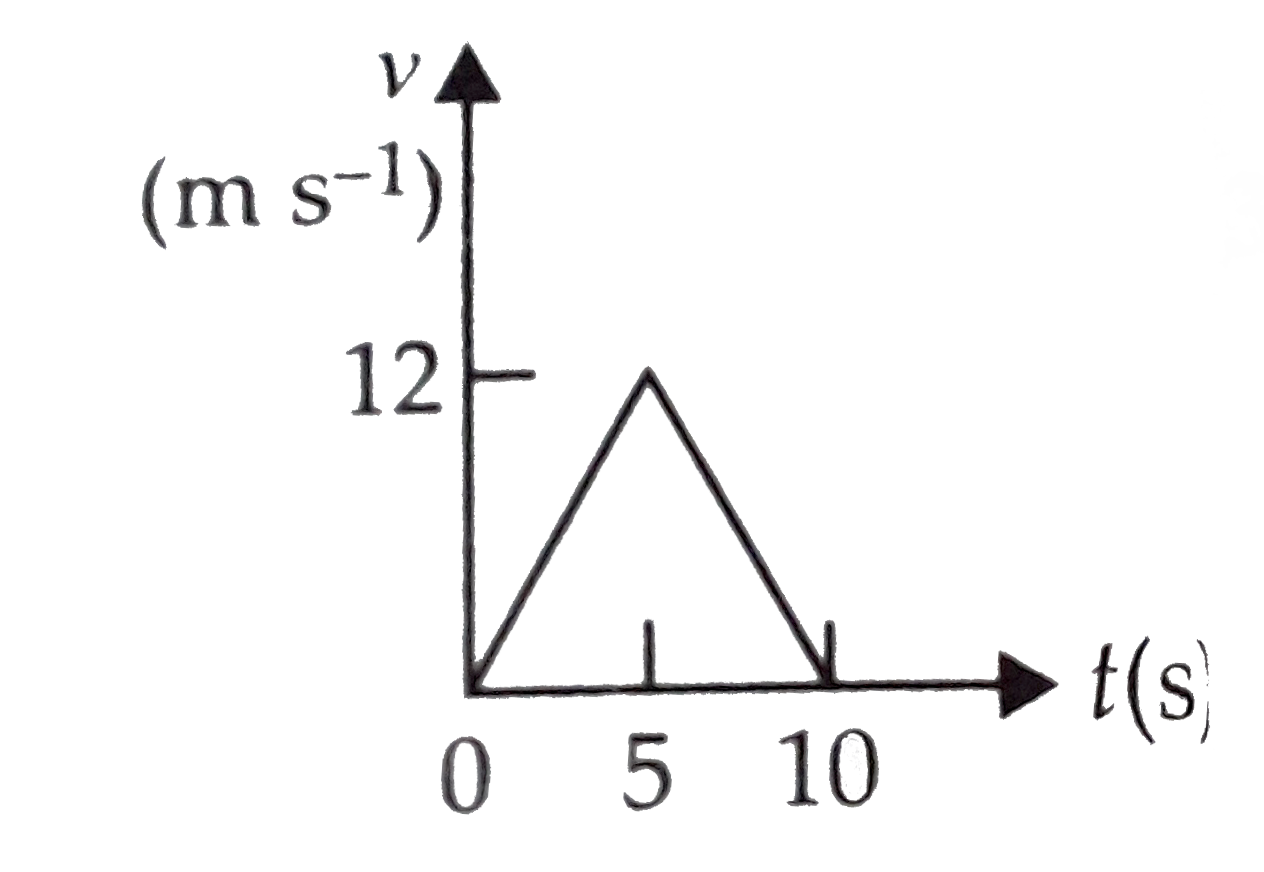
View SolutionA particle moves along a straight line according to the law s=16−2t+3t3, where s metres is the distance of the particle from a fixed point at the end of t second. The acceleration of the particle at the end of 2s is
View SolutionThe distance form a fixed point O of a particle pmoving in a straight line from O is given by s=16+48t−t3. The direction of motion of the particle after t=4 sec is
View SolutionA particle starts moving from rest from a fixed point in a fixed direction. The distance s from the fixed point at a time t is given by s = t2+at−b+17, where a, b are real numbers. It the particle comes to rest after 5 sec at a distance of s = 25 units from the fixed point, then values of a and b are respectively
View SolutionThe distance covered by a particle moving in a straight line from a fixed point on the line is s, where s2=at2+2bt+⋅ Then prove that acceleration is proportional to s−3.
View SolutionThe distance covered by a particle moving in a straight line from a fixed point on the line is s, where s2=at2+2bt+c. Then prove that acceleration is proportional to s−3.
View SolutionA particle starts moving from a certain point in a certain direction. Distance from fixed point at time t s=t^(2)+at-b+17 Is given by where a, b are real numbers. If the particle comes to rest at s = 25 unit distance from the fixed point after 5 seconds, then the values of a and b are respectively
View Solutionएक कण एक निश्चित बिंदु से एक निश्चित दिशा में विराम से चलना प्रारम्भ करता है। निश्चित बिंदु से समय t पर दूरी s=t2+at−b+17 से दी जाती है जहाँ a,b वास्तविक संख्याएँ है। यदि कण 5 सेकण्ड बाद निश्चित बिंदु से s=25 इकाई दूरी पर विराम में आता है , तो क्रमशः a औऱ b के मान है
View SolutionThe speed-time graph of a particle moving along a fixed direction as shown in the figure. The distance traversed by the particle between t = 0 s to t = 10 s is
View SolutionA particle starts moving from rest from a fixed point in a fixed direction. The distances from the fixed point at a time t is given by s=t2+at−b+17, where a, b are real numbers. If the particle comes to rest after 5 sec at a distance of s = 25 units from the fixed point, then values of a and bare respectively
View Solutionএকটি কণা একটি নির্দিষ্ট বিন্দুতে স্থিতাবস্থা থেকে একটি নির্দিষ্ট দিকে যাত্রা শুরু করে | কণাটির t সময়ে ওই নির্দিষ্ট বিন্দু থেকে দূরত্ব s এবং s=t2+at−b+17 , a,b বাস্তব সংখ্যা | কণাটি যদি আবার স্থিতাবস্থায় আসে 5 sec পরে s=25 একক দূরত্বে (প্রারম্ভিক বিন্দু থেকে) তবে a এবং b-র মান হল যথাক্রমে হল
View SolutionThe distance covered by a particle moving in a straight line from a fixed point on the line is s, where s2=at2+2bt+c. Then prove that acceleration is proportional to s−3.
View SolutionA particle moves from rest away from a fixed point O, k with an acceleration a = kx2 where k = constant and x x is the distance from O. The particle is at rest when at a distance S from O. What is its velocity when at a distance of 2S from O ?
View SolutionA particle moves along a straight line according to the law s=16−2t+3t3 where s metres is the distance of the particle from a fixed point at the end of t sec the acceleration of the particle at the end of 2s is
View Solution