A circular wire of radius r has a uniform linear charge density λ=λ0cos2θ, The total charge on the wire is απλ0r2 Find α.
The correct Answer is:1
To solve the problem, we need to find the value of α given that the total charge Q on a circular wire of radius r with a linear charge density λ=λ0cos2θ is expressed as Q=απλ0r2.
1. Understanding the Charge Density:
The linear charge density is given as:
λ=λ0cos2θ
This means that the charge density varies with the angle θ around the circular wire.
2. Calculating the Differential Charge:
The differential charge dQ on an infinitesimal segment of the wire can be expressed as:
dQ=λ⋅ds
where ds is the infinitesimal arc length. For a circular wire, ds=rdθ. Thus,
dQ=λ0cos2θ⋅rdθ
3. Integrating to Find Total Charge:
To find the total charge Q, we integrate dQ from θ=0 to θ=2π:
Q=∫2π0dQ=∫2π0λ0cos2θ⋅rdθ
This simplifies to:
Q=λ0r∫2π0cos2θdθ
4. Evaluating the Integral:
We can use the identity for cos2θ:
cos2θ=1+cos2θ2
Therefore, the integral becomes:
∫2π0cos2θdθ=∫2π01+cos2θ2dθ=12(∫2π01dθ+∫2π0cos2θdθ)
The integral of cos2θ over one complete period is zero, so:
∫2π0cos2θdθ=12⋅2π=π
5. Substituting Back to Find Total Charge:
Now substituting back into the expression for Q:
Q=λ0r⋅π=πλ0r
6. Relating to Given Expression:
We are given that:
Q=απλ0r2
Setting the two expressions for Q equal gives:
πλ0r=απλ0r2
7. Solving for α:
Dividing both sides by πλ0 (assuming λ0≠0):
1r=αr
Thus,
α=1r2
Final Result:
Since we need to express α in terms of r and we are looking for a constant, we find:
α=1
The linear charge density is given as:
λ=λ0cos2θ
This means that the charge density varies with the angle θ around the circular wire.
The differential charge dQ on an infinitesimal segment of the wire can be expressed as:
dQ=λ⋅ds
where ds is the infinitesimal arc length. For a circular wire, ds=rdθ. Thus,
dQ=λ0cos2θ⋅rdθ
To find the total charge Q, we integrate dQ from θ=0 to θ=2π:
Q=∫2π0dQ=∫2π0λ0cos2θ⋅rdθ
This simplifies to:
Q=λ0r∫2π0cos2θdθ
We can use the identity for cos2θ:
cos2θ=1+cos2θ2
Therefore, the integral becomes:
∫2π0cos2θdθ=∫2π01+cos2θ2dθ=12(∫2π01dθ+∫2π0cos2θdθ)
The integral of cos2θ over one complete period is zero, so:
∫2π0cos2θdθ=12⋅2π=π
Now substituting back into the expression for Q:
Q=λ0r⋅π=πλ0r
We are given that:
Q=απλ0r2
Setting the two expressions for Q equal gives:
πλ0r=απλ0r2
Dividing both sides by πλ0 (assuming λ0≠0):
1r=αr
Thus,
α=1r2
Since we need to express α in terms of r and we are looking for a constant, we find:
α=1
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The dimensional formula of linear charge density λ is
A[M−1L−1T+1A]B[M0L−1T+1A]C[M−1L−1T+1A−1]D[M0L−1T+1A−1] - Question 2 - Select One
A half ring of radius r has a linear charge density λ.The potential at the centre of the half ring is
Aλ4εoln0Bλ4π2ε0rCλ4πε0rDλ4πε0r2 - Question 3 - Select One
A thin non-conducting ring of radius R has a linear charge density λ=λ0cosθ, where θ is measured as shown. The total electric dipole moment of the charge distribution is
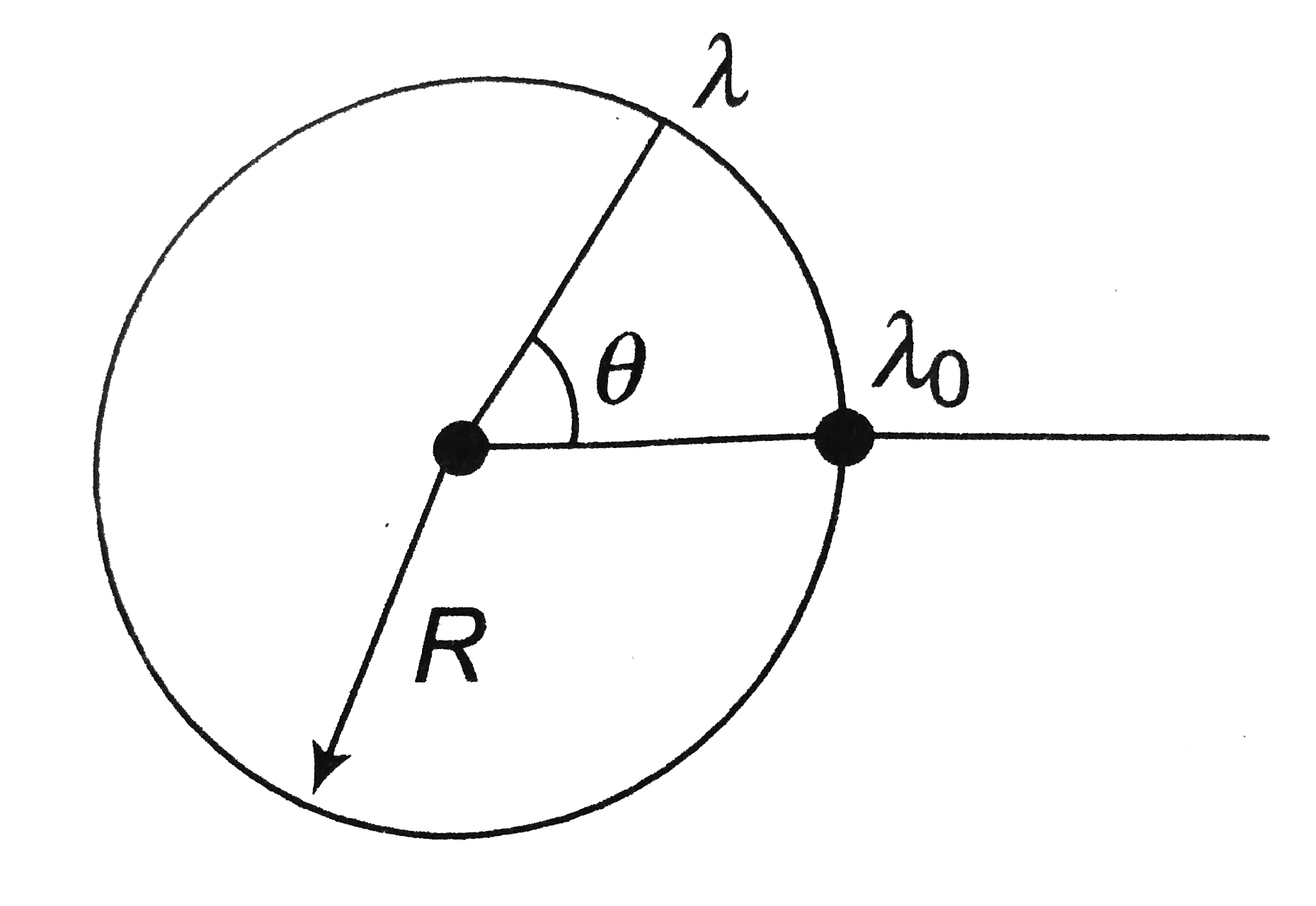 AR2λ0B2πR2λ0CπR2λ02DπR2λ0
AR2λ0B2πR2λ0CπR2λ02DπR2λ0
A thin nonconducting ring of radius R has a linear charge density λ=λ0cosφ, where λ0 is a constant , ϕ is the azimutahl angle. Find the magnitude of the electric field strength
(a) at the centre of the ring ,
(b) on the axis of the ring as a function of the distance x from its centre. Investegation the obtained function at x>>R.View SolutionCharge on the ring is given as λ=λ0cosθ C/m .Find dipole moment of the ring
View SolutionA non conducting ring of radius R1 is charged such that the linear charge density is λ1cos2θ where θ is the polar angle. If the radius is increased to R2 keeping the charge constant, the linear charge density is changed to λ2cos2θ. The relation connecting R1, R2λ1 and λ2 will be
View SolutionA circle of radius a has charge density given by λ=λ0cos2θ on its circumference, where λ0 is a positive constant and θ is the angular position of a point on the circle with respect to some reference line. The potential at the centre of the circle is
View Solutionthe following diagram represents a semi circular wire of linear charge density λ=λ0sinθ where λ0is a positive constant.The electric potential at o is (take k=14πε0
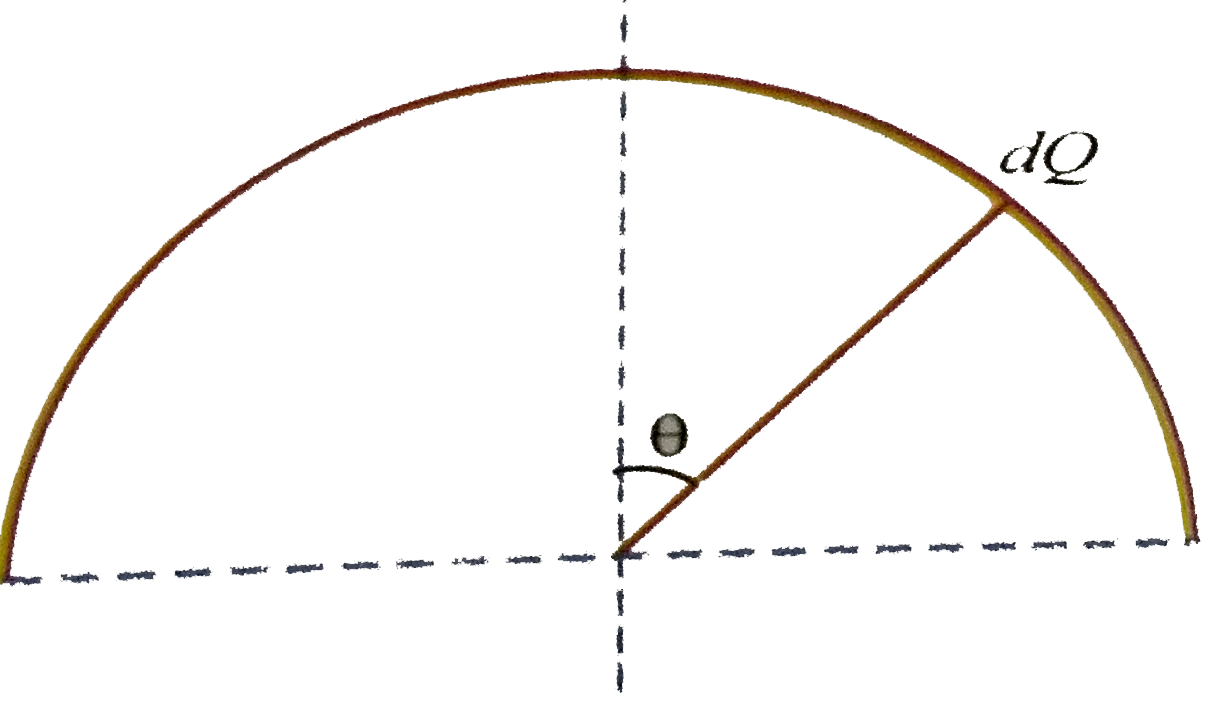 View Solution
View Solution

