Question

Obtain the formulae for displacement, velocity, acceleration and time period of a particle executing simple harmonic motion. When is the velocity of particle maximum and when zero ? Acceleration ?

Explanation

Let us consider a particle executing a uniform circular motion with uniform speed
. O is the center of the circle.
OP = a = radius of the circle.
XX. and YY are the two perpendicular axes along diameters.
The projection of the uniform circular motion on a diameter of a circle executes a simple harmonic motion.

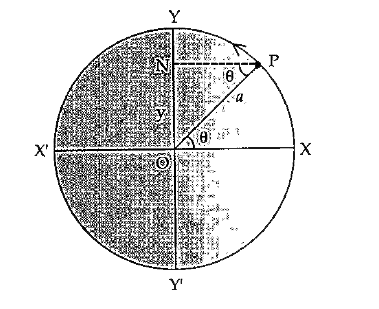
The distance travelled by the foot of the perpendicular (N) drawn from the revolving particle (P) at any instant of time t from its mean position (O) is known as displacement. When the particle is at P, the displacement of the foot of the perpendicular (N) from O along Yaxis is y.
`ON = y = OP sin theta`
`Or, y = a sin omega t (because theta = omea t and OP = a = ` tan radius of the circle, it is the amplitude of the motion)
`therefore y =a sin ometa t ...(i)`
The rate of change of displacemtn is the velocity.
Differentiating eqn. (i) with respect time t
`(dy)/(dt) = (d)/(dt) (a sin omega t)`
`or, v = a omega cos theta t ...(ii)`
or, `v = a omega (sqrt) (1 - sin ^(2) omega f)`
or, `v = a omega (sqrt) [1- (y ^(2) //a ^(2)) ]`
`omega v = a omega (sqrt) (a ^(2) - t ^(2))...(iii)`
The rate of change of velocity is the acceleration.
`(d ^(2) y )/(dt ^(2)) = (d)/(dt) (a cos omega t )`
or,` alpha =- omega ^(2) a sin omega t .....(iv)`
`therefore alpha = - omega ^(2) y ...(v)`
(-ve sign indicates that the acceleration is the opposite direction of the displacement)
Formula of time period:
The period of the projection `= T = 2pi // omega = ...(vi)`
From equation (v) considering the magnitude of acceleration `(alpha)`
`alpha = omega ^(2) y`
`therefore omega ^(2) = alpha //y`
Putting in equation (vi)
`T = pi (sqrt) (y //alpha ) ....(vii)`
Maximum velocity and zero velocity : The velocity is , when y = a i.e. when the projection passes through the end points of the motion where displacement is maximum. The velocity is maximum, when the projection passes through the mean position (y = 0) of the motion,
Maximum acceleration and zero acceleration : The acceleration is 0, when y = 0, i.e. the projection passes through the mean position. It is maximum, when the particle passes through the end points of the motion. The acceleration is maximum when the projection passes through the end points of the motion.