How is force related to the momentum of a body?
To understand how force is related to the momentum of a body, we can break down the explanation into several steps:
Step 1: Understand the Definitions
- Momentum (P): Momentum of a body is defined as the product of its mass (m) and its velocity (v). Mathematically, this is expressed as:
P=m⋅v
Step 2: Newton's Second Law of Motion
- According to Newton's second law of motion, the force (F) acting on an object is equal to the rate of change of its momentum. This can be mathematically expressed as:
F=dPdt
where dPdt is the derivative of momentum with respect to time, indicating how momentum changes over time.
Step 3: Relate Force to Mass and Acceleration
- Force can also be expressed in terms of mass and acceleration (a) using the formula:
F=m⋅a
where acceleration is defined as the change in velocity over time.
Step 4: Connecting Momentum and Force
- Since acceleration (a) is the change in velocity (v) over time (t), we can rewrite it as:
a=dvdt
- Therefore, we can express force in terms of momentum:
F=m⋅dvdt
- If we substitute P=m⋅v into this equation, we can see that:
F=d(m⋅v)dt
- This shows that force is indeed related to the rate of change of momentum.
Step 5: Conclusion
- From the above derivations, we conclude that:
F=dPdt
This means that force is equal to the rate of change of momentum of a body.
Summary
- The relationship between force and momentum can be summarized as follows: Force is directly proportional to the rate of change of momentum. This can be expressed mathematically as:
F=Pt
where P is momentum and t is time.
- Momentum (P): Momentum of a body is defined as the product of its mass (m) and its velocity (v). Mathematically, this is expressed as:
P=m⋅v
- According to Newton's second law of motion, the force (F) acting on an object is equal to the rate of change of its momentum. This can be mathematically expressed as:
F=dPdt
where dPdt is the derivative of momentum with respect to time, indicating how momentum changes over time.
- Force can also be expressed in terms of mass and acceleration (a) using the formula:
F=m⋅a
where acceleration is defined as the change in velocity over time.
- Since acceleration (a) is the change in velocity (v) over time (t), we can rewrite it as:
a=dvdt
- Therefore, we can express force in terms of momentum:
F=m⋅dvdt
- If we substitute P=m⋅v into this equation, we can see that:
F=d(m⋅v)dt
- This shows that force is indeed related to the rate of change of momentum.
- From the above derivations, we conclude that:
F=dPdt
This means that force is equal to the rate of change of momentum of a body.
- The relationship between force and momentum can be summarized as follows: Force is directly proportional to the rate of change of momentum. This can be expressed mathematically as:
F=Pt
where P is momentum and t is time.
Topper's Solved these Questions
- SELF ASSESSMENT PAPER -3Book:ICSEChapter:SELF ASSESSMENT PAPER -3Exercise:SECTION-A
Explore 31 Videos
- SELF ASSESSMENT PAPER -2Book:ICSEChapter:SELF ASSESSMENT PAPER -2Exercise:SECTION .B.
Explore 37 Videos
- SELF ASSESSMENT PAPER -5Book:ICSEChapter:SELF ASSESSMENT PAPER -5Exercise:SECTION-B
Explore 35 Videos
Similar Questions
Knowledge Check
A force of 50 N acts on a body for 10 s. What will be the change in momentum of the body ?
A200 N.sB400 N.sC500 N.sD1000 N.s
(A) : A rocket works on the principle of conservation of linear momentum.
(r) : When there is no external force on a system of two bodies the rate of change in momenta of two bodies are equal and opposite
View SolutionAssertion: If net force on a system is zero, then momentum of every individual body remains constant.
Reason: If momentum of a system is constant, then kinetic energy of the system may change.
View SolutionAssertion : The force of tension on a body always act away from the body.
Reason: Friction force always opposes the motion of a body.
View SolutionThe kinetic energy of a body is increased by 21%. What is the percentage increase in the magnitude of linear momentum of the body?
View SolutionA force of 10 N acts on a body of mass 2 kg for 3s , initially at rest. Calculate change in momentum of the body.
View SolutionStatement A : If the force varies with time in a complicated way then the average force is measured by the total change in momentum of the body
Statement B : Change in momentum and impulsive force are numerically equal
View SolutionA force lime graph for the motion of a body is as shown in figure. Change in linear momentum between 0 and 6s is:
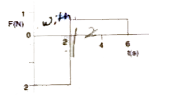
View Solution