For , which one of the following values of l is not possible ?
1
2
3
4
The correct Answer is:4
To determine which value of the azimuthal quantum number l is not possible for a principal quantum number n=4, we will follow these steps:
Step 1: Understand the relationship between n and l
The azimuthal quantum number l can take on values from 0 to n−1. This means that for a given principal quantum number n, the possible values of l are:
l=0,1,2,…,(n−1)
Step 2: Calculate the maximum value of l for n=4
Given n=4:
l can take values from 0 to n−1=4−1=3
Thus, the possible values of l are:
l=0,1,2,3
Step 3: Identify the options provided
We need to check the options provided in the question to see which one exceeds the maximum value of l calculated in Step 2. The options are:
1. l=1
2. l=2
3. l=3
4. l=4
Step 4: Determine which value of l is not possible
From the possible values of l (0, 1, 2, 3), we can see that:
- l=1 is possible
- l=2 is possible
- l=3 is possible
- l=4 is not possible because it exceeds the maximum value of 3.
Conclusion
The value of l that is not possible for n=4 is:
Option 4: l=4
The azimuthal quantum number l can take on values from 0 to n−1. This means that for a given principal quantum number n, the possible values of l are:
l=0,1,2,…,(n−1)
Given n=4:
l can take values from 0 to n−1=4−1=3
Thus, the possible values of l are:
l=0,1,2,3
We need to check the options provided in the question to see which one exceeds the maximum value of l calculated in Step 2. The options are:
1. l=1
2. l=2
3. l=3
4. l=4
From the possible values of l (0, 1, 2, 3), we can see that:
- l=1 is possible
- l=2 is possible
- l=3 is possible
- l=4 is not possible because it exceeds the maximum value of 3.
The value of l that is not possible for n=4 is:
Option 4: l=4
Topper's Solved these Questions
- STRUCTURE OF ATOM BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRUCTURE OF ATOM EXERCISE - ASSIGNMENT ( SECTION -B) Objective Type Questions (One option is correct)
38 Videos
- STRUCTURE OF ATOM BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRUCTURE OF ATOM EXERCISE - ASSIGNMENT ( SECTION -C) Objective Type Questions (More than one options are correct)
15 Videos
- STRUCTURE OF ATOM BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRUCTURE OF ATOM EXERCISE - Try Yourself
40 Videos
- STRUCTURE OF ATOMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRUCTURE OF ATOMEXERCISE - ASSIGNMENT (SECTION -D) Assertion-Reason Type Questions
15 Videos
- SURFACE CHEMISTRY BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SURFACE CHEMISTRY EXERCISE - Assignment Section - C (Assertion - Reason type questions)
10 Videos
Similar Questions
Knowledge Check
Which one of the following is not possible in a cyclic process?
AWork done by the system is positiveBHeat added to the system is positiveCWok done on the system is positiveDHeat removed from the system is negativeWhich one of the following is a possible nuclear reaction ?
A.510B+.42He→.137N+.11HB.2311Na+.11H→.2010Ne+.42HeC.23993Np→.23994Pu+.−1β0+¯vD.117N+.11H→.126C+.−1β0+¯vThe table below shows the numbers of rows and columns in each of 5 matrices.
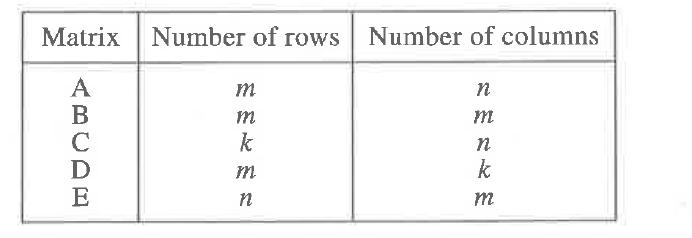
For distinct values of k, m, and n, which of the following matrix products is NOT possible?AEDBDCCCEDAC
A stretched string of length L , fixed at both ends can sustain stationary waves of wavelength λ Which of the following value of wavelength is not possible ?
View SolutionWhen the value of (n + l) is not more than 3 which of the following subshell is not possible to exist?
View SolutionConsider the following sets of quantum numbers.
(i) nlms300+1/2
(ii) nlms221+1/2
(iii) nlms43−2−1/2
(iv) nlms10−1−1/2
(v) nlms323+1/2
Which of the following sets of quantum number is not possible ?
View SolutionExplain giving reasons, which of the following sets of quantum numbers are not possible?
(a) n=0, l=0, ml=0,ms=+12
(b) n=1,l=0,ml=0,ms=−12
(c) n=1,l=1,ml=0,ms=+12
(d) n=2,l=1,ml=0,ms=−12
(e) n=3,l=3,ml=−3,ms=+12
(f) n=3,l=1,ml=0,ms=+12
View SolutionWhich one of the following sets of quantum number is possible for electron in a 4f- orbital?
View Solution
