Calculate the work done when 1 mole of a gas expands reversibly and isothermally from 5 atm to 1 atm at 300 K. [Value of log 5 = 0.6989].
−4015 J
+4015 J
Zero
−15 J
The correct Answer is:A
To calculate the work done when 1 mole of a gas expands reversibly and isothermally from 5 atm to 1 atm at 300 K, we can use the formula for work done in an isothermal reversible process:
1. Identify the Given Data:
- Initial Pressure, P1=5atm
- Final Pressure, P2=1atm
- Temperature, T=300K
- Number of moles, n=1mol
- Universal gas constant, R=8.314J/mol⋅K
- Given value of log5=0.6989
2. Use the Formula for Work Done:
The formula for work done during isothermal expansion is:
W=−2.303nRTlog(P1P2)
3. Substitute the Values into the Formula:
W=−2.303×1mol×8.314J/mol⋅K×300K×log(51)
W=−2.303×1×8.314×300×log(5)
4. Calculate log(5):
We know that log(5)=0.6989.
5. Perform the Calculation:
W=−2.303×1×8.314×300×0.6989
W=−2.303×2494.2×0.6989
W=−2.303×1742.4
W≈−401.7J
6. Round the Result:
The work done W≈−415J (considering significant figures and rounding).
Final Answer:
The work done when 1 mole of gas expands isothermally and reversibly from 5 atm to 1 atm at 300 K is approximately:
W≈−415J
- Initial Pressure, P1=5atm
- Final Pressure, P2=1atm
- Temperature, T=300K
- Number of moles, n=1mol
- Universal gas constant, R=8.314J/mol⋅K
- Given value of log5=0.6989
The formula for work done during isothermal expansion is:
W=−2.303nRTlog(P1P2)
W=−2.303×1mol×8.314J/mol⋅K×300K×log(51)
W=−2.303×1×8.314×300×log(5)
We know that log(5)=0.6989.
W=−2.303×1×8.314×300×0.6989
W=−2.303×2494.2×0.6989
W=−2.303×1742.4
W≈−401.7J
The work done W≈−415J (considering significant figures and rounding).
The work done when 1 mole of gas expands isothermally and reversibly from 5 atm to 1 atm at 300 K is approximately:
W≈−415J
Topper's Solved these Questions
- THERMODYNAMICSBook:AAKASH INSTITUTE ENGLISHChapter:THERMODYNAMICSExercise:SECTION-B
Explore 20 Videos
- THERMODYNAMICSBook:AAKASH INSTITUTE ENGLISHChapter:THERMODYNAMICSExercise:SECTION-C
Explore 13 Videos
- THERMODYNAMICSBook:AAKASH INSTITUTE ENGLISHChapter:THERMODYNAMICSExercise:TRY YOURSELF
Explore 66 Videos
- THE SOLID STATE Book:AAKASH INSTITUTE ENGLISHChapter:THE SOLID STATE Exercise:Assignment (SECTION - D) (ASSERTION-REASON TYPE QUESTION)
Explore 20 Videos
Similar Questions
Calculate entropy change when 10mol of an ideal gas expands reversible and isothermally from an initial volume of 10L to 100L at 300K.
View SolutionThe molar entropy content of 1 mole of oxygen (O2) gas at 300K and 1atm is 250Jmole−1K−1. Calculate ΔG when 1 mole of oxygen is expanded reversibility and isothermally from 300K, 1 atm to double its volume ( Take R=8.314Jmole−1K−1,loge=2.303)
View Solution
Knowledge Check
What is the work done when 1 mole of a gas expands isothermally from 25 L to 250 L at a constant pressure of 1 atm and a temperature of 300 K ?
A(a) −3542JB(b) −5744JC(c) −2657JD(d) −4890JWhat will be the work done when one mole of a gas expands isothermally from 15 L to 50 L against a constant pressure of 1 atm at 25∘C?
A−3542calB−843.3calC−718calD−60.23cal
Calculate the work done when 1 mol of an ideal gas is compressed reversibly from 1 bar to 4 bar at a constant temperature of 300 K
View SolutionCalculate the work done by 1.0mol of an ideal gas when it expands at external pressure 2atm from 10atm to 2atm at 27∘C
View SolutionWork done during isothermal expansion of one mole of an ideal gas from 10 atm to 1atm at 300 k is
View SolutionCalculate the work done during isothermal reversible expansion expansion of one mol of an ideal gas from 10 atm to 1 atm at 300K.
View SolutionCalculate the work done during isothermal reversible expansion expansion of one mol of an ideal gas from 10 atm to 1 atm at 300K.
View SolutionThe pressure-volume of varies thermodynamic process is shown in graphs:
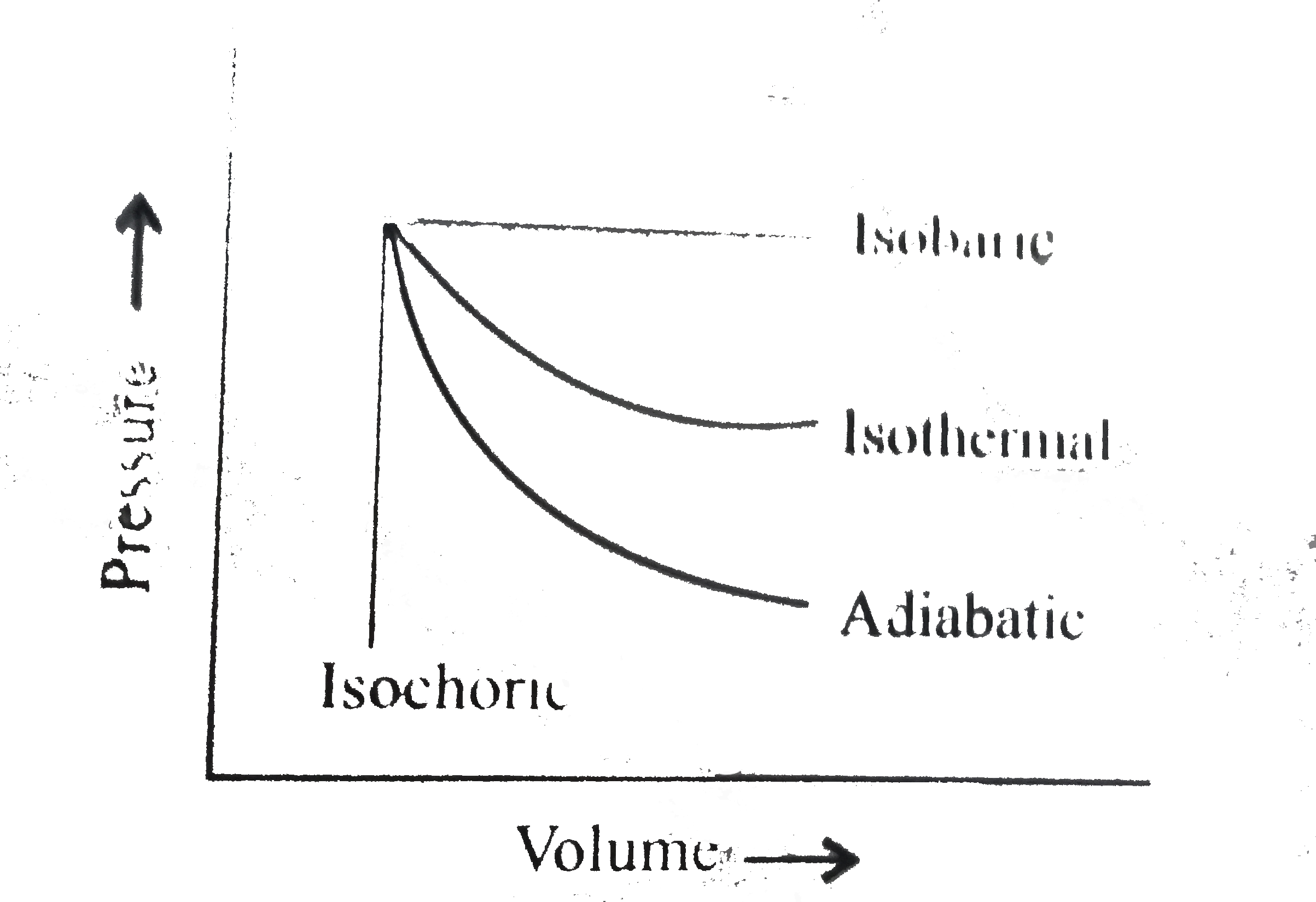
Work is the mole of transference of energy. It has been observed that reversible work done by the system is the maximum obtainable work.
wrev>wirr
The works of isothermal and adiabatic processes are different from each other.
wisothermal reversible=−2.303nRTlog10(V2V1)
=2.303nRTlog10(P2P1)
wadiabatic reversible=CV(T1−T2)
Calculate work done when 1 mole of an ideal gas is expanded reversibly from 30L to 60L at a constant temperature of 300K
View Solution