Calculate the work done when 1 mol of an ideal gas is compressed reversibly from 1 bar to 4 bar at a constant temperature of 300 K
4.01 kJ
−8.02kJ
18.02kJ
3.45kJ
The correct Answer is:D
To calculate the work done when 1 mole of an ideal gas is compressed reversibly from 1 bar to 4 bar at a constant temperature of 300 K, we can use the formula for work done in a reversible isothermal process:
1. Identify the Given Values:
- Number of moles (n) = 1 mol
- Initial pressure (P1) = 1 bar
- Final pressure (P2) = 4 bar
- Temperature (T) = 300 K
- Gas constant (R) = 8.314 J/(mol·K)
2. Use the Formula for Work Done:
The work done (W) during a reversible isothermal process can be calculated using the formula:
W=−nRTln(P2P1)
Here, since we are compressing the gas, we will take the logarithm of the ratio of the final pressure to the initial pressure.
3. Substitute the Values into the Formula:
W=−1×8.314×300×ln(41)
4. Calculate the Natural Logarithm:
ln(4)=1.3863
Thus, we can rewrite the equation:
W=−1×8.314×300×1.3863
5. Perform the Calculation:
W=−1×8.314×300×1.3863≈−3457.69 J
6. Convert the Work Done to Kilojoules:
Since the answer is required in kilojoules, we convert joules to kilojoules:
W=−3457.69 J×1 kJ1000 J=−3.45769 kJ
Rounding to two decimal places, we get:
W≈−3.46 kJ
7. Final Answer:
The work done when 1 mole of an ideal gas is compressed reversibly from 1 bar to 4 bar at a constant temperature of 300 K is approximately -3.46 kJ.
- Number of moles (n) = 1 mol
- Initial pressure (P1) = 1 bar
- Final pressure (P2) = 4 bar
- Temperature (T) = 300 K
- Gas constant (R) = 8.314 J/(mol·K)
The work done (W) during a reversible isothermal process can be calculated using the formula:
W=−nRTln(P2P1)
Here, since we are compressing the gas, we will take the logarithm of the ratio of the final pressure to the initial pressure.
W=−1×8.314×300×ln(41)
ln(4)=1.3863
Thus, we can rewrite the equation:
W=−1×8.314×300×1.3863
W=−1×8.314×300×1.3863≈−3457.69 J
Since the answer is required in kilojoules, we convert joules to kilojoules:
W=−3457.69 J×1 kJ1000 J=−3.45769 kJ
Rounding to two decimal places, we get:
W≈−3.46 kJ
The work done when 1 mole of an ideal gas is compressed reversibly from 1 bar to 4 bar at a constant temperature of 300 K is approximately -3.46 kJ.
Topper's Solved these Questions
Similar Questions
The pressure-volume of varies thermodynamic process is shown in graphs:
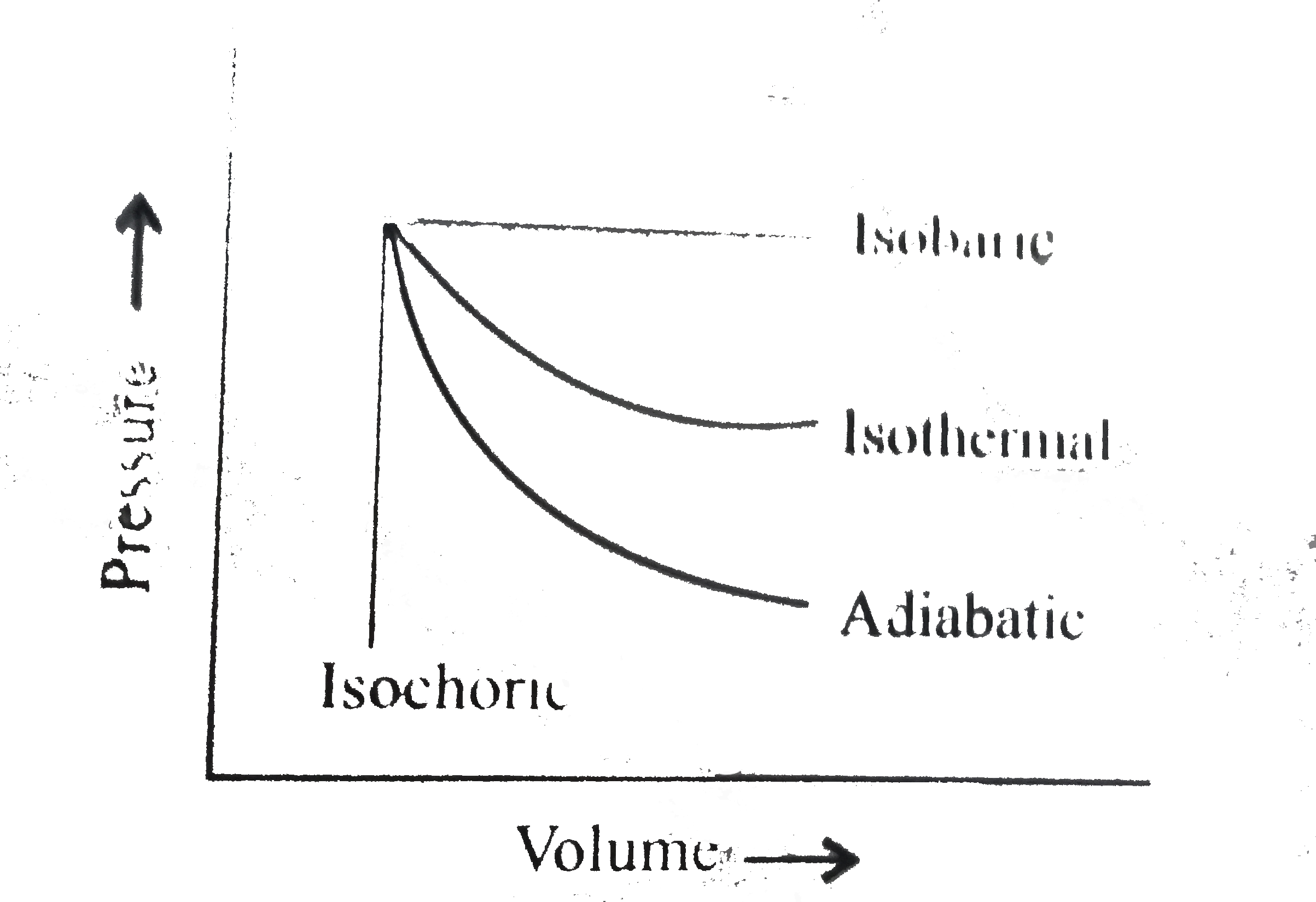
Work is the mole of transference of energy. It has been observed that reversible work done by the system is the maximum obtainable work.
wrev>wirr
The works of isothermal and adiabatic processes are different from each other.
wisothermal reversible=−2.303nRTlog10(V2V1)
=2.303nRTlog10(P2P1)
wadiabatic reversible=CV(T1−T2)
Calculate work done when 1 mole of an ideal gas is expanded reversibly from 30L to 60L at a constant temperature of 300K
View SolutionWhat will be the entropy change when two moles of an ideal gas expand reversibly from initial volume of 1 litre to 10 litre at constant temperature of 300 K?
View Solution
Knowledge Check
What is the work done when 1 mole of a gas expands isothermally from 25 L to 250 L at a constant pressure of 1 atm and a temperature of 300 K ?
A(a) −3542JB(b) −5744JC(c) −2657JD(d) −4890J
Calculate the work done in calories when 5 moles of an ideal gas are compressed isothermally and reversibly from a pressure of 1.5 atm to 15 atm at 27∘C.
View SolutionCalculate the maximum work down when pressure on 10g of hydrogen is reduced from 20 to 1atm at a constant temperature of 273K. The gas behaves ideally. Will there be any change in internal energy. Also calculate q.(R=2calK−1mol−1)
View SolutionCalculate the work done when 1 mole of a gas expands reversibly and isothermally from 5 atm to 1 atm at 300 K. [Value of log 5 = 0.6989].
View SolutionCalculate q,w, and ΔU for the isothermal reversible expansion of one mole of an ideal gas from an initial pressure of 2.0 bar to a final pressure of 0.2 bar at a constant temperature of 273K.
View SolutionWhen an ideal gas is compressed adiabatically and reversibly, the final temperature is:
View Solution1 mole of an ideal gas is expanded from an initial pressure of 1 bar to final pressure of 0.1 bar at constant temperature of 273 K. Predict which of the following is not true?
View SolutionThe pressure-volume of varies thermodynamic process is shown in graphs:
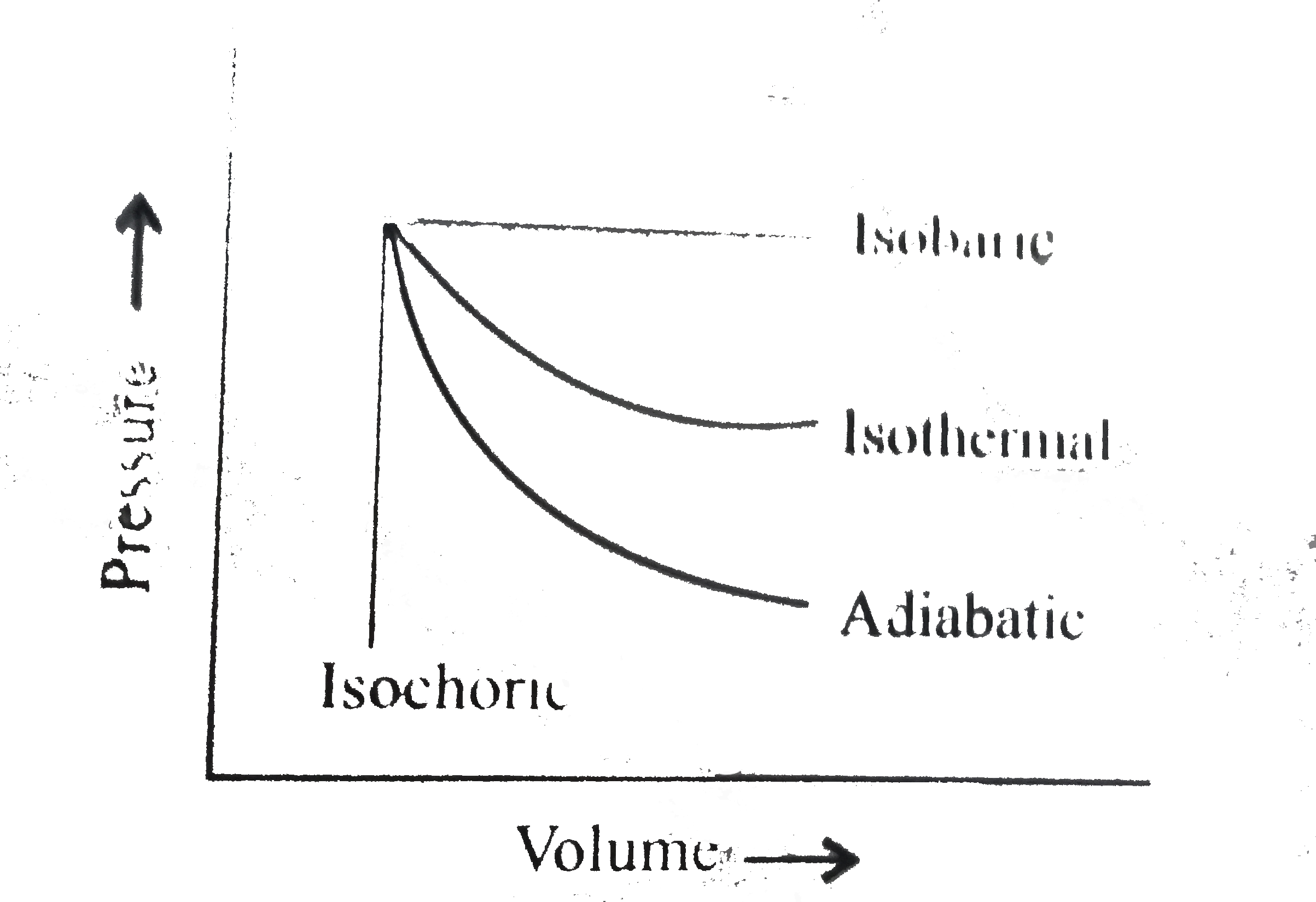
Work is the mole of transference of energy. It has been observed that reversible work done by the system is the maximum obtainable work.
wrev>wirr
The works of isothermal and adiabatic processes are different from each other.
wisothermal reversible=2.303nRTlog10(V2V1)
=2.303nRTlog10(P2P1)
wadiabatic reversible=CV(T1−T2)
The q value and work done in isothermal reversible expansion of one mole of an ideal gas from initial pressure of 1 bar to final pressure of 0.1 bar at constant temperature 273K are:
View Solution
