A dilute solution NaCI was placed between two Pt electrodes 8 cm apart across which a potential of 4V was appied how far would the Na+ move in 2.5 hour? Ionic conductance of Na+ at infinit e dikution at 25∘C is 50.11 mho cm2
To solve the problem step by step, we will follow the outlined approach from the transcript while providing a clear and structured solution.
Step 1: Understand the Concept of Ionic Mobility
Ionic mobility (μ) is defined as the speed of an ion under the influence of an electric field. It can be expressed mathematically as:
μ=sE
where s is the speed of the ion and E is the potential gradient (voltage per unit length).
Step 2: Calculate the Potential Gradient
The potential gradient E can be calculated using the formula:
E=VL
where V is the applied voltage and L is the distance between the electrodes. Given:
- V=4V
- L=8cm=0.08m
Calculating E:
E=4V0.08m=50V/m
Step 3: Relate Ionic Mobility to Conductance
The ionic mobility is also related to the equivalent conductance (λe) by the formula:
μ=λeF
where F is Faraday's constant (96500C/mol). Given that the ionic conductance of Na+ at infinite dilution is 50.11mho cm2:
λe=λionic=50.11mho cm2=50.11×10−4mho m2
Step 4: Calculate Ionic Mobility
Substituting the values into the mobility equation:
μ=50.11×10−496500
Calculating μ:
μ≈5.19×10−7m2/V s
Step 5: Calculate Speed of Na+
The speed s of the ion can be calculated as:
s=μ⋅E
Substituting the values:
s=(5.19×10−7)⋅(50)=2.595×10−5m/s
Step 6: Convert Time to Seconds
Convert the time from hours to seconds:
Time=2.5hours=2.5×60×60=9000s
Step 7: Calculate Distance Moved by Na+
Using the formula for distance:
Distance=s⋅Time
Substituting the values:
Distance=(2.595×10−5)⋅(9000)≈0.23355m=23.36cm
Final Answer
The Na+ ion would move approximately 2.34 cm in 2.5 hours.
---
Ionic mobility (μ) is defined as the speed of an ion under the influence of an electric field. It can be expressed mathematically as:
μ=sE
where s is the speed of the ion and E is the potential gradient (voltage per unit length).
The potential gradient E can be calculated using the formula:
E=VL
where V is the applied voltage and L is the distance between the electrodes. Given:
- V=4V
- L=8cm=0.08m
E=4V0.08m=50V/m
The ionic mobility is also related to the equivalent conductance (λe) by the formula:
μ=λeF
where F is Faraday's constant (96500C/mol). Given that the ionic conductance of Na+ at infinite dilution is 50.11mho cm2:
λe=λionic=50.11mho cm2=50.11×10−4mho m2
Substituting the values into the mobility equation:
μ=50.11×10−496500
Calculating μ:
μ≈5.19×10−7m2/V s
The speed s of the ion can be calculated as:
s=μ⋅E
Substituting the values:
s=(5.19×10−7)⋅(50)=2.595×10−5m/s
Convert the time from hours to seconds:
Time=2.5hours=2.5×60×60=9000s
Using the formula for distance:
Distance=s⋅Time
Substituting the values:
Distance=(2.595×10−5)⋅(9000)≈0.23355m=23.36cm
The Na+ ion would move approximately 2.34 cm in 2.5 hours.
Topper's Solved these Questions
- ELECTROCHEMISTRY BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROCHEMISTRY EXERCISE - Assignment (SECTION - J) (Aakash Challengers Questions)
10 Videos
- ELECTROCHEMISTRY BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROCHEMISTRY EXERCISE - Assignment (SECTION - H) (Multiple True-False Type Question)
3 Videos
- COORDINATION COMPOUNDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - COORDINATION COMPOUNDS EXERCISE - Assignment (Section-J Aakash Challenger Questions )
10 Videos
- ENVIRONMENTAL CHEMISTRYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ENVIRONMENTAL CHEMISTRYEXERCISE - ASSIGNMENT (SECTION-D) (Assertion - Reason Type Questions)
4 Videos
Similar Questions
A dilute aqueous solution of KCIwas placed between two electrodes 10 cm apart, across which a potential of 6 volt was applied. How far would the K+ ion move in 2 hours at 25∘C? Ionic conductance of K+ ion at infinite dilution at 25∘C is 73.52ohm−1cm2mole−1?
View SolutionA dilute solution of KCl was placed between two Pt electrodes 10cm apart across which a potential difference of 10 volt was applied. Which is /are correct statement (Given: molar conductivity of K+ at infinite dilution is 96.5Scm2mol−1
View Solution0.5N solution of a salt placed between two Pt electrodes 2.0 cm apart and having area of cross-section 2.5 cm2 has resistance of 25 ohms. Calculate the conductance and cell constant.
View SolutionThe resistance of decinormal solution of a salt occupying a volume between two platinum electrodes 1.80cm apart and 5.4cm2 area was found to be 32ohm. Calculate k and ∧eq.
View SolutionThe conductance of 0.0015 M aqueous solution of a weak monobasic acid was determined by using a conductivity cell consisting of platinized Pt electrodes. The distance between the electrodes is 120 cm with an area of cross-section of 1cm2. The conductance of this solution was found to be 5×10−7S. The pH of the solution is 4. Calculate the value of limiting molar conductivity.
View SolutionA sample of water from a large swimming pool has a resistance of 10000Ω at 25∘C when placed in a certain conductace cell. When filled with 0.02MKCI solution, the cell has a resistance of 100Ω at 25∘C,585gm of NaCI were dissolved in the pool, which was throughly stirred. A sample of this solution gave a resistance of 8000Ω.
Given: Molar conductance of NaCI at that concentration is 125Ω−1cm2mol−1 and molar conductivity of KCI at 0.02M is 200W−1cm2mol−1.
Conductivity (Scm−1) of H2O is:
View SolutionA sample of water from a large swimming pool has a resistance of 10000Ω at 25∘C when placed in a certain conductace cell. When filled with 0.02MKCI solution, the cell has a resistance of 100Ω at 25∘C,585gm of NaCI were dissolved in the pool, which was throughly stirred. A sample of this solution gave a resistance of 8000Ω.
Given: Molar conductance of NaCI at that concentration is 125Ω−1cm2mol−1 and molar conductivity of KCI at 0.02M is 200W−1cm2mol−1.
Cell constant (in cm−1) of conductane cell is:
View SolutionA sample of water from a large swimming pool has a resistance of 10000Ω at 25∘C when placed in a certain conductace cell. When filled with 0.02MKCI solution, the cell has a resistance of 100Ω at 25∘C,585gm of NaCI were dissolved in the pool, which was throughly stirred. A sample of this solution gave a resistance of 8000Ω.
Given: Molar conductance of NaCI at that concentration is 125Ω−1cm2mol−1 and molar conductivity of KCI at 0.02M is 200W−1cm2mol−1.
Volume (in Litres) of water in the pool is:
View SolutionTwo parallel plate capacitors A and B have the same separation d=8.85×10−4m between the plates. The plate area of A and B are 0.04m2 and 0.02m2 respectively. A slab of dielectric constant (relative permittivity) K=9 has dimensions such that it can exactly fill the space between the plates of capacitor B.
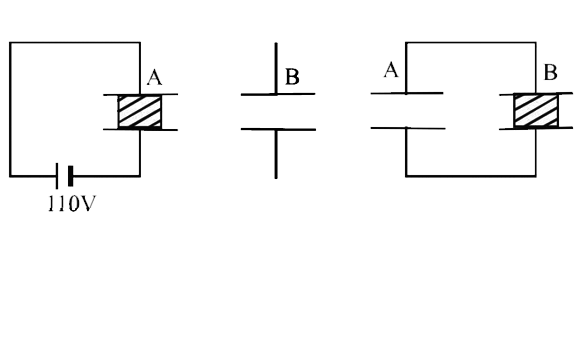
(i) The dielectric slab is placed inside. A as shown in figure (a). A is then charged to a potential difference of 110V. Calculate the capacitance of A and the energy stored in it.
The battery is disconnected and then the dielectric slab is moved from A. Find the work done by the external agency in removing the slab from A.
(iii) The same dielectric slab is now placed inside B, filling it completely, The two capacitors A and B are then connected as shown in figure(c). Calculate the energy stored in the system.
View SolutionThe resistance of 0.5M solution of an electrolyte in a cell was found to be 50Ω. If the electrodes in the cell are 2.2cm apart and have an area of 4.4cm2 then the molar conductivity (in Sm2mol−1) of the solution is
View Solution
