Displacement of a person moving from A to B along a semicircular path of radius R is 100 m. What is the distance travelled by him ?
To solve the problem of finding the distance traveled by a person moving from point A to point B along a semicircular path of radius R, given that the displacement is 100 m, we can follow these steps:
1. Understanding Displacement:
- Displacement is the shortest distance between the initial and final positions. In this case, the displacement from point A to point B along the semicircular path is given as 100 m.
2. Relating Displacement to Radius:
- In a semicircular path, the displacement (straight line from A to B) is equal to the diameter of the semicircle. The diameter D is given by:
D=2R
- Since the displacement is 100 m, we can set up the equation:
2R=100m
- From this, we can find the radius R:
R=1002=50m
3. Calculating the Distance Traveled:
- The distance traveled along the semicircular path is the arc length of the semicircle. The formula for the arc length L of a semicircle is:
L=πR
- Now substituting the value of R:
L=π×50m
- Using the approximate value of π≈3.14:
L≈3.14×50=157m
4. Final Result:
- Therefore, the distance traveled by the person along the semicircular path is approximately:
Distance traveled≈157m
- Displacement is the shortest distance between the initial and final positions. In this case, the displacement from point A to point B along the semicircular path is given as 100 m.
- In a semicircular path, the displacement (straight line from A to B) is equal to the diameter of the semicircle. The diameter D is given by:
D=2R
- Since the displacement is 100 m, we can set up the equation:
2R=100m
- From this, we can find the radius R:
R=1002=50m
- The distance traveled along the semicircular path is the arc length of the semicircle. The formula for the arc length L of a semicircle is:
L=πR
- Now substituting the value of R:
L=π×50m
- Using the approximate value of π≈3.14:
L≈3.14×50=157m
- Therefore, the distance traveled by the person along the semicircular path is approximately:
Distance traveled≈157m
Topper's Solved these Questions
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Try Yourself
40 Videos
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - A)
50 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - D)
15 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - SECTION D
16 Videos
Similar Questions
Knowledge Check
A particle is moving on a circular path of radius r with uniform speed v. What is the displacement of the particle after it has described an angle of 60∘?
Ar√2Br√3CrD2r
A girl runs along a circular track of radius 5 m starting from point A. What will be the distance travelled by her and speed
if she stops halfway at B in 400 s?
View SolutionA girl runs along a circular track of radius 5 m starting from point A. What will be the distance travelled by her and speed
if she comes back to the initial position in 300 s
View SolutionA particle of-mass m moves along a circular path of radius r with velocity v. What is its instantaneous angular momentum about a point on the plane of the orbit at a distance x from the centre of the axis of rotation.
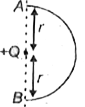
View SolutionThe work done in slowly moving an electron of charge e and mass m from A to B along semicircular path (as shown in the figure ) in vertical plane in the field of charge Q is .
View SolutionA particle is moving in a circular path of radius r. The displacement after half a circle would be
View SolutionFind the centre of mass of a uniform semicircular ring of radius R and mass M.
View SolutionA person runs along a circular path of radius 5 m. Find the magnitude of the displacement if he runs all the way around the circle ?
View Solution
