A particle of-mass m moves along a circular path of radius r with velocity v. What is its instantaneous angular momentum about a point on the plane of the orbit at a distance x from the centre of the axis of rotation.
To find the instantaneous angular momentum of a particle of mass m moving along a circular path of radius r with velocity v about a point O at a distance x from the center of the circular path, we can follow these steps:
Step 1: Understand the Concept of Angular Momentum
Angular momentum L about a point is given by the formula:
L=m⋅v⋅rOA
where rOA is the position vector from point O to point A (the position of the particle).
Step 2: Define the Position Vectors
Let:
- O be the point at a distance x from the center C of the circular path.
- A be the position of the particle on the circular path.
The position vector →rOA can be expressed as:
→rOA=→r−→x
where →r is the position vector of point A from the center C, and →x is the position vector of point O from the center C.
Step 3: Calculate the Position Vector
In the circular motion, the position vector →r of the particle is:
→r=r^r
where ^r is the unit vector in the direction of the radius.
The position vector →x of point O is:
→x=x^i
where ^i is the unit vector along the horizontal direction.
Thus, we have:
→rOA=r^r−x^i
Step 4: Substitute into the Angular Momentum Formula
Now substituting →rOA into the angular momentum formula:
L=m⋅v⋅(r^r−x^i)
Step 5: Calculate the Cross Product
The angular momentum can be expressed as:
L=m⋅v⋅(r^r−x^i)
This can be simplified using the properties of the cross product. The angular momentum about point O is:
L=mv⋅(^r×r^r−^i×x^i)
Since ^r×^r=0 and ^i×^i=0, we focus on the term involving x:
L=mv⋅(r^r×^i)
Step 6: Final Expression
Thus, the final expression for the instantaneous angular momentum about point O is:
L=mv(r−x)
Angular momentum L about a point is given by the formula:
L=m⋅v⋅rOA
where rOA is the position vector from point O to point A (the position of the particle).
Let:
- O be the point at a distance x from the center C of the circular path.
- A be the position of the particle on the circular path.
→rOA=→r−→x
where →r is the position vector of point A from the center C, and →x is the position vector of point O from the center C.
In the circular motion, the position vector →r of the particle is:
→r=r^r
where ^r is the unit vector in the direction of the radius.
→x=x^i
where ^i is the unit vector along the horizontal direction.
→rOA=r^r−x^i
Now substituting →rOA into the angular momentum formula:
L=m⋅v⋅(r^r−x^i)
The angular momentum can be expressed as:
L=m⋅v⋅(r^r−x^i)
This can be simplified using the properties of the cross product. The angular momentum about point O is:
L=mv⋅(^r×r^r−^i×x^i)
Since ^r×^r=0 and ^i×^i=0, we focus on the term involving x:
L=mv⋅(r^r×^i)
Thus, the final expression for the instantaneous angular momentum about point O is:
L=mv(r−x)
Topper's Solved these Questions
- BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)24videos
CIRCULAR MOTION
- BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM MOMENT OF INERTIA, TORQUE)22videos
CIRCULAR MOTION
- BOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - OBJECTIVE QUESTIONS FROM PREVIOUS IAS EXAMINATIONS50videos
COMPETITION CARE UNIT
Similar Questions
A particle of mass m is moving on a circular path of radius r with uniform speed v , rate of change of linear momentum is
View SolutionA particle of mass 'm' is moving on a circular path of radius 'r' with uniform speed 'v'. Rate of change of linear momentum is
View SolutionA particle of mass m moves along line PC with velocity v as shown. What is the angular momentum of the particle about O?
 View Solution
View SolutionA particle of mass m is rotating in a plane in circular path of radius r. Its angular momentum is L. The centripetal force acting on the particle is
View SolutionA particle of mass m is moving with constant velocity v parallel to the x-axis as shown in the figure. Its angular momentum about origin O is
 View Solution
View SolutionA particle of charge 'q' and mass 'm' move in a circular orbit of radius 'r' with frequency 'v' the ratio of the magnetic moment to angular momentum is:
View SolutionA particle of mass 50 gm moving in a circle at 5 cm radius with a constant speed of 10 cm/s. What is its angular momentum about (i) centre of the circle, (ii) a point on the axis of the circle at 4 cm distance from its centre ? Which of these will be always in the same direction ? [Fig.]
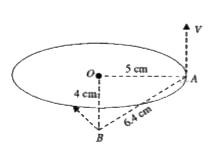 View Solution
View SolutionA Particle of mass 'M' moves in a uniform circular path of radius 'r' with a constant speed 'v' then its centripetal acceleration is .
View SolutionA satellite of mass m is in a circular orbit of radius r round the Earth. Calculate its angular momentum with respect to the centre of the orbit in terms of the mass M of the Earth and G.
View Solution
A particle is moving on a circular path of radius r with uniform velocity v. The change in velocity when the particle moves from P to Q is (∠POQ=40∘)View Solution

