Shown that work done by a conservative force on a particle moving between two points is path independent.
To show that the work done by a conservative force on a particle moving between two points is path independent, we can follow these steps:
Step 1: Define the Points and Paths
Let us consider two points, A and B. There are multiple paths that can be taken to move from point A to point B. For our analysis, we will consider two specific paths: Path 1 and Path 2.
Step 2: Understand Conservative Forces
A conservative force is defined as a force for which the work done is independent of the path taken and depends only on the initial and final positions. A key property of conservative forces is that the net work done over a complete cycle is zero.
Step 3: Work Done in a Complete Cycle
If we move from point A to point B along Path 1 and then return from B to A along the same Path 1, the total work done in this complete cycle is zero:
W(1)AB+W(1)BA=0
This implies:
W(1)AB=−W(1)BA
where W(1)AB is the work done from A to B along Path 1, and W(1)BA is the work done from B to A along Path 1.
Step 4: Work Done Along Different Paths
Now, consider moving from A to B along Path 1 and returning from B to A along Path 2. The total work done in this case is also zero:
W(1)AB+W(2)BA=0
This implies:
W(1)AB=−W(2)BA
Step 5: Relate the Work Done
From the two equations derived, we have:
1. W(1)AB=−W(1)BA
2. W(1)AB=−W(2)BA
Setting these equal gives:
−W(1)BA=−W(2)BA
This simplifies to:
W(1)BA=W(2)BA
Step 6: Conclusion
Since the work done in returning from B to A is the same for both paths, we conclude that the work done by a conservative force on a particle moving between two points A and B is indeed path independent.
Final Statement
Thus, we have shown that the work done by a conservative force on a particle moving between two points is independent of the path taken.
---
Let us consider two points, A and B. There are multiple paths that can be taken to move from point A to point B. For our analysis, we will consider two specific paths: Path 1 and Path 2.
A conservative force is defined as a force for which the work done is independent of the path taken and depends only on the initial and final positions. A key property of conservative forces is that the net work done over a complete cycle is zero.
If we move from point A to point B along Path 1 and then return from B to A along the same Path 1, the total work done in this complete cycle is zero:
W(1)AB+W(1)BA=0
This implies:
W(1)AB=−W(1)BA
where W(1)AB is the work done from A to B along Path 1, and W(1)BA is the work done from B to A along Path 1.
Now, consider moving from A to B along Path 1 and returning from B to A along Path 2. The total work done in this case is also zero:
W(1)AB+W(2)BA=0
This implies:
W(1)AB=−W(2)BA
From the two equations derived, we have:
1. W(1)AB=−W(1)BA
2. W(1)AB=−W(2)BA
−W(1)BA=−W(2)BA
This simplifies to:
W(1)BA=W(2)BA
Since the work done in returning from B to A is the same for both paths, we conclude that the work done by a conservative force on a particle moving between two points A and B is indeed path independent.
Thus, we have shown that the work done by a conservative force on a particle moving between two points is independent of the path taken.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBook:AAKASH INSTITUTE ENGLISHChapter:WORK, ENERGY AND POWERExercise:IILUSTRATION
Explore 2 Videos
- WORK, ENERGY AND POWERBook:AAKASH INSTITUTE ENGLISHChapter:WORK, ENERGY AND POWERExercise:TRY YOURSELF
Explore 95 Videos
- WAVESBook:AAKASH INSTITUTE ENGLISHChapter:WAVESExercise:ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
Explore 12 Videos
Similar Questions
Work done by the conservative force on a system is equal to :
View SolutionThis question contains Statement-1 and Statement-2. Of the four choices given after the statements, choose the one that best describes the two statements.
Statement-1: For a charged particle moving from point P to point Q, the net work done by an electrostatic field on the particle is independent of the path connecting point P to point Q.
Statement-2: The net work done by a conservative force on an object moving along a closed loop is zero.
View Solution
Knowledge Check
The frictional force between two surfaces is independent of
ANature of the surfaceBSize of the bodyCArea of contactDMass of the bodyAssertion:The work done by a conservative force such as gravity depends on the initial and final positions only
Reason: The work done by a force can not be calculated if the exact nature of the force is not known.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true not but reason is not the correct explanation of assertion.CIf assertion is true but reason is falseDIf both assertion and reason are false.Assertion: Work done in moving a charge between any two points in a unifrom electric field is independent of the path followed by the charge, between these points.
Reason: Electrostatic forces are non-conservative.AIf both assertion and reason are ture and reason is the correct explanation of assertion.BIf both assertin and reason are ture but reason is not the correct explanation of assertion .CIf assertion is true but reason is false.DIf both assertion and reason are false.
The potential energy function of a particle in the x-y plane is given by U=k(x+y), where (k) is a constant. The work done by the conservative force in moving a particlae from (1,1) to (2,3) is .
View SolutionSTATEMENT-1: Work-energy theorem is consequence of Newton's laws of motion.
STATEMENT-2: The work done by a conservative force along a closed path is zero.
STATEMENT3: Work done by a constant force is independent of the path.
View SolutionA particle is moving in a conservative force field from point A to point B.UA and UB are the potential energies of the particle at point A and B and WC is the work done by conservative forces in the process of taking the particle from A and B, which of the following is true?
View SolutionA particle moves in x-y plane in figure under the influence of a friction force with magnitude 3.00N and acting in the direction opposite to the particle's displacement. Calculate the work done by the friction force on particle as it moves along the following paths: (a) path OA followed by AC (b) path OA followed by AC and the return path CO, (c) path OC followed by the return path CO, and (d) each of your three answers should be non-zero. What is the significant of this observation?
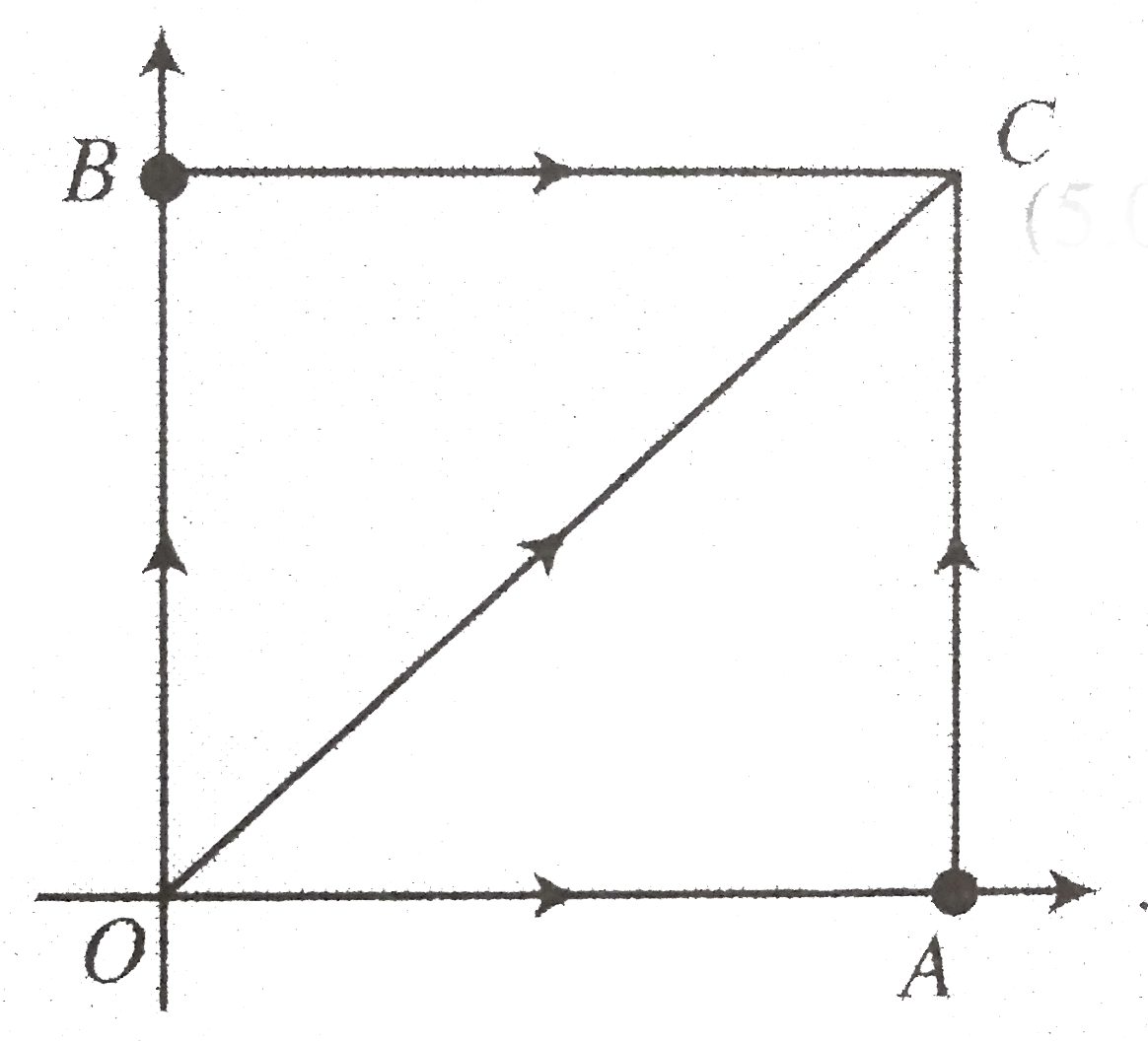
View SolutionWhat is the amount of work done in moving a charge of 15 between two points kept at a potentia difference of 1.2 V?
View Solution
