Three equal masses of 1.5 kg each are fixed at the vertices of an equilateral triangle of side 1 m. What is the force acting on a particle of mass 1 kg placed at its centroid ?
The correct Answer is:Zero
To solve the problem of finding the force acting on a particle of mass 1 kg placed at the centroid of an equilateral triangle with three equal masses of 1.5 kg each at its vertices, we can follow these steps:
1. Identify the Setup:
We have three masses, each of 1.5 kg, located at the vertices of an equilateral triangle with a side length of 1 m. A particle of mass 1 kg is placed at the centroid of this triangle.
2. Calculate the Distance from the Centroid to the Vertices:
The distance from the centroid of an equilateral triangle to any of its vertices can be calculated using the formula:
d=a√3
where a is the side length of the triangle. For our triangle:
d=1m√3≈0.577m
3. Calculate the Gravitational Force from Each Mass:
The gravitational force F exerted by each mass on the 1 kg mass can be calculated using Newton's law of gravitation:
F=G⋅m1⋅m2r2
where:
- G is the gravitational constant (6.674×10−11N m2/kg2),
- m1=1.5kg (mass at the vertex),
- m2=1kg (mass at the centroid),
- r=0.577m (distance from the centroid to the vertex).
Plugging in the values:
F=(6.674×10−11)⋅(1.5)⋅(1)(0.577)2≈(6.674×10−11)⋅1.50.333≈3.0×10−10N
4. Determine the Direction of Forces:
Each of the three forces acts along the line connecting the centroid to each vertex. The angles between the forces from different vertices are 120 degrees.
5. Calculate the Net Force:
Since the forces are equal in magnitude and symmetrically placed, we can analyze the components of these forces. The angle between any two forces is 120 degrees, which means the net force can be calculated using vector addition.
The resultant of three equal forces F at angles of 120 degrees can be shown to be zero due to symmetry. This can be confirmed by resolving the forces into components along the x and y axes. The components in one direction will cancel out the components in the opposite direction.
Thus, the net force acting on the mass at the centroid is:
Fnet=0N
Final Answer:
The force acting on the particle of mass 1 kg placed at the centroid is 0 N.
We have three masses, each of 1.5 kg, located at the vertices of an equilateral triangle with a side length of 1 m. A particle of mass 1 kg is placed at the centroid of this triangle.
The distance from the centroid of an equilateral triangle to any of its vertices can be calculated using the formula:
d=a√3
where a is the side length of the triangle. For our triangle:
d=1m√3≈0.577m
The gravitational force F exerted by each mass on the 1 kg mass can be calculated using Newton's law of gravitation:
F=G⋅m1⋅m2r2
where:
- G is the gravitational constant (6.674×10−11N m2/kg2),
- m1=1.5kg (mass at the vertex),
- m2=1kg (mass at the centroid),
- r=0.577m (distance from the centroid to the vertex).
F=(6.674×10−11)⋅(1.5)⋅(1)(0.577)2≈(6.674×10−11)⋅1.50.333≈3.0×10−10N
Each of the three forces acts along the line connecting the centroid to each vertex. The angles between the forces from different vertices are 120 degrees.
Since the forces are equal in magnitude and symmetrically placed, we can analyze the components of these forces. The angle between any two forces is 120 degrees, which means the net force can be calculated using vector addition.
Fnet=0N
The force acting on the particle of mass 1 kg placed at the centroid is 0 N.
Topper's Solved these Questions
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION -A (Objective Type Questions (one option is correct))
50 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION -B (Objective Type Questions (one option is correct))
20 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
15 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - ASSIGNMENT SECTION - D
9 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
Similar Questions
Three masses of 1 kg each are kept at the vertices of an equilateral triangle of side 3 m. What is the force acting on a point mass of 5 kg placed at its centroid ?
View SolutionThree equal masses of mkg each are fixed at the vertices of an equilateral triangle ABC.
a. What is the force acting on a mass 2m placed at the centroid G of the triangle?
b. What is the force if the mass at the vertex A is doubled? Take AG=BG=CG=1m
View Solution
Knowledge Check
Three masses each of mass m are palced at the vertices of an equilateral triangles ABC of side l as shown in figure. The force acting on a mass 2m placed at the centroid O of the triangle is
 AzeroB3Gm2l2C5Gm2l2D7Gm2l2
AzeroB3Gm2l2C5Gm2l2D7Gm2l2
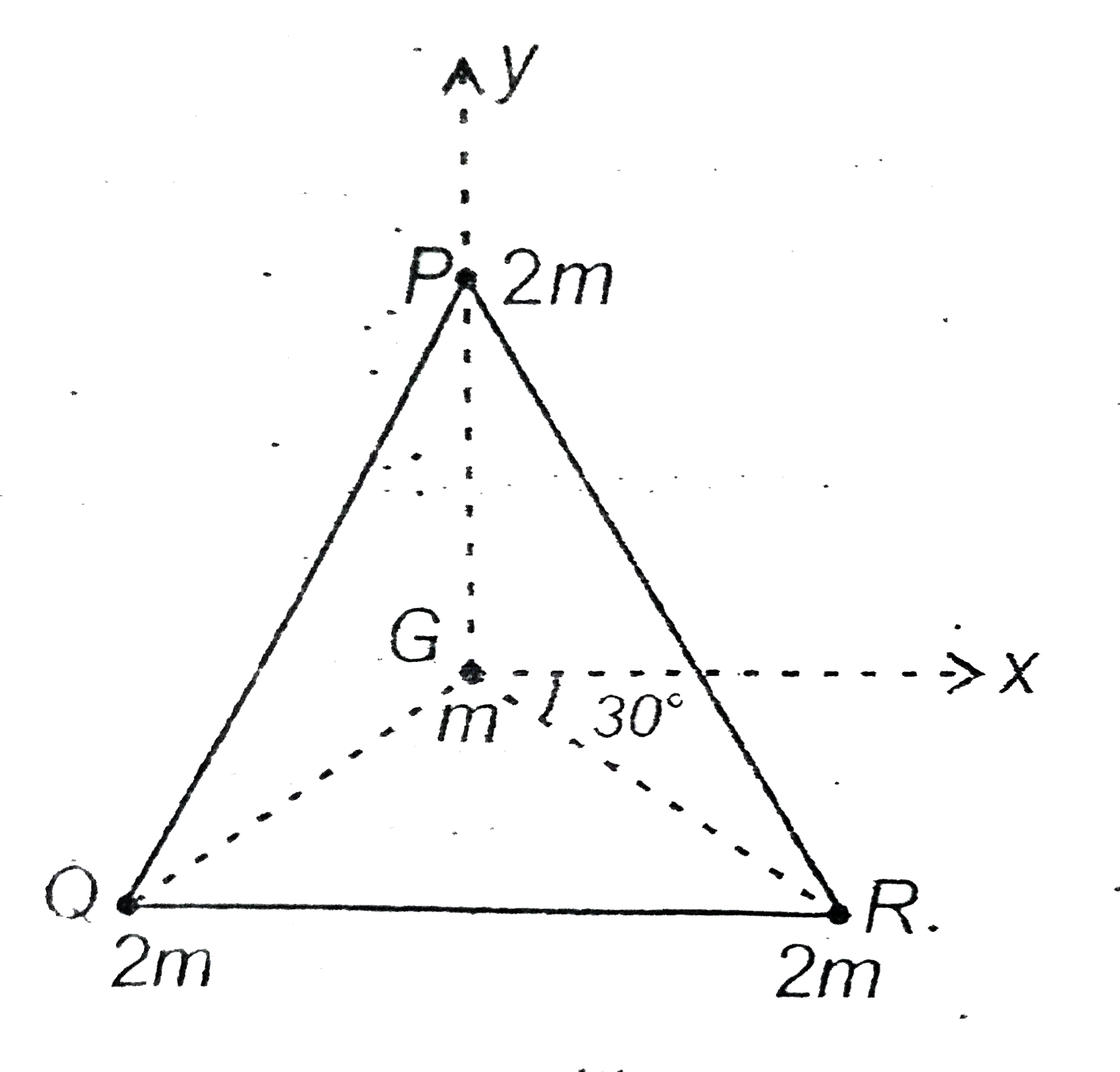
Three equal masses 2m each are placed at the vertices an equilateral triangle PQR
(i) What is the force acting on a mass m placed at the centroid G of the triangle ?
(ii) What is the force on mass m if the mass at the vertex P is quadrupled ?
Take PG = QG = RG = 1m
View SolutionThree equal masses of 1 kg each are placed at the vertices of an equilateral triangle of side 1 m, then the gravitational force on one of the masses due to other masses is (approx.)
View SolutionThree equal masses of 1 kg each are placed at the vertices of an equilateral triangle and other particle of mass 2kg is placed on centroid of triangle which is at a distance of √2 m from each of the vertices of the triangle .The force in newton , acting on the particle of mass 2 kg is
View SolutionThree equal masses m are placed at the three corners of an equilateral triangle of side a. find the force exerted by this system on another particle of mass m placed at (a) the mid point of a side (b) at the center of the triangle.
View SolutionThree identical particles each of mass m are placed at the vertices of an equilateral triangle of side a. Fing the force exerted by this system on a particle P of mass m placed at the

(a) the mid point of a side
(b) centre of the triangle
View SolutionThree masses 3, 4 and 5 kg are placed at the centre of an equiliteral triangle of 1 m sides. Locate its centre of mass.
View SolutionThree equal masses m are placed at the three corners of an equilateral triangle of side a. The force exerted by this system on another particle of mass m placed at the mid-point of a side.
View Solution