The equation of a standing wave in a string fixed at both ends is given as y=2sinkxcosωt
The amplitude and frequency of a particle vibrating at the mid of an antinode and a node are respecetively
A,ω2π
A√2,ω2π
A,ω2π
√2A,ω2π
The correct Answer is:D
Step by step video & image solution for The equation of a standing wave in a string fixed at both ends is given as y=2 sin kx cos omegat The amplitude and frequency of a particle vibrating at the mid of an antinode and a node are respecetively by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - Assignment (Section-C)
11 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - Assignment (Section-D)
9 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - Assignment (Section-A)
55 Videos
- WAVE OPTICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Assignment (Section-J (Aakash Challengers question))
1 Video
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - D)
13 Videos
Similar Questions
The equation of a standing wave in a string fixed at both its ends is given as y=2A sin kx cos ωt. The amplitude and frequency of a particle vibrating at the point of string midway between a node and an antinode is
View SolutionExplain the formation of standing waves in a string clamped at both ends and discuss the various modes of vibration.
View SolutionThe equation of a standing wave produced on a string fixed at both ends is y=0.4sinπx10cos600πt where y' is measured in om 10 What could be the smallest length of string?
View SolutionThe velocity of waves in a string fixed at both ends is 2 m / s . The string forms standing waves with nodes 5.0 cm apart. The frequency of vibration of the string in Hz is
View SolutionA wave is represente by y=Asin2(kx−ωt+ϕ). The amplitude and wavelength of wave is given by
View SolutionThe equation of a standing wave, produced on a string fixed at both ends, is y=(0.4cm)sin[(0.314cm−1)x]cos[(600πs−1)t] What could be the smallest length of the string ?
View SolutionThe displacement equation of a standing wave in a homogeneous elastic medium is given by y=acos(kx)cos(ωt)
Match the physical quantities α in the column (I) to the correct statement in the column (II)
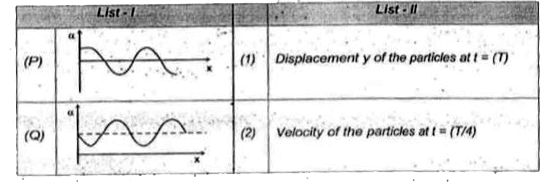

View SolutionThe wave-function for a certain standing wave on a string fixed at both ends is y(x,t)=0.5sin(0.025πx)cos500t where x and y are in centimeters and t is seconds. The shortest possible length of the string is :
View SolutionA travelling wave in a stretched string is described by the equation y=Asin(kx−ωt) the maximum particle velocity is
View SolutionA string of length l is fixed at both ends and is vibrating in second harmonic. The amplitude at anti-node is 5 mm. The amplitude of a particle at distance l/8 from the fixed end is
View Solution