If velocity of bird (B) in downward direction with respect to surface (water and air) is 3m/s. Then what is the velocity of bird (B) with respect to fish (F) ? (.aμw=43)
To find the velocity of the bird (B) with respect to the fish (F), we can follow these steps:
Step 1: Understand the given information
We know that the velocity of the bird (B) in the downward direction with respect to the surface (water and air) is given as 3 m/s. We also have the refractive index (μ) of water with respect to air, which is given as 43.
Step 2: Establish the relationship between the bird's height and the fish's height
The apparent height of the bird (B) as seen from the fish (F) is affected by the refractive index. The apparent height (h') of the bird with respect to the fish can be expressed as:
h′=μ⋅h
where h is the actual height of the bird above the water surface.
Step 3: Differentiate the height to find the velocity
To find the velocity of the bird with respect to the fish, we need to differentiate the apparent height with respect to time:
d(h′)dt=μ⋅dhdt
Here, dhdt is the velocity of the bird (B) with respect to the surface, which is given as 3 m/s.
Step 4: Substitute the values into the equation
Now, substituting the values we have:
d(h′)dt=43⋅3
This simplifies to:
d(h′)dt=4 m/s
Step 5: Conclusion
Thus, the velocity of the bird (B) with respect to the fish (F) is 4 m/s.
Summary of the Solution
The velocity of the bird with respect to the fish is 4 m/s.
---
We know that the velocity of the bird (B) in the downward direction with respect to the surface (water and air) is given as 3 m/s. We also have the refractive index (μ) of water with respect to air, which is given as 43.
The apparent height of the bird (B) as seen from the fish (F) is affected by the refractive index. The apparent height (h') of the bird with respect to the fish can be expressed as:
h′=μ⋅h
where h is the actual height of the bird above the water surface.
To find the velocity of the bird with respect to the fish, we need to differentiate the apparent height with respect to time:
d(h′)dt=μ⋅dhdt
Here, dhdt is the velocity of the bird (B) with respect to the surface, which is given as 3 m/s.
Now, substituting the values we have:
d(h′)dt=43⋅3
This simplifies to:
d(h′)dt=4 m/s
Thus, the velocity of the bird (B) with respect to the fish (F) is 4 m/s.
The velocity of the bird with respect to the fish is 4 m/s.
Similar Questions
If velocity of fish in upward direction is 4 m/s with respect to surface and velocity of bird in downward direction with respect to surface is 3 m/s. Then find the velocity of fish with respect to bird (Given that .aμw=43)
View SolutionThe refractive index of water with respect to air is 4/3. What is the refractive index of aiir with respect to water ?
View SolutionRain is falling vertically downward with velocity 4m/s. A man is moving horizontally with velocity 3m/s, the velocity of rain with respect to man is
View SolutionIn the figure shown the velocity of A with respect to ground is (20ˆi+15ˆj)m/s, then what will be the magnitude of velocity of the wedge 'B' with respect to ground frame. (A remains in contact with B)
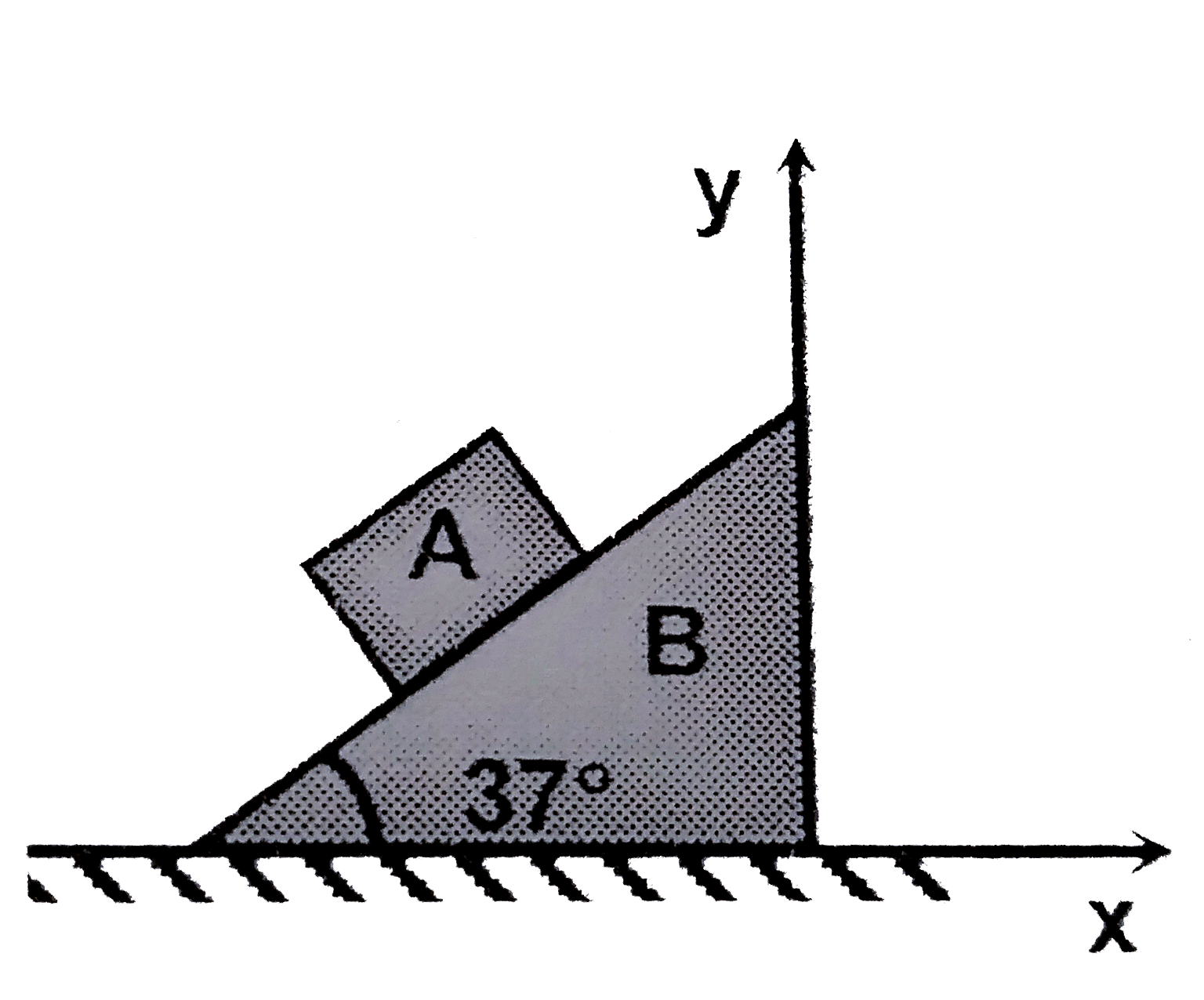
View SolutionA turck is moving on the ground with a velocity v=12m/s and a box is moving on the truck with respect to it with a velocity u=5m/s as shown in the figure. What is the velocity of the box with respect to the ground?

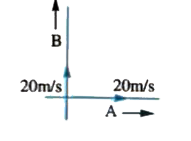
View SolutionConsdier the situation shown in figure
(I ) find out velocity of B with respect to A
(ii ) Find out velocity of A with respect to B
View SolutionIf the refractive index of water with respect to air (aμw) is 53. Calculate the refractive index of air with respect to water (wμa).
View SolutionWhat is the relation between the refractive index of water with respect to air (aμw) and the refractive index of air with respect to water (wμa).
View SolutionA boat is moving with a velocity 3ˆi+4ˆj with respect to ground. The water in the river is moving with a velocity −3ˆi−4ˆj with respect to ground. The relative velocity of the boat with respect to water is.
View SolutionAn object A is moving with 5 m/s and B is moving with 20 m/s in the same direction. (Positive x-axis). Find a) velocity of B relative to A.
b) Velocity of A relative to B
View Solution
