Assuming photoemission to take place , the factor by which the maximum velocity of the emitted photoelectrons changes when the wavelength of the incident radiation is increased four times , is
4
14
2
12
The correct Answer is:4
To solve the problem step by step, we will analyze the relationship between the wavelength of the incident radiation and the maximum velocity of the emitted photoelectrons.
Step 1: Understand the relationship between kinetic energy and wavelength
The maximum kinetic energy (K.E.) of the emitted photoelectrons can be expressed using the equation:
K.E.=12mV2m=hcλ−ϕ
where:
- m is the mass of the electron,
- Vm is the maximum velocity of the emitted photoelectrons,
- h is Planck's constant,
- c is the speed of light,
- λ is the wavelength of the incident radiation,
- ϕ is the work function of the material.
Step 2: Neglect the work function
Assuming that the energy of the incident photons is much greater than the work function (i.e., hcλ≫ϕ), we can neglect ϕ:
12mV2m=hcλ
Step 3: Rearranging the equation
From the above equation, we can express Vm:
V2m=2hcmλ
This shows that V2m is inversely proportional to λ:
V2m∝1λ
Step 4: Relate the velocities for different wavelengths
Let Vm1 be the maximum velocity for the initial wavelength λ1 and Vm2 for the new wavelength λ2. We can write:
V2m1V2m2=λ2λ1
Step 5: Substitute the new wavelength
Given that the wavelength is increased four times, we have:
λ2=4λ1
Substituting this into the equation gives:
V2m1V2m2=4λ1λ1=4
Step 6: Solve for the ratio of velocities
Taking the square root of both sides, we find:
Vm1Vm2=√4=2
This implies:
Vm2=Vm12
Step 7: Determine the factor of change
The factor by which the maximum velocity changes is:
Factor=Vm2Vm1=Vm1/2Vm1=12
Conclusion
Thus, the factor by which the maximum velocity of the emitted photoelectrons changes when the wavelength of the incident radiation is increased four times is:
12
---
The maximum kinetic energy (K.E.) of the emitted photoelectrons can be expressed using the equation:
K.E.=12mV2m=hcλ−ϕ
where:
- m is the mass of the electron,
- Vm is the maximum velocity of the emitted photoelectrons,
- h is Planck's constant,
- c is the speed of light,
- λ is the wavelength of the incident radiation,
- ϕ is the work function of the material.
Assuming that the energy of the incident photons is much greater than the work function (i.e., hcλ≫ϕ), we can neglect ϕ:
12mV2m=hcλ
From the above equation, we can express Vm:
V2m=2hcmλ
This shows that V2m is inversely proportional to λ:
V2m∝1λ
Let Vm1 be the maximum velocity for the initial wavelength λ1 and Vm2 for the new wavelength λ2. We can write:
V2m1V2m2=λ2λ1
Given that the wavelength is increased four times, we have:
λ2=4λ1
Substituting this into the equation gives:
V2m1V2m2=4λ1λ1=4
Taking the square root of both sides, we find:
Vm1Vm2=√4=2
This implies:
Vm2=Vm12
The factor by which the maximum velocity changes is:
Factor=Vm2Vm1=Vm1/2Vm1=12
Thus, the factor by which the maximum velocity of the emitted photoelectrons changes when the wavelength of the incident radiation is increased four times is:
12
Topper's Solved these Questions
- DUAL NATURE OF RADIATION AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - ASSIGNMENT SECTION B.Objective (Only one answer)
6 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - ASSIGNMENT SECTION C. Objective (More than one answer)
4 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - Try Yourself
41 Videos
- CURRENT ELECTRICITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - ASSIGNMENT SECTION-J
10 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - comprehension
3 Videos
Similar Questions
How does K.E. of photoelectrons change when the intensity of radiation is increased?
View SolutionWhen light of sufficiently high frequency is incident on a metallic surface, electrons are emitted from the metallic surface. This phenomenon is called photoelectric emission. Kinetic energy of the emitted photoelectrons depends on the wavelength of incident light and is independent of the intensity of light. Number of emitted photoelectrons depends on intensity. (hv−ϕ) is the maximum kinetic energy of emitted photoelectron (where ϕ is the work function of metallic surface). Reverse effect of photo emission produces X-ray. X-ray is not deflected by electric and magnetic fields. Wavelength of a continuous X-ray depends on potential difference across the tuve. Wavelength of charasteristic X-ray depends on the atomic number.
Q. If potential difference across the tube is increased then
View Solution
Knowledge Check
According to Einstein's photoelectric equation, the plot of the maximum kinetic energy of the emitted photoelectrons from a metal versus frequency of the incident radiation gives a straight line whose slope
Adepends on the intensity of incident radiation.Bdepends on the nature of the metal and also on the intensity of incident radiation.Cis same for all metals and independent of the intensity of the incident radiation.Ddepends on the nature of the metal.
When light of sufficiently high frequency is incident on a metallic surface, electrons are emitted from the metallic surface. This phenomenon is called photoelectric emission. Kinetic energy of the emitted photoelectrons depends on the wavelength of incident light and is independent of the intensity of light. Number of emitted photoelectrons depends on intensity. (hv−ϕ) is the maximum kinetic energy of emitted photoelectron (where ϕ is the work function of metallic surface). Reverse effect of photo emission produces X-ray. X-ray is not deflected by electric and magnetic fields. Wavelength of a continuous X-ray depends on potential difference across the tuve. Wavelength of charasteristic X-ray depends on the atomic number.
Q. If frequency (υ>υ0) of incident light becomes n times the initial frequency (v), then KE of the emitted photoelectrons becomes (v0 threshold frequency).
View SolutionThreshold frequency for a certain metal is v0. When light of frequency 2v0 is incident on it, the maximum velocity of photoelectrons is 4×108cms−1. If frequency of incident radiation is increased to 5v0, then the maximum velocity of photoelectrons, in cm s−1, will be
View SolutionThe threshold frequency for certain metal is v0. When light of frequency 2v0 is incident on it, the maximum velocity of photoelectrons is 4×106ms−1. If the frequency of incident radiation is increaed to 5v0, then the maximum velocity of photoelectrons will be
View SolutionLight of wavelength 3000Å is incident on a metal surface whose work function is 1 eV. The maximum velocity of emitted photoelectron will be
View SolutionConsider the two following statements I and II, and identify the correct choice given in the answers
1. In photovoltaic cells, the photoelectric current produced is not proportional to the intensity of incident light.
2. In gas-filled photoemissive cells, the velocity of photoelectrons depends on the wavelength of the incident radiation.
View SolutionA If the wavelength of the incident radiation changes from λ1 to λ2 then the maximum kinetic energy of the emitted photo electrons changes from K to K,, then the work function of the emitter surface is
View SolutionFig. shows the variation of stopping potential V0 with the frequency v of the incident radiation for two photosensitive metal P and Q:
(i) Explain which metal has smaller threshold wavelength
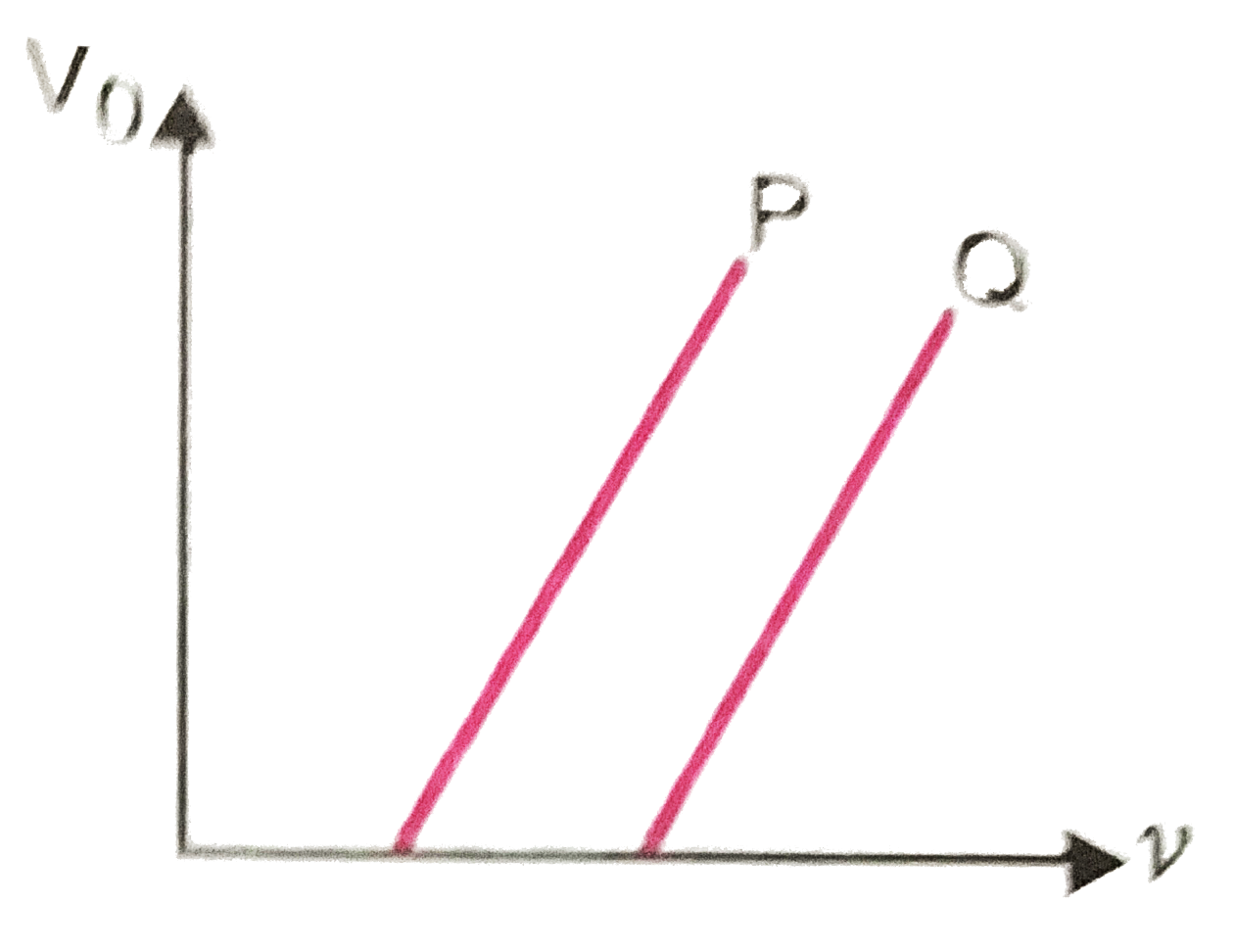
(ii) Explain, giving reason, which metal emits photoelectrons having smaller kinetic energy, for the same wavelength of incident radiation.
(iii) If the distance between the light source and metal P is doubled, how will the stopping potential change.
View Solution
