The number of neutrons released when .92U235 undergoes fission by absorbing .0n1 and (.56Ba144+.36Kr89) are formed, is.
2
3
1
0
The correct Answer is:B
To solve the problem of determining the number of neutrons released during the fission of Uranium-235, we can follow these steps:
1. Write the Fission Reaction:
The fission of Uranium-235 when it absorbs a neutron can be represented as:
23592U+10n→14456Ba+8936Kr+x10n
Here, x represents the number of neutrons released.
2. Conservation of Mass Number:
In nuclear reactions, the mass number must be conserved. We will set up an equation based on the mass numbers on both sides of the reaction.
- Left-hand side (LHS) mass number:
235+1=236
- Right-hand side (RHS) mass number:
144+89+x
3. Set Up the Equation:
Now we equate the total mass numbers from both sides:
236=144+89+x
4. Calculate the Right-Hand Side:
First, calculate 144+89:
144+89=233
Now, substitute this back into the equation:
236=233+x
5. Solve for x:
Rearranging the equation gives:
x=236−233
Therefore:
x=3
6. Conclusion:
The number of neutrons released during the fission of Uranium-235 is 3.
Final Answer:
The number of neutrons released is 3.
---
The fission of Uranium-235 when it absorbs a neutron can be represented as:
23592U+10n→14456Ba+8936Kr+x10n
Here, x represents the number of neutrons released.
In nuclear reactions, the mass number must be conserved. We will set up an equation based on the mass numbers on both sides of the reaction.
- Left-hand side (LHS) mass number:
235+1=236
- Right-hand side (RHS) mass number:
144+89+x
Now we equate the total mass numbers from both sides:
236=144+89+x
First, calculate 144+89:
144+89=233
Now, substitute this back into the equation:
236=233+x
Rearranging the equation gives:
x=236−233
Therefore:
x=3
The number of neutrons released during the fission of Uranium-235 is 3.
The number of neutrons released is 3.
Topper's Solved these Questions
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - Assignment Section B Objective (One option is correct )
10 Videos
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - Assignment Section C Objective (More than one option are correct )
10 Videos
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - Try Yourself
36 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Assignment Section J (Aakash Challengers Questions)
5 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - Assignment (Section D) (ASSERTION-REASON TYPE QUESTIONS)
13 Videos
Similar Questions
In the given arrangement the block is released from the position where the spring is unstretched. The speed of the block when it has descended through 2cm is x10. Find the value of x.
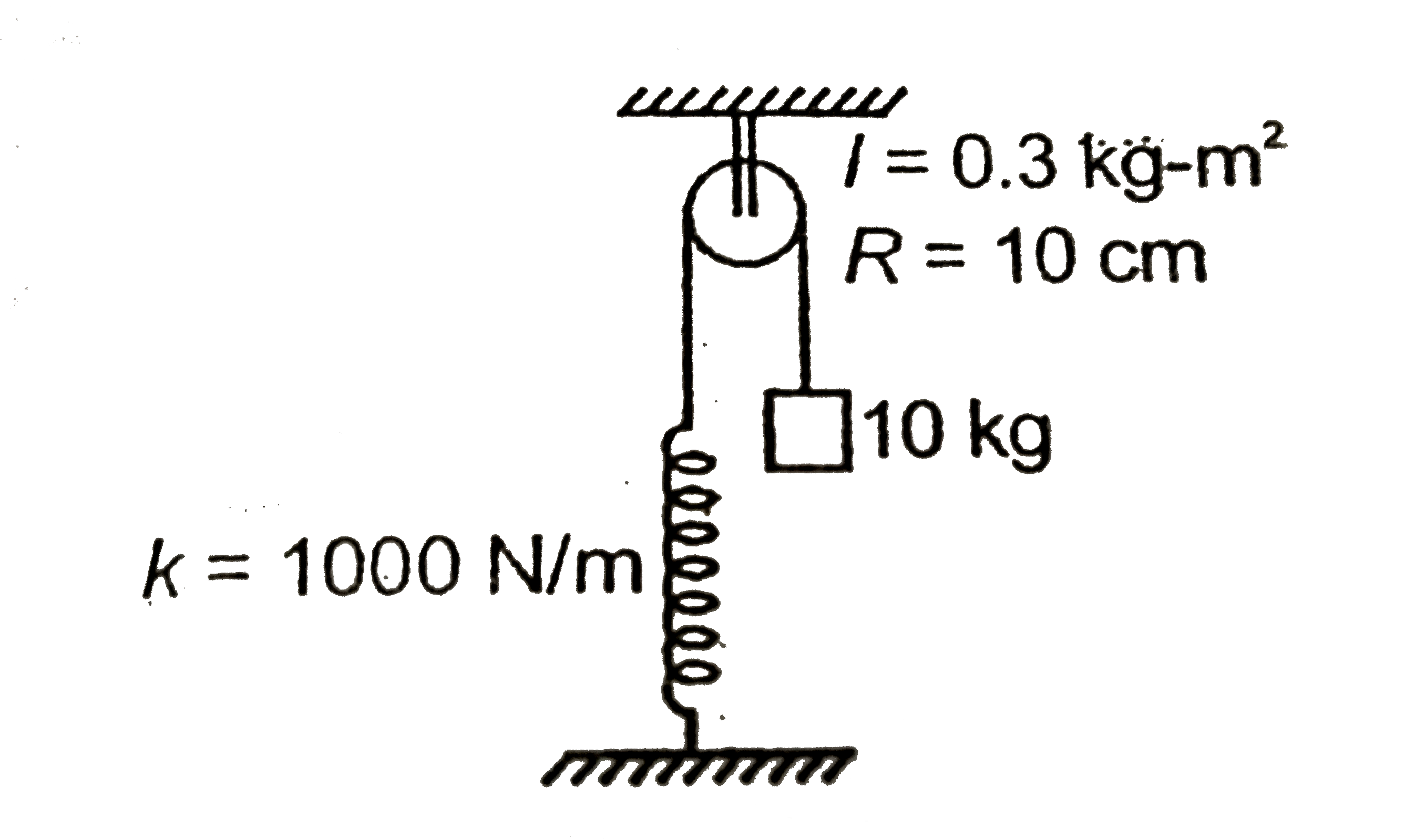
View SolutionCalculate the number of neutrons emitted when .92U235 undergoes controlled nuclear fission to .54Xe142 and .38Sr90.
View SolutionFind the amount of energy released when 1 atom of Uranium .92U235(235.0439amu) undergoes fission by slow neturon (1.0087amu) and is splitted into Krypton .36Kr92(91.8973amu) and Barium .56Br141(140.9139amu) assuming no energy is lost. Hence find the enrgy in kWh, when 1g of it undergoes fission.
View SolutionThe .92U235 absorbs a slow neturon (thermal neutron) & undergoes a fission represented by .92U235+.0n1→.92U236→.56Ba141+36Kr92+30n1+E. Calculate:
The energy released when 1g of .92U235 undergoes complete fission in N if m=[N] then find m−25. [N] greatest integer
Given
.92U235=235.1175amu (atom),
.56Ba141=140.9577amu (atom),
.36r92=91.9263amu(atom),.0n1=1.00898amu,1amu=931MeV/C2
View SolutionIn equation .92U235+.0n1→.56Ba144+.36Kr89+X : X is-
View Solution200 Mev energy is released when one nucleus of .235U undergoes fission. Find the number of fissions per second required for producing a power of 1 mega watt.
View SolutionA neutron strikes a 92U235 nucleus and as a result 36Kr93and56Ba140 are produced with
View Solution92U235 mucleus absorbs a slow neutron and undergoes fission into 54X139 and 38sr94 nuclie The other particies produced in this fission process are
View SolutionThe isotopic masses of .1H2 and .2He4 are 2.0141 and 4.0026 amu, respectively. Calculate the quantity of energy liberated when two moles of .1H2 undergo fission to form 1 mol of .2He4. The velocity of light in vacuum is 3.0×108ms−1.
View SolutionIf an atom of _92235U, after absorbing a slow neutron, undergoes fission to form an atom of −54138Xe and an atom of _3894Sr, the other particles produced are
View Solution
