A far off planet is estimated to be at a distance D from the earth. If its diametrically opposite extremes subtend an angle θ at an observatory situated on the earth, the approximate diameter of the planet is
θD
Dθ
Dθ
1Dθ
The correct Answer is:3
To solve the problem of finding the approximate diameter of a far-off planet that subtends an angle θ at an observatory on Earth, we can follow these steps:
Step 1: Understand the Geometry
We have an observatory on Earth and a planet at a distance D. The planet's diametrically opposite extremes subtend an angle θ at the observatory. We can visualize this scenario as a triangle formed by the observatory and the two extremes of the planet.
Step 2: Define the Variables
Let:
- D = distance from the observatory to the planet
- d = diameter of the planet
- The angle subtended at the observatory is θ.
Step 3: Consider Half of the Angle
Since the diameter subtends the angle θ, each half of the diameter subtends an angle of θ2.
Step 4: Use Trigonometric Relationships
In the triangle formed (let's label the observatory point as O, one extreme of the planet as A, and the other extreme as C), we can use the tangent function:
tan(θ2)=oppositeadjacent=d2D
Where d2 is the opposite side (half the diameter of the planet) and D is the adjacent side (distance from the observatory to the planet).
Step 5: Approximate for Small Angles
For small angles (which is the case here), we can use the approximation:
tan(θ2)≈θ2
Thus, we can rewrite the equation as:
θ2=d2D
Step 6: Solve for the Diameter d
Rearranging the equation gives:
d2=D⋅θ2
Multiplying both sides by 2:
d=D⋅θ
Conclusion
The approximate diameter of the planet is:
d≈D⋅θ
Final Answer
Thus, the approximate diameter of the planet is d=D⋅θ.
---
We have an observatory on Earth and a planet at a distance D. The planet's diametrically opposite extremes subtend an angle θ at the observatory. We can visualize this scenario as a triangle formed by the observatory and the two extremes of the planet.
Let:
- D = distance from the observatory to the planet
- d = diameter of the planet
- The angle subtended at the observatory is θ.
Since the diameter subtends the angle θ, each half of the diameter subtends an angle of θ2.
In the triangle formed (let's label the observatory point as O, one extreme of the planet as A, and the other extreme as C), we can use the tangent function:
tan(θ2)=oppositeadjacent=d2D
Where d2 is the opposite side (half the diameter of the planet) and D is the adjacent side (distance from the observatory to the planet).
For small angles (which is the case here), we can use the approximation:
tan(θ2)≈θ2
Thus, we can rewrite the equation as:
θ2=d2D
Rearranging the equation gives:
d2=D⋅θ2
Multiplying both sides by 2:
d=D⋅θ
The approximate diameter of the planet is:
d≈D⋅θ
Thus, the approximate diameter of the planet is d=D⋅θ.
Topper's Solved these Questions
- UNITS AND MEASUREMENTSBook:AAKASH INSTITUTE ENGLISHChapter:UNITS AND MEASUREMENTSExercise:ASSIGNMENT SECTION B Objective (One option is correct )
Explore 45 Videos
- UNITS AND MEASUREMENTSBook:AAKASH INSTITUTE ENGLISHChapter:UNITS AND MEASUREMENTSExercise:ASSIGNMENT SECTION C Objective (More than one option is correct )
Explore 10 Videos
- UNITS AND MEASUREMENTSBook:AAKASH INSTITUTE ENGLISHChapter:UNITS AND MEASUREMENTSExercise:Try Yourself
Explore 82 Videos
- THERMODYNAMICSBook:AAKASH INSTITUTE ENGLISHChapter:THERMODYNAMICSExercise:ASSIGNMENT (SECTION -D) (Assertion - Reason Type Questions)
Explore 10 Videos
- WAVE OPTICSBook:AAKASH INSTITUTE ENGLISHChapter:WAVE OPTICSExercise:Assignment (Section-J (Aakash Challengers question))
Explore 1 Video
Similar Questions
The moon's distance from the earth is 360000 km and its diameter subtends an angle of 31' at the eye of the observer. Find the diameter of the moon.
View SolutionThe moons distance from the earth is 360,000kms and its diameter subtends an angle of 31 at the eye of the observer. Find the diameter of the moon.
View SolutionThe moon's distance from the earth is 360000km and its diameter subtends an angle of 42' at the eye of the observer. The diameter of the moon in kilometers is
View SolutionAssuming the distance of the earth from the moon to be 38,400 km and the angle subtended by the moon at the eye of a person on the earth to be 31’, find the diameter of the moon.
View SolutionAssuming the distance of the earth from the moon to be 38,400 km and the angle subtended by the moon at the eye of a person on the earth to be 31, find the diameter of the moon.
View SolutionA pole has to be erected at a point on the boundary of a circular park of diameter 13 metres in such a way that the differences of its distances from two diametrically opposite fixed gates A and B on the boundary is 7 metres. Is it possible to do so? If yes, at what distances from the two gates should the pole be erected?
View SolutionA pole has to be erected at a point on the boundary of a circular park of diameter 13 metres in such a way that the difference of its distances from two diametrically opposite fixed gates A and B on the boundary is 7 metres. Is it the possible to do so? If yes, at what distances from the two gates should the pole be erected?
View SolutionAssuming the average distance of the earth from the sun to be 149700000 km and the angle subtended by the sun at the eye of a person on the earth to be 32', find the sun's diameter.
View Solution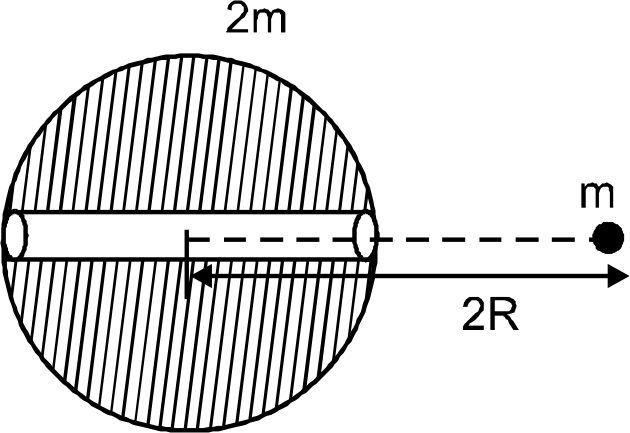
A solid spherical planet of mass 2m and radius 'R' has a very small tunnel along its diameter. A small cosmic particle of mass m is at a distance 2R from the centre of the planet as shown. Both are initially at rest, and due to gravitational attraction, both start moving toward each other. After some time, the cosmic particle passes through the centre of the planet. (Assume the planet and the cosmic particle are isolated from other planets)
View SolutionTaking the sum's distance as 1.4950×108 km and the angle subtended by the sum at a point O on earth as half a degree, find approximately the diameter of the sun.
View Solution