Frequency is the function of density (ρ) , length (a) and surface tension (T) . Then its value is
kρ1/2l3/2T−1/2
kρ3/2l3/2T−1/2
kρ1/2l3/2T−3/4
kT1/2ρ−1/2l−3/2
The correct Answer is:4
To find the relationship for frequency (f) as a function of density (ρ), length (a), and surface tension (T), we can use dimensional analysis. Here’s a step-by-step solution:
Step 1: Define the relationship
Assume that frequency (f) can be expressed as:
f=K⋅ρA⋅aB⋅TC
where K is a dimensionless constant, and A, B, and C are the powers we need to determine.
Step 2: Write down the dimensions
The dimensions of frequency (f) are:
(f)=T−1
Now, we will write the dimensions of each variable:
- Density (ρ):
(ρ)=ML3=ML−3
- Length (a):
(a)=L
- Surface tension (T):
(T)=FL=MLT−2L=MT−2
Step 3: Substitute dimensions into the equation
Substituting the dimensions into the equation gives:
T−1=(ML−3)A⋅(L)B⋅(MT−2)C
Step 4: Expand the right-hand side
This expands to:
T−1=MA+C⋅L−3A+B⋅T−2C
Step 5: Equate the dimensions
Now we equate the dimensions on both sides:
1. For mass (M):
A+C=0
2. For length (L):
−3A+B=0
3. For time (T):
−2C=−1
Step 6: Solve the equations
From the third equation:
−2C=−1⟹C=12
Substituting C into the first equation:
A+12=0⟹A=−12
Now substituting A into the second equation:
−3(−12)+B=0⟹32+B=0⟹B=−32
Step 7: Write the final expression
Now we can substitute the values of A, B, and C back into the original equation:
f=K⋅ρ−12⋅a−32⋅T12
Step 8: Simplify the expression
This can be rewritten as:
f=K⋅T12ρ12⋅a32
Conclusion
Thus, the frequency is given by:
f∝T12ρ12⋅a32
Assume that frequency (f) can be expressed as:
f=K⋅ρA⋅aB⋅TC
where K is a dimensionless constant, and A, B, and C are the powers we need to determine.
The dimensions of frequency (f) are:
(f)=T−1
- Density (ρ):
(ρ)=ML3=ML−3
- Length (a):
(a)=L
- Surface tension (T):
(T)=FL=MLT−2L=MT−2
Substituting the dimensions into the equation gives:
T−1=(ML−3)A⋅(L)B⋅(MT−2)C
This expands to:
T−1=MA+C⋅L−3A+B⋅T−2C
Now we equate the dimensions on both sides:
1. For mass (M):
A+C=0
2. For length (L):
−3A+B=0
3. For time (T):
−2C=−1
From the third equation:
−2C=−1⟹C=12
A+12=0⟹A=−12
−3(−12)+B=0⟹32+B=0⟹B=−32
Now we can substitute the values of A, B, and C back into the original equation:
f=K⋅ρ−12⋅a−32⋅T12
This can be rewritten as:
f=K⋅T12ρ12⋅a32
Thus, the frequency is given by:
f∝T12ρ12⋅a32
Topper's Solved these Questions
- UNITS AND MEASUREMENTSBook:AAKASH INSTITUTE ENGLISHChapter:UNITS AND MEASUREMENTSExercise:ASSIGNMENT SECTION C Objective (More than one option is correct )
Explore 10 Videos
- UNITS AND MEASUREMENTSBook:AAKASH INSTITUTE ENGLISHChapter:UNITS AND MEASUREMENTSExercise:ASSIGNMENT SECTION D (Linked Comprehension)
Explore 3 Videos
- UNITS AND MEASUREMENTSBook:AAKASH INSTITUTE ENGLISHChapter:UNITS AND MEASUREMENTSExercise:ASSIGNMENT SECTION A Objective (One option is correct )
Explore 50 Videos
- THERMODYNAMICSBook:AAKASH INSTITUTE ENGLISHChapter:THERMODYNAMICSExercise:ASSIGNMENT (SECTION -D) (Assertion - Reason Type Questions)
Explore 10 Videos
- WAVE OPTICSBook:AAKASH INSTITUTE ENGLISHChapter:WAVE OPTICSExercise:Assignment (Section-J (Aakash Challengers question))
Explore 1 Video
Similar Questions
The dependency of speed of water surface waves (capillary waves) on the density of water (ρ) their wavelength (λ) and surface tension (γ) is -
View SolutionOn heating a liquid, its surface tension
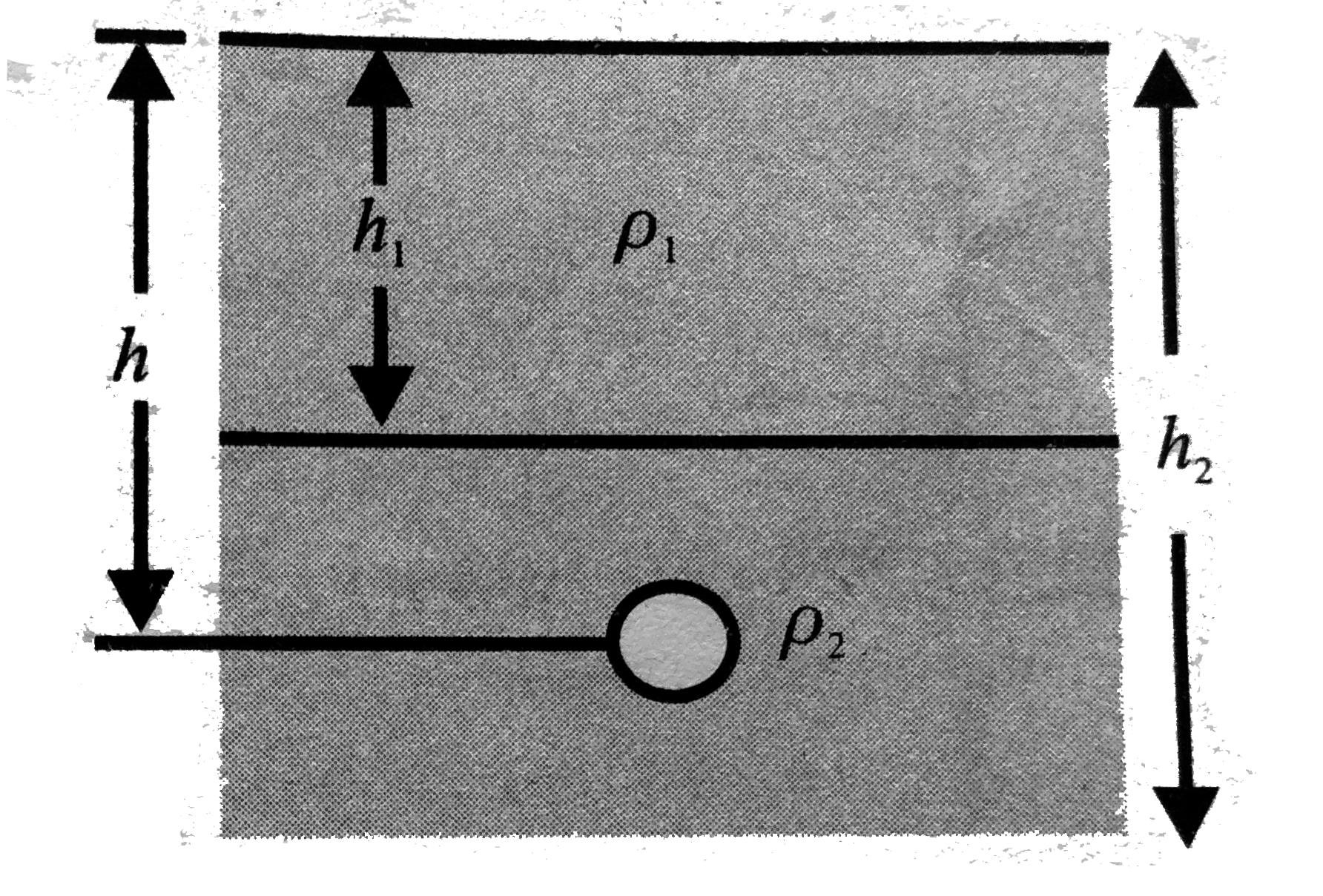
View SolutionCalculate the pressure inside a small air bubble of radus r situated at a depth h below the free surface of liquids of densities ρ1 and ρ2 and surface tennsions T1 and T2. The thickness of the first and second liquids are h1 and h2 respectively. Take atmosphere pressure =P0.
View SolutionThe time period of oscillation T of a small drop of liquid under surface tension depends upon the density ρ, the radius r and surface tension σ. Using dimensional analysis show that T∝√ρr3σ
View SolutionA sphere of density ρ is half submerged in a liquid of density σ and surface tension T. The sphere remains in equilibrium. Find radius of the sphere (assume the force due to surface tension acts tangentially to surface of sphere)
View SolutionWhat is the surface energy of a soap bubble of radus r and surface tension T?
View SolutionA spherical liquid drop is placed on a horizontal plane . A small distrubance cause the volume of the drop to oscillate . The time period oscillation (T) of the liquid drop depends on radius (r) of the drop , density (ρ) and surface tension tension (S) of the liquid. Which amount the following will be be a possible expression for T (where k is a dimensionless constant)?
View SolutionIt has been observed that velocity of ripple waves produced in water (ρ) , and surface tension (T) . Prove that V2∝T/λρ.
View SolutionA capillary tube with inner cross-section in the form of a square of side a is dipped vertically in a liquid of density ρ and surface tension ρ which wet the surface of capillary tube with angle of contact θ . The approximate height to which liquid will be raised in the tube is : (Neglect the effect of surface tension at the corners of capillary tube)
View SolutionA needle of length l and density ρ will float on a liquid of surface tension σ if its radius r is less than or equal to:
View Solution
