Let X and Y be the sets of all positive divisions of400 and 1000 respectively (including 1 and the number). Then is T, then is-
The correct Answer is:C
To solve the problem step by step, we need to find the sets of positive divisors for 400 and 1000, determine their intersection, and then calculate T4.
Step 1: Find the positive divisors of 400
To find the positive divisors of 400, we can start by determining its prime factorization.
1. Prime Factorization of 400:
400=24×52
2. Finding Divisors:
The formula for finding the number of divisors from the prime factorization pe11×pe22 is (e1+1)(e2+1).
- For 400=24×52:
Number of divisors=(4+1)(2+1)=5×3=15
3. List of Divisors:
The positive divisors of 400 are:
1,2,4,5,8,10,16,20,25,40,50,80,100,200,400
Step 2: Find the positive divisors of 1000
Next, we will find the positive divisors of 1000.
1. Prime Factorization of 1000:
1000=103=(2×5)3=23×53
2. Finding Divisors:
- For 1000=23×53:
Number of divisors=(3+1)(3+1)=4×4=16
3. List of Divisors:
The positive divisors of 1000 are:
1,2,4,5,8,10,20,25,40,50,100,125,200,250,500,1000
Step 3: Find the intersection of sets X and Y
Now, we will find the intersection of the two sets of divisors.
1. Divisors of 400 (Set X):
X={1,2,4,5,8,10,16,20,25,40,50,80,100,200,400}
2. Divisors of 1000 (Set Y):
Y={1,2,4,5,8,10,20,25,40,50,100,125,200,250,500,1000}
3. Finding Intersection X∩Y:
The common elements in both sets are:
X∩Y={1,2,4,5,8,10,20,25,40,50,100,200}
Step 4: Count the number of elements in the intersection
The number of elements in X∩Y is:
T=12
Step 5: Calculate T4
Now we will calculate T4:
T4=124=3
Final Answer
Thus, the final answer is:
T4=3
To find the positive divisors of 400, we can start by determining its prime factorization.
400=24×52
The formula for finding the number of divisors from the prime factorization pe11×pe22 is (e1+1)(e2+1).
- For 400=24×52:
Number of divisors=(4+1)(2+1)=5×3=15
The positive divisors of 400 are:
1,2,4,5,8,10,16,20,25,40,50,80,100,200,400
Next, we will find the positive divisors of 1000.
1000=103=(2×5)3=23×53
- For 1000=23×53:
Number of divisors=(3+1)(3+1)=4×4=16
The positive divisors of 1000 are:
1,2,4,5,8,10,20,25,40,50,100,125,200,250,500,1000
Now, we will find the intersection of the two sets of divisors.
X={1,2,4,5,8,10,16,20,25,40,50,80,100,200,400}
Y={1,2,4,5,8,10,20,25,40,50,100,125,200,250,500,1000}
The common elements in both sets are:
X∩Y={1,2,4,5,8,10,20,25,40,50,100,200}
The number of elements in X∩Y is:
T=12
Now we will calculate T4:
T4=124=3
Thus, the final answer is:
T4=3
Topper's Solved these Questions
- SETS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SETS EXERCISE - SECTION-H(Multiple True-False type Questions)
2 Videos
- SETS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SETS EXERCISE - SECTION-I(Aakash Challengers Questions)
4 Videos
- SETS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SETS EXERCISE - SECTION-F(Matrix-Match Type Questions)
1 Videos
- SEQUENCES AND SERIES BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - J) Aakash Challengers
11 Videos
- STATISTICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STATISTICSEXERCISE - Section-C Assertion-Reason
15 Videos
Similar Questions
Let X= the sets of all letters in the word "DELHI" and Y=the sets of all letters in the word "INDIA" Find X∪Y.
View SolutionIf x = 4y + 5 and y = kx + 4 are the lines of regression on X on Y and Y on X respectively, where k is a positive constant, prove that it can't exceed 14.
View SolutionLet X be the set of all positive such that f(x+y)=f(xy) for all x≥4,y≥4. If f(8)=9, then f(9) is equal to.
View SolutionThe number of the positive integral solutions (x,y,z) of the equation xyz = 24 is t, then the number of all possible factors of t is
View Solutionif X and Y are two sets , then X∩(Y∪X)'equals
View SolutionThe x and y coordinates of the particle at any time are x=5t−2t2andy=10t respectively, where x and y are in meters and t in seconds. The acceleration of the particle at t=2s is:
View SolutionThe x and y coordinates of the particle at any time are x=5t−2t2andy=10t respectively, where x and y are in meters and t in seconds. The acceleration of the particle at t=2s is:
View SolutionThe x and y coordinates if the particle at any time are x=5t−2t2 and t = 10t respectively, where x and y are in meters and t in second.The acceleation of the aprticle at t = 2 s is
View SolutionConsider radioactive decay of A to B with which further decays either to X or Y, λ1,λ2 and λ3 are decay constant for A to B decay, B to X decay and Bto Y decay respectively. At t=0, the number of nuclei of A,B,X and Y are N0,N0 zero and zero respectively.
N1,N2,N3 and N4 are the number of nuclei of A,B,X and Y at any instant t.
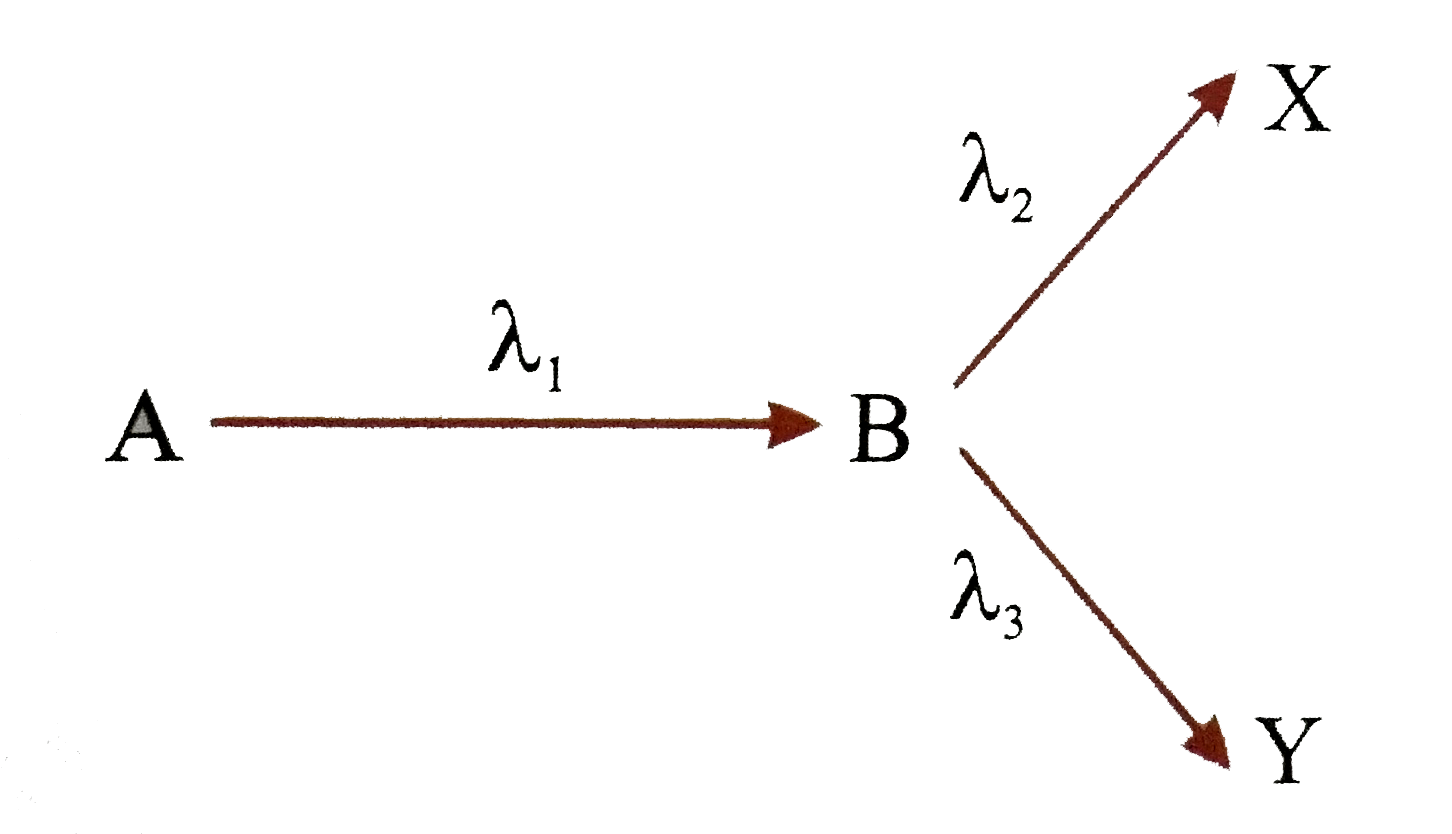
The net rate of accumulation of B at any instant is
View SolutionLet R be a relation on the set of all real numbers defined by xRy⇔|x−y|≤12 Then R is
View Solution
