A person's lungs might holds 6.0 L of air at body temperature (310K) and atmospheric pressure (101 kPa). Given that the air contains 21% oxygen, find the number of oxygen molecules in the lungs.
To find the number of oxygen molecules in a person's lungs, we can follow these steps:
Step 1: Convert the volume from liters to cubic meters
The volume of air in the lungs is given as 6.0 L. We need to convert this to cubic meters (m³) because the SI unit for volume is cubic meters.
V=6.0L=6.0×10−3m3
Step 2: Convert the pressure from kilopascals to pascals
The atmospheric pressure is given as 101 kPa. We need to convert this to pascals (Pa).
P=101kPa=101×103Pa=101000Pa
Step 3: Use the ideal gas law to find the number of molecules of air
The ideal gas law is given by the equation:
PV=NkBT
Where:
- P = pressure in pascals
- V = volume in cubic meters
- N = number of molecules
- kB = Boltzmann constant (1.38×10−23J/K)
- T = temperature in Kelvin
Rearranging the equation to solve for N:
N=PVkBT
Substituting the known values:
N=(101000Pa)(6.0×10−3m3)(1.38×10−23J/K)(310K)
Step 4: Calculate N
Now we can calculate N:
N=(101000)(6.0×10−3)(1.38×10−23)(310)
Calculating the numerator:
101000×6.0×10−3=606
Calculating the denominator:
(1.38×10−23)(310)=4.278×10−21
Now substituting back:
N=6064.278×10−21≈1.42×1023
Step 5: Calculate the number of oxygen molecules
Since the air contains 21% oxygen, we can find the number of oxygen molecules (NO2):
NO2=0.21×N
Substituting the value of N:
NO2=0.21×1.42×1023≈2.98×1022
Final Answer
The number of oxygen molecules in the lungs is approximately:
NO2≈2.98×1022molecules
---
The volume of air in the lungs is given as 6.0 L. We need to convert this to cubic meters (m³) because the SI unit for volume is cubic meters.
The atmospheric pressure is given as 101 kPa. We need to convert this to pascals (Pa).
The ideal gas law is given by the equation:
- P = pressure in pascals
- V = volume in cubic meters
- N = number of molecules
- kB = Boltzmann constant (1.38×10−23J/K)
- T = temperature in Kelvin
Now we can calculate N:
Since the air contains 21% oxygen, we can find the number of oxygen molecules (NO2):
The number of oxygen molecules in the lungs is approximately:
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Try Yourself43videos
KINETIC THEORY
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Assignment (Section-A) Objective type questions (One option is correct)49videos
KINETIC THEORY
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)15videos
GRAVITATION
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-D) (Assertion-Reason Type Questions)15videos
LAWS OF MOTION
Similar Questions
An amount n (in moles) of a monatomic gas at initial temperature T0 is enclosed in a cylindrical vessel fitted with a light piston. The surrounding air has a temperature Ts(>T0) . And the atmospheric pressure is Pa . Heat may be conducted between the surrounding and the gas through the bottom of the cylinder. The bottom has a surface area A, thickness x and thermal conductivity K. Assuming all change to be slow, find the distance moved by the piston in time t.
View SolutionThe figure given below shows three glass chambers that are connected by valves of negligible volume. At the outset of an experiment, the valves are closed and the chambers contain the gases as detailed in the diagram. All the chambers are at the temperature of 300K and external pressure of 1.0atm.
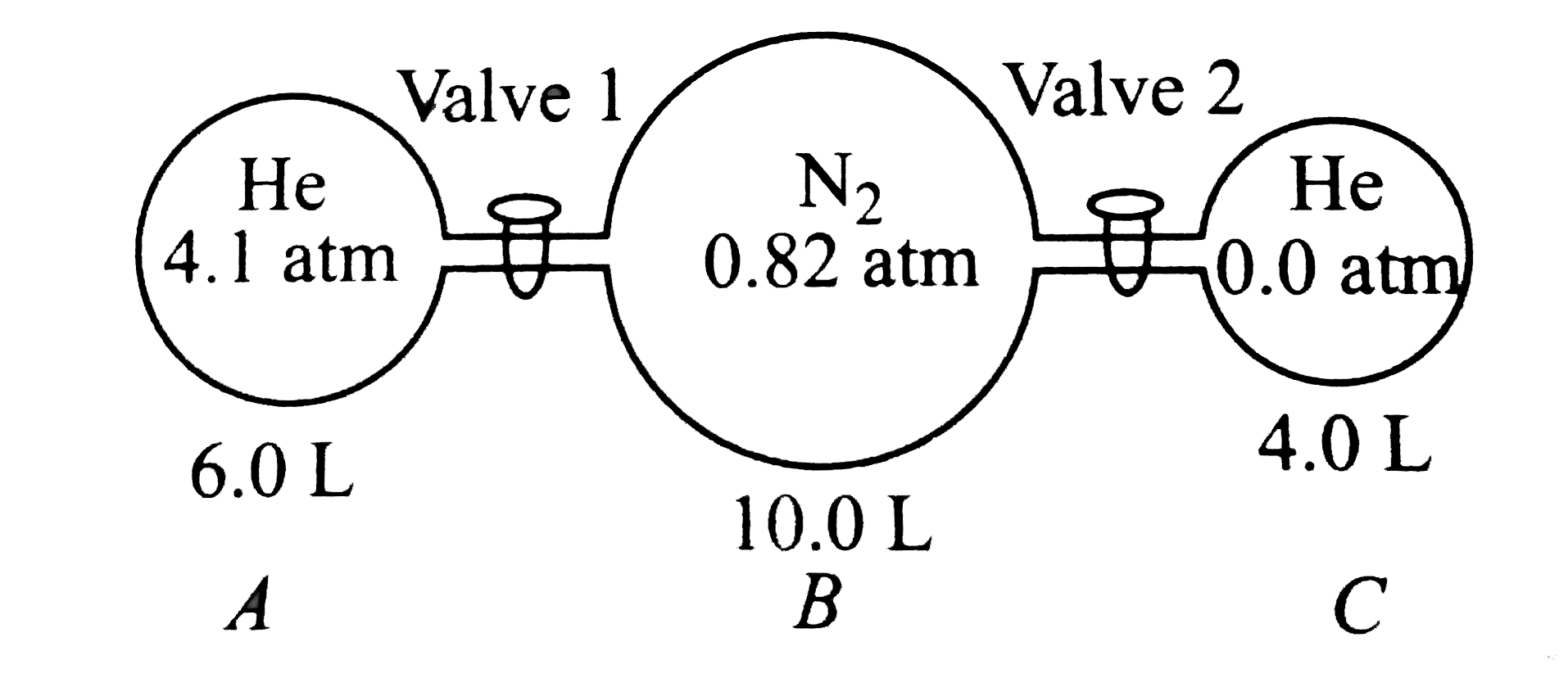
Which of the following represents the total kinetic energy of all the gas molecules after both valves are opened?
(R=0.082atmLK−1mol−1=8.314JK_1mol−1)View Solution
Knowledge Check
- Question 1 - Select One
Air contains 21% of oxygen by volume. The number of moles of O2 present in 5L of air at STP conditions
A2.23×10−1B4.68×10−4C4.68×10−2D0.0234
Air entering the lungs ends up in tiny sacs called alveoli.From the alveoli, the oxygen diffuses into the blood. The average radius of the alveoli is 0.0050 cm and the air inside contains 14 per cent oxygen. Assuming that the pressure in the alveoli is 1.0 atm and the temperature is 37∘C, calculate the number of oxygen molecules in one of the alveoli.
View SolutionA monatomic ideal gas is contained in a rigid container of volume V with walls of total inner surface area A, thickness x and thermal conductivity K. The gas is at an initial temperature t0 and pressure P0 . Find the pressure of the gas as a function of time if the temperature of the surrounding air is Ts . All temperature are in absolute scale.
View SolutionConsider a cubical vessel of edge a having a small hole in one of its walls. The total thermal resistance of the walls is r. At time t=0 , it contains air at atmospheric pressure pa and temperature T0 . The temperature of the surrounding air is Ta(>T0) . Find the amount of the gas (in moles) in the vessel at time t. Take Cv of air to be 5R/2.
View SolutionTwo identical soap bubbles each of radius r and of the same surface tension T combine to form a new soap bubble of radius R. The two bubbles contain air at the same temperature. If the atmospheric pressure is p0 then find the surface tension T of the soap solution in terms of p0, r and R. Assume process is isothermal.
View SolutionOxygen is filled in a closed metal jar of volume 1.0×10−3m3 at a pressure of 1.5×105Pa. and temperature 400K.The jar has a small leak in it. The atmospheric pressure is 1.0×105Pa and the atmospheric temperature is 300K. Find the mass of the gas that leaks out by time the pressure and the temperature inside the jar equlise with the surrounding.
View SolutionConsider
(i) C(s)+O2⇔CO2(g)KP2=78
(ii) 2C(s)+O2⇔2CO(g)KP2=12.5atm
As 100L of air (80%N2,20%O2 by volume) is pased over excess heated coke to establish these equilibrium the equilibrium mixture is found to measure 105L at constnat temperature & pressure (105atm). Assuming no other reaction, find the sum of partial pressure of COandCO2 in the final equilibrium mixture.View SolutionAn athelet takes 20 breaths per minute at room temperature. The air inhaled in each breath is 200mL which contains 20% oxygen by volume, while exhaled air contains 10% oxygen by volume. Assuming that all the oxygen consumed if used for converting glucose into CO2 and H2O(l) , how much glucose will be burnt in the body in one hour and what is the heat produced ? ( Room temperature −27∘C and enthalpy of combustion of glucose is −2822.5kJmol−1at0∘C)
View Solution

