In the given square each side is of 1m and resistance of wire is 1Ω/m and resistance between AE is 1Ω. A constant potential difference is applied across A and C. If potentials of B and E are same, then

(a) CEED=1√2 (b) CEED=1
(c ) CEED=2 (d) CEED=√2
Strategy : The potential of points B and E will be same , when this is a balanced bridge circuit.
RAERAB=RCERBC⇒(1+x)×12+x1=1−x1
1+x=2+x−2x−x2
x=−2±√4+42
=−2+2√22
⇒x=(√2−1)

Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Try Yourself
34 Videos
- CURRENT ELECTRICITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - ASSIGNMENT(SECTION-A(OBJECTIVE TYPE QUESTIONS))
69 Videos
- COMMUNICATION SYSTEMS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - COMMUNICATION SYSTEMS EXERCISE - ASSIGNMENT SECTION D (Assertion-Reason)
10 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
Similar Questions
ABCD is a square where each side is a uniform wire of resistance 1Ω. A point E lies on CD such that if a uniform wire of resistance 1Ω is connected across AE and constant potential difference is applied across A and C, then B and E are equipotential.

View Solution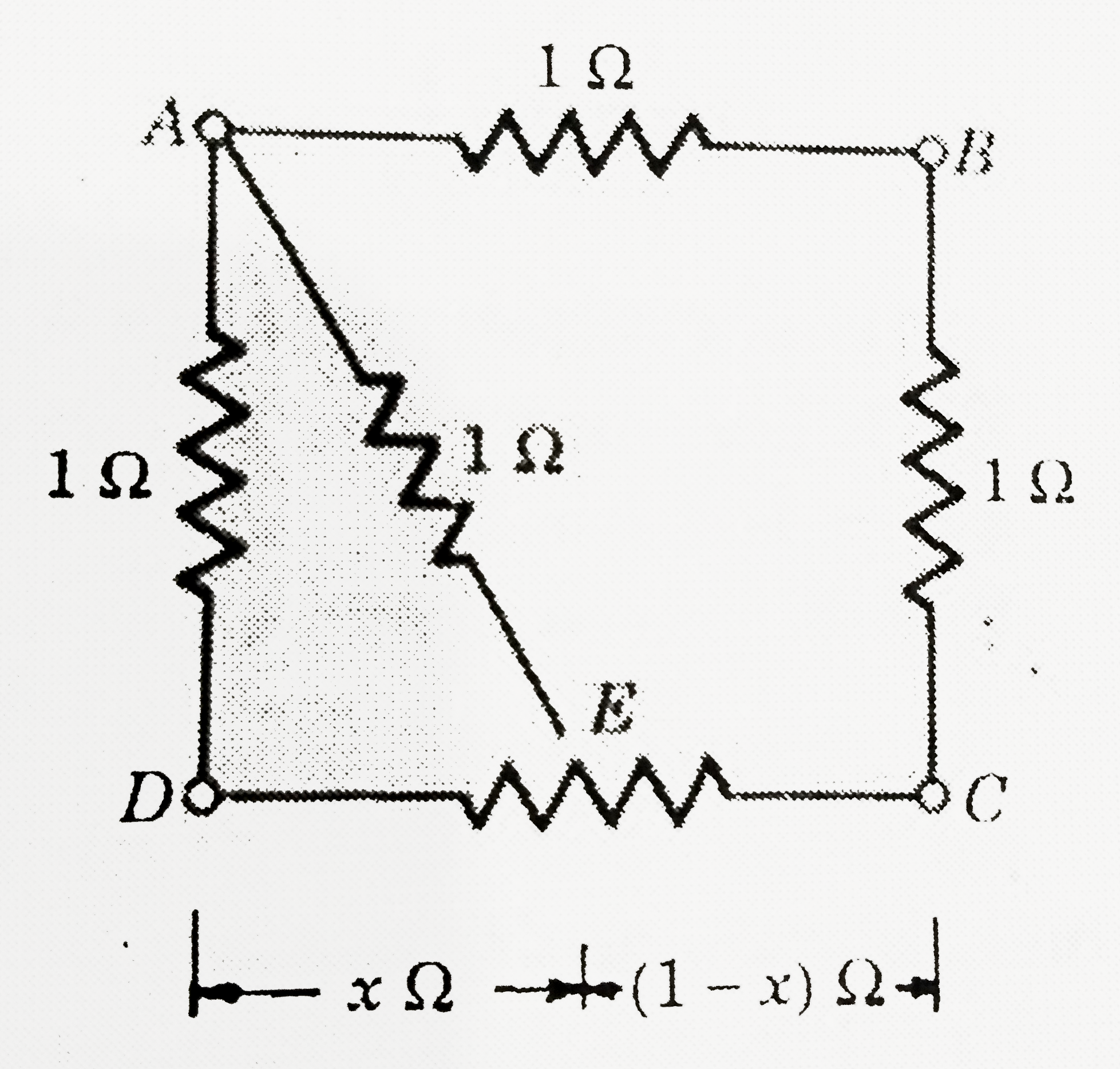
In figure. ABCD is a square where side is a uniform wire of resistance 1Ω. Find the a point E on CD such that if a uniform wire of resistance 1Ω is connected across AE and a constant potential differene is applied across A and C the points B and E are equipotential.
View Solution
Knowledge Check
ABCD is a square where each side is a uniform wire of resistance 1Ω. A point E lies on CD such that if a uniform wire of resistance 1Ω is connected across AE and constant potential difference is applied across A and C, then B and E are equipotential.
 ACEED=1BCEED=1√2CCEED=1√2DCEED=√2
ACEED=1BCEED=1√2CCEED=1√2DCEED=√2A wire has linear resistance ρ (inΩm−1). Find the resistance R between points A and B if the side of the big square is d.
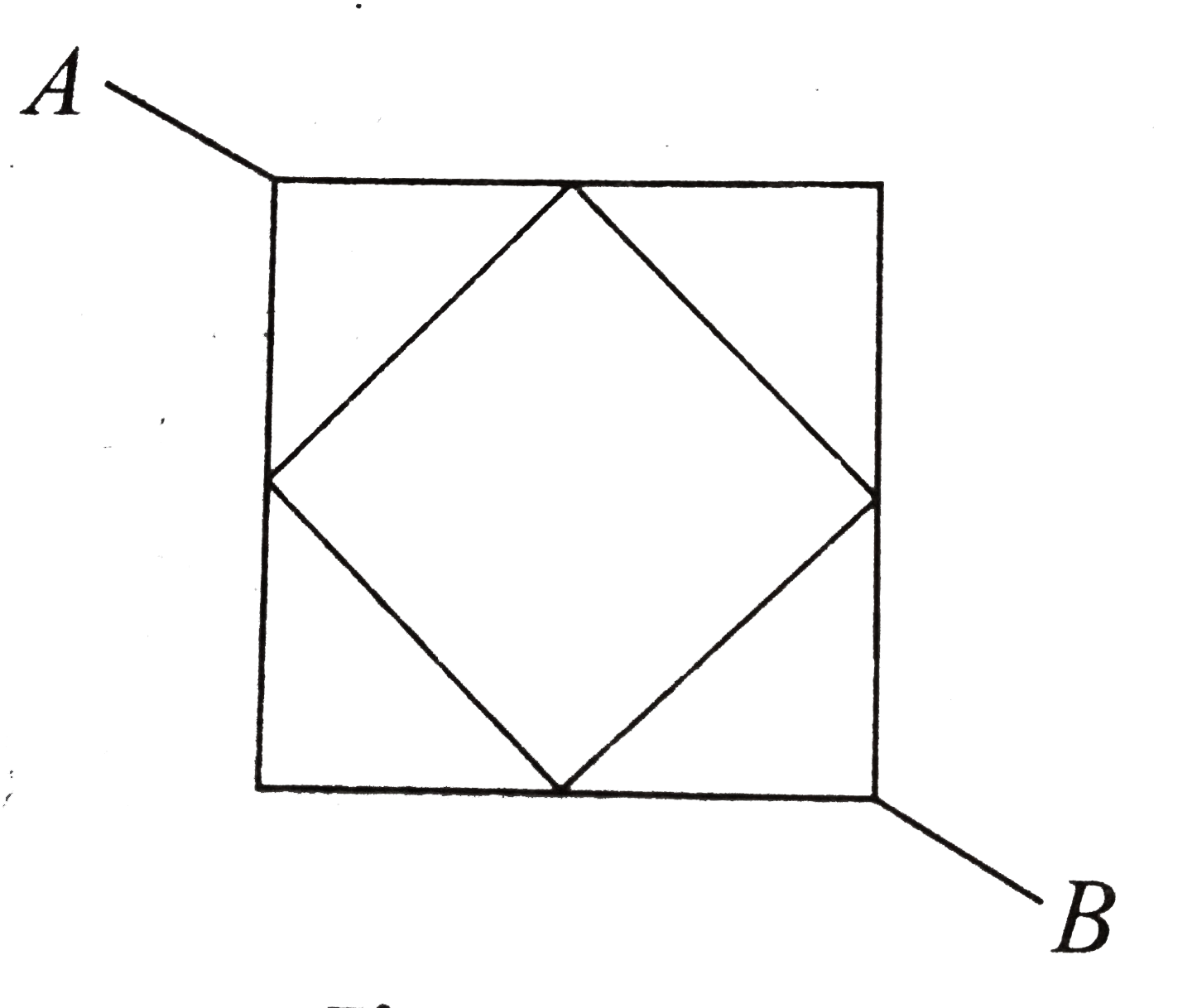 Aρd√2B(√2)ρdC2ρdDnone of these
Aρd√2B(√2)ρdC2ρdDnone of theseIn the circuit of figure, the current in 4Ω resistance is 1.2A , what is the potential difference between B and C?
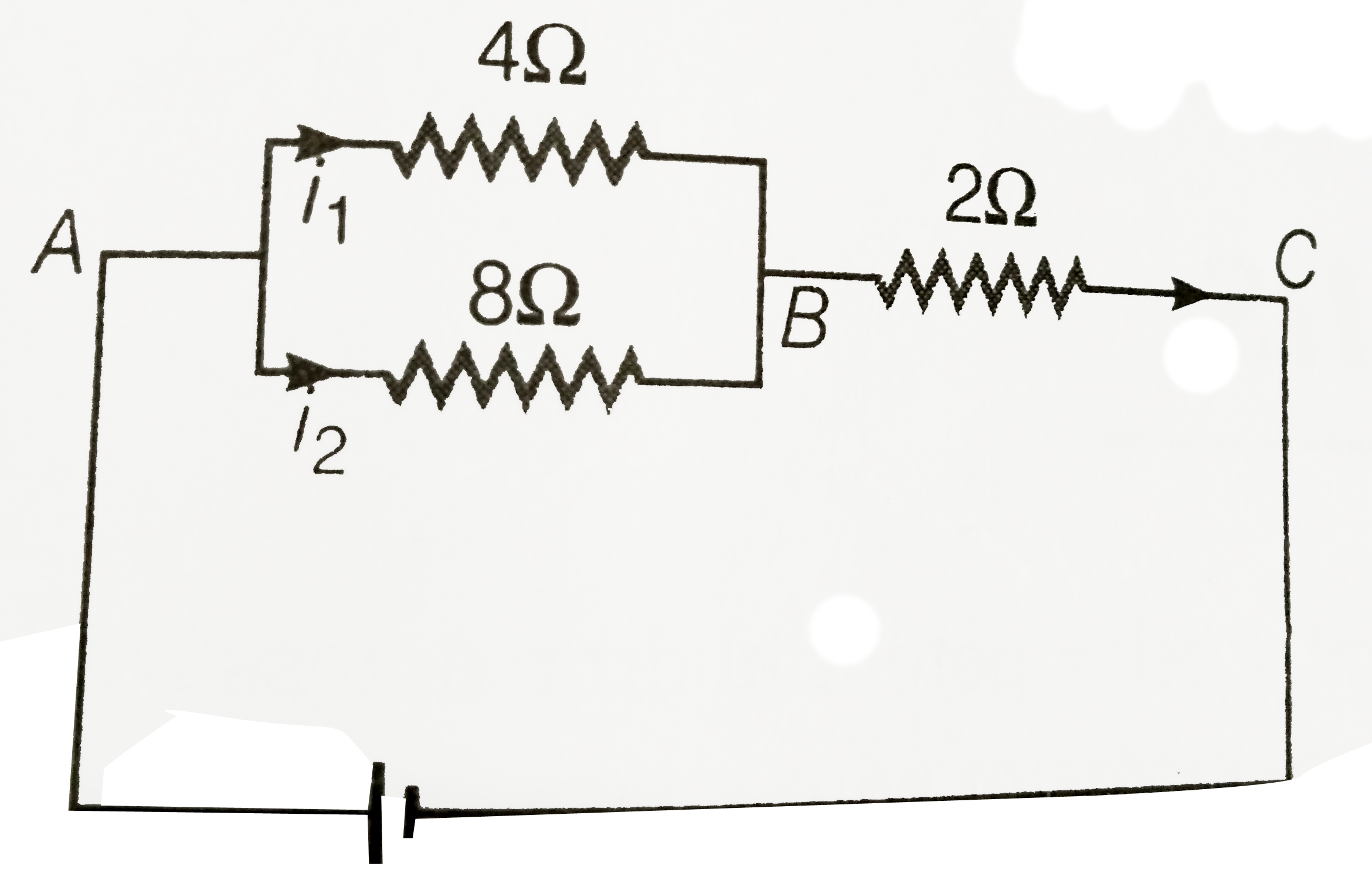 A3.6VB6.3VC1.8VD2.4V
A3.6VB6.3VC1.8VD2.4V
A wire has linear resistance ρ (inΩm−1). Find the resistance R between points A and B if the side of the big square is d.

View SolutionIn the circuit of figure, the current in 4Ω resistance is 1.2A , what is the potential difference between B and C?

View Solution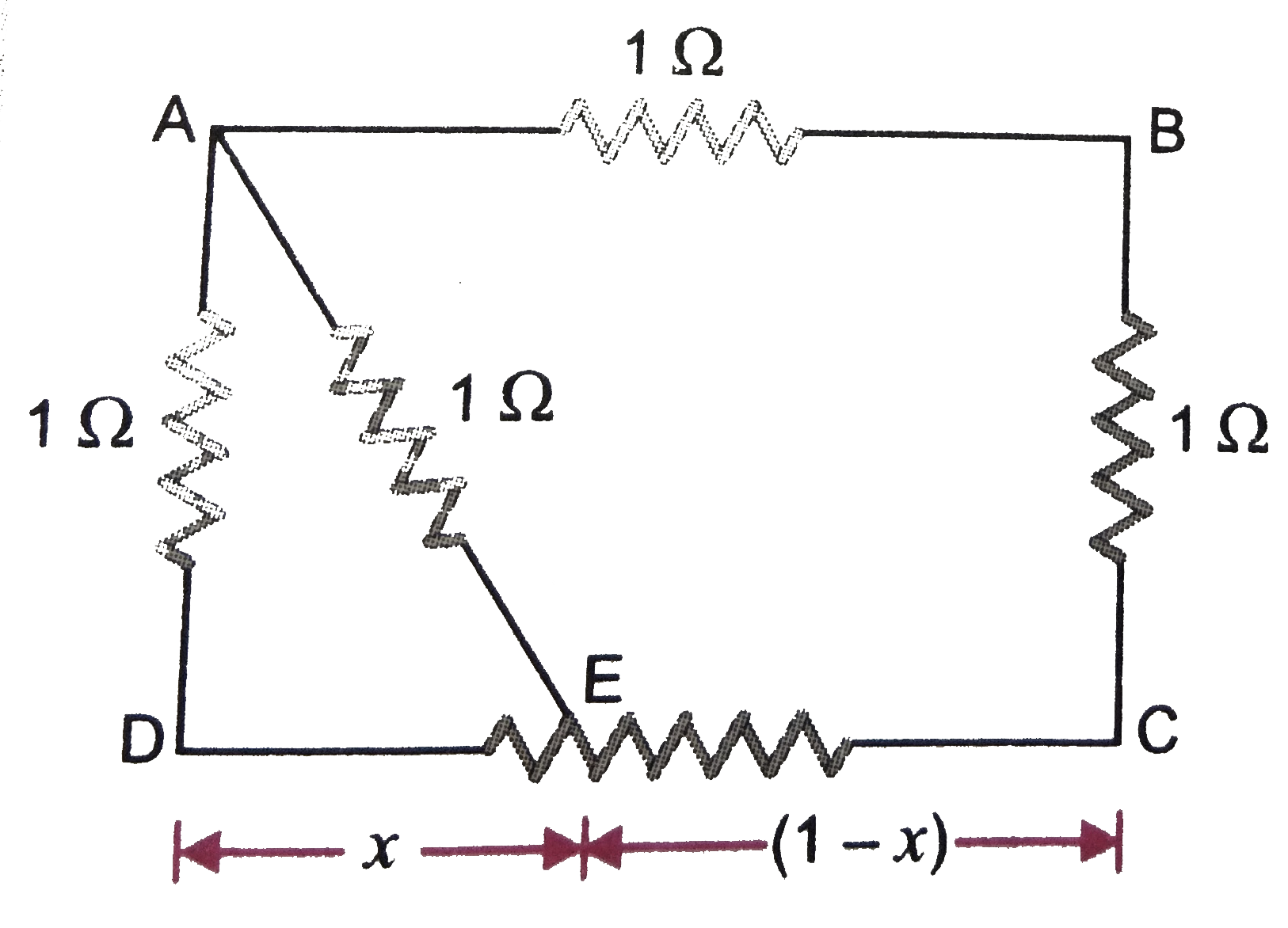
ABCD is a square where each side is a uniform wire of resistance 1Ω. Find a point E on CD such that if a uniform wire of resistance 1Ω is connected across AE and a constant potential is applied across A and C, the points B and E will be equipotential.
View SolutionIn the given circuit diagram, potential difference across 2Ω resistance is
View SolutionA wire of length 1.6m has a resistance 8Ω is connected to a battery of 2 volts and internal resistance 2Ω. What is the potential gradient ?
View Solution
