When a body is rolling without slipping on a rough horizontal surface, the work done by friction is :
Always zero
May be zero
Always positive
Always negative
The correct Answer is:A
To solve the problem of determining the work done by friction when a body is rolling without slipping on a rough horizontal surface, we can follow these steps:
1. Understanding Rolling Without Slipping:
- When a body rolls without slipping, there is a specific relationship between the linear velocity of the center of mass (v) and the angular velocity (ω) of the body. This relationship is given by the equation:
v=r⋅ω
where r is the radius of the rolling body.
2. Identifying the Role of Friction:
- In rolling motion, friction is necessary to prevent slipping. It provides the torque needed for the body to rotate. However, it does not do work in this scenario.
3. Analyzing the Point of Contact:
- At the point of contact between the rolling body and the surface, there is no relative motion. This means that the point of contact does not move relative to the surface while the body rolls.
4. Calculating Work Done by Friction:
- The work done by a force is defined as:
Work=Force×Displacement
- Since the point of contact does not move (displacement is zero), the work done by the friction force can be expressed as:
Work=f×0=0
where f is the friction force.
5. Conclusion:
- Therefore, the work done by friction when a body is rolling without slipping on a rough horizontal surface is zero.
Final Answer:
The work done by friction is zero.
- When a body rolls without slipping, there is a specific relationship between the linear velocity of the center of mass (v) and the angular velocity (ω) of the body. This relationship is given by the equation:
v=r⋅ω
where r is the radius of the rolling body.
- In rolling motion, friction is necessary to prevent slipping. It provides the torque needed for the body to rotate. However, it does not do work in this scenario.
- At the point of contact between the rolling body and the surface, there is no relative motion. This means that the point of contact does not move relative to the surface while the body rolls.
- The work done by a force is defined as:
Work=Force×Displacement
- Since the point of contact does not move (displacement is zero), the work done by the friction force can be expressed as:
Work=f×0=0
where f is the friction force.
- Therefore, the work done by friction when a body is rolling without slipping on a rough horizontal surface is zero.
The work done by friction is zero.
Similar Questions
Which of the following statement (s) is / are correct for a spherical body rolling without slipping on a rough horizontal ground at rest?
View Solution
A ring of radius R rolls without slipping on a rough horizontal surface with a constant velocity. The radius of curvature of the path followed by any particle of the ring at the highest point of its path will be :
View Solution
Knowledge Check
A body is rolling without slipping on a horizontal plane. If the rotational energy of the body is 40% of the total kinetic energy, then the body might be
AcylinderBhollow sphereCsolid cylinderDring
A 1 Kg solid sphere rolls without slipping on a rough horizontal suface as shown in figure. Select incorrect alternative
View SolutionA body is rolling without slipping on a horizontal plane. The rotational energy of the body is 40%of the total kinetic energy. Identify the body.
View SolutionA body is rolling without slipping on a horizontal plane. The rotational energy of the body is 40% of the total kinetic energy. Identify the body
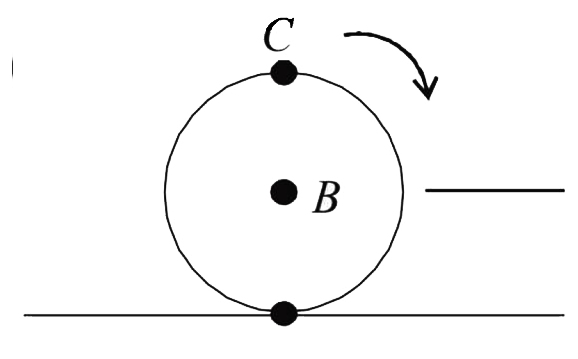
View SolutionA sphere is rolling without slipping on a fixed horizontal plane surface. In the figure, A is the point of contact, B is the centre of the sphere and C is its topmost point. Then
View SolutionA sphere of mass m is rolling without sliding along positive x-axis on a rough horizontal surface of coefficient of friction μ. It elastically collides with a wall and then returns. The correct statement of frictional force (f) acting on the sphere is
View Solution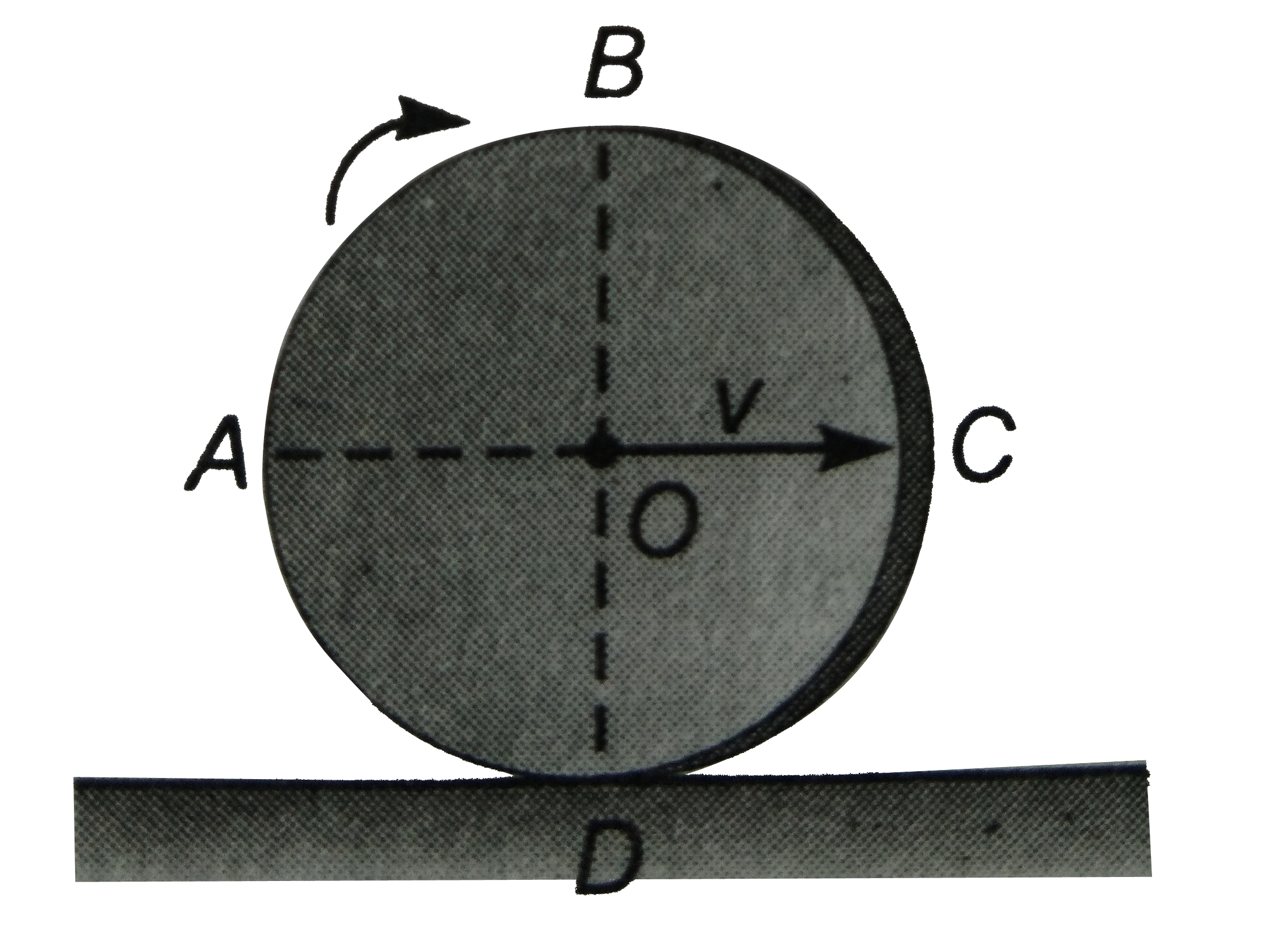
A uniform circular ring rolls without slipping on a horizontal surface. At any instant, its position is as shown in the figure. Then
View SolutionWhen a rolling body enters onto a smooth horizontal surface, it will
View Solution
