A heavy solid sphere is thrown on a horizontal rough surface with initial velocity u without rolling. What will be its speed, when it starts pure rolling motion ?
3u5
2u5
5u7
2u7
The correct Answer is:C
To find the speed of a heavy solid sphere when it starts pure rolling motion after being thrown on a rough horizontal surface with an initial velocity u, we can follow these steps:
1. Identify Initial Conditions:
- The sphere is thrown with an initial velocity u and is not rolling initially.
2. Understand the Forces Acting on the Sphere:
- When the sphere slides on the rough surface, friction acts in the opposite direction to the motion. This frictional force will slow down the linear motion of the sphere and will also cause it to start rotating.
3. Define Linear and Angular Motion:
- Let v be the linear velocity of the sphere at the moment it starts pure rolling.
- Let ω be the angular velocity of the sphere at that moment.
4. Use the Relationship Between Linear and Angular Velocity:
- For pure rolling motion, the relationship between linear velocity v and angular velocity ω is given by:
v=rω
where r is the radius of the sphere.
5. Apply Conservation of Angular Momentum:
- Since the net torque about the point of contact is zero when the sphere starts rolling, we can apply the conservation of angular momentum.
- The initial angular momentum Li about the point of contact (point A) is:
Li=Mur
- The final angular momentum Lf about point A when it starts rolling is:
Lf=IAω
where IA is the moment of inertia about point A.
6. Calculate the Moment of Inertia:
- The moment of inertia I of a solid sphere about its center is:
I=25Mr2
- Using the parallel axis theorem, the moment of inertia about point A is:
IA=I+Mr2=25Mr2+Mr2=75Mr2
7. Set Up the Equation from Conservation of Angular Momentum:
- Equating the initial and final angular momentum:
Mur=75Mr2ω
- Substitute ω with vr:
Mur=75Mr2(vr)
8. Simplify the Equation:
- Cancel M and r (assuming r≠0):
u=75v
9. Solve for v:
- Rearranging gives:
v=57u
Final Answer:
The speed of the sphere when it starts pure rolling motion is:
v=57u
- The sphere is thrown with an initial velocity u and is not rolling initially.
- When the sphere slides on the rough surface, friction acts in the opposite direction to the motion. This frictional force will slow down the linear motion of the sphere and will also cause it to start rotating.
- Let v be the linear velocity of the sphere at the moment it starts pure rolling.
- Let ω be the angular velocity of the sphere at that moment.
- For pure rolling motion, the relationship between linear velocity v and angular velocity ω is given by:
v=rω
where r is the radius of the sphere.
- Since the net torque about the point of contact is zero when the sphere starts rolling, we can apply the conservation of angular momentum.
- The initial angular momentum Li about the point of contact (point A) is:
Li=Mur
- The final angular momentum Lf about point A when it starts rolling is:
Lf=IAω
where IA is the moment of inertia about point A.
- The moment of inertia I of a solid sphere about its center is:
I=25Mr2
- Using the parallel axis theorem, the moment of inertia about point A is:
IA=I+Mr2=25Mr2+Mr2=75Mr2
- Equating the initial and final angular momentum:
Mur=75Mr2ω
- Substitute ω with vr:
Mur=75Mr2(vr)
- Cancel M and r (assuming r≠0):
u=75v
- Rearranging gives:
v=57u
The speed of the sphere when it starts pure rolling motion is:
v=57u
Similar Questions
A solid sphere is thrown on a horizontal rough surface with initial velocity of centre of mass V0 without rolling. Velocity of its centre of mass when its starts pure rolling is
View SolutionA uniform ring of radius R is given a back spin of angular velocity V0/2R and thrown on a horizontal rough surface with velocity of centre to be V0. The velocity of the centre of the ring when it starts pure rolling will be
View SolutionA uniform disc of mass m and radius R is thrown on horizontal lawn in such a way that it initially slides with speed V0 without rolling. The distance travelled by the disc till it starts pure rolling is (Coefficient friction between the contact is 0.5)
View SolutionA solid sphere of radius 2.45m is rotating with an angular speed of 10rad/s. When this rotating sphere is placed on a rough horizontal surface then after sometime it starts pure rolling. Find the linear speed of the sphere after it starts pure rolling.
View SolutionA solid sphere is in pure rolling motion on an inclined surface having inclination θ
View SolutionA solid sphere of radius r is rolling on a horizontal surface. The ratio between the rotational kinetic energy and total energy.
View SolutionA sphere rolling on a horizontal rough surface Collides elastically with a smooth vertical wall, as shown in Fig. State which of the following statements is true or false.
 a. After collision, the velocity of the centre of mass gets reversed.
a. After collision, the velocity of the centre of mass gets reversed.
b. Angular momentum of the sphere about the point of contact with the wall is conserved.
c. Angular momentum of the sphere about a stationary point on the horizontal surface is conserved.
d. Just after collision the point of contact with the horizontal surface is moving towards the wall.
e. After collision the friction force acts on the sphere such that it decreases the linear speed and increases the angular speed.
f. Finally, when the sphere starts rolling, it is moving away from the wall.
View SolutionA uniform solid sphere of radius R, rolling without sliding on a horizontal surface with an angular velocity ω0, meets a rough inclined plane of inclination θ=60∘. The sphere starts pure rolling up the plane with an angular velocity ω Find the value of ω.
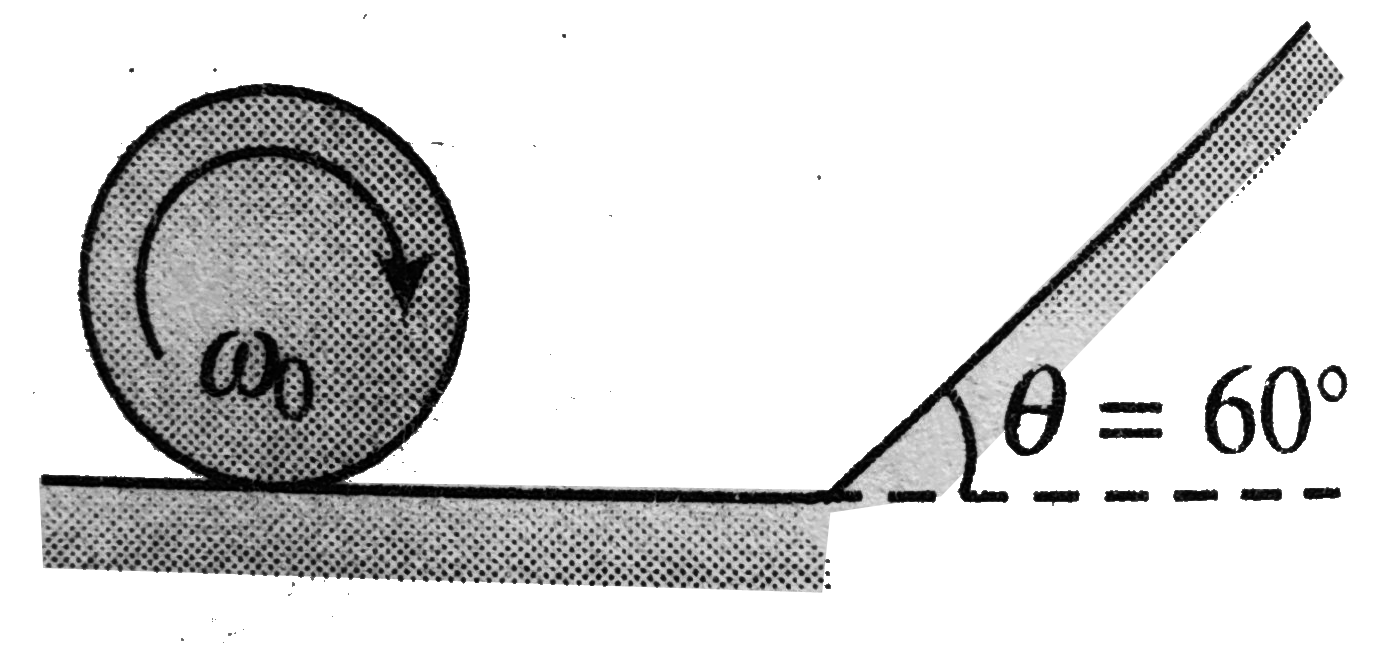
View SolutionA solid sphere rolls on horizontal surface without slipping. What is the ratio of its rotational to translation kinetic energy.
View SolutionA uniform sphere of radius R is palced on a rough horizontal surface and given a linear velocity v0 and an angular velocity ω0 as shown . If the angular velocity and the linear velocity both become zero simultaneously, then
View Solution
