A solid spherical ball rolls on a table. Ratio of its rotational kinetic energy to total kinetic energy is
12
16
710
27
The correct Answer is:D
Step by step video & image solution for A solid spherical ball rolls on a table. Ratio of its rotational kinetic energy to total kinetic energy is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
A circular disc rolls down an inclined plane . The ratio of rotational kinetic energy to total kinetic energy is
View SolutionThe moment of inertia of a solid cylinder about its axis is given by (1/2)MR2. If this cylinder rolls without slipping the ratio of its rotational kinetic energy to its translational kinetic energy is -
View SolutionA uniform thin spherical shell of mass m and radius R rolls down without slipping over an inclined plane of angle θ with the horizontal. The ratio of rotational kinetic energy to the total kinetic energy of the shell will be
View SolutionA solid sphere is rolling without slipping on a horizontal plane. The ratio of its rotational kinetic energy and translational kinetic energy is
View SolutionA hollow sphere is rolling without slipping on a rough surface. The ratio of translational kinetic energy to rotational kinetic energy is
View SolutionIf a sphere is rolling, the ratio of the translation energy to total kinetic energy is given by
View SolutionA ring is rolling without slipping. The ratio of translational kinetic energy to rotational kinetic energy is
View SolutionPortion AB of the wedge shown in figure is rough and BC is smooth. A solid cylinder rolld without slipping from A to B. Find the ratio of translational kinetic energy to rotational kinetic energy, when the cylinder reaches point C.
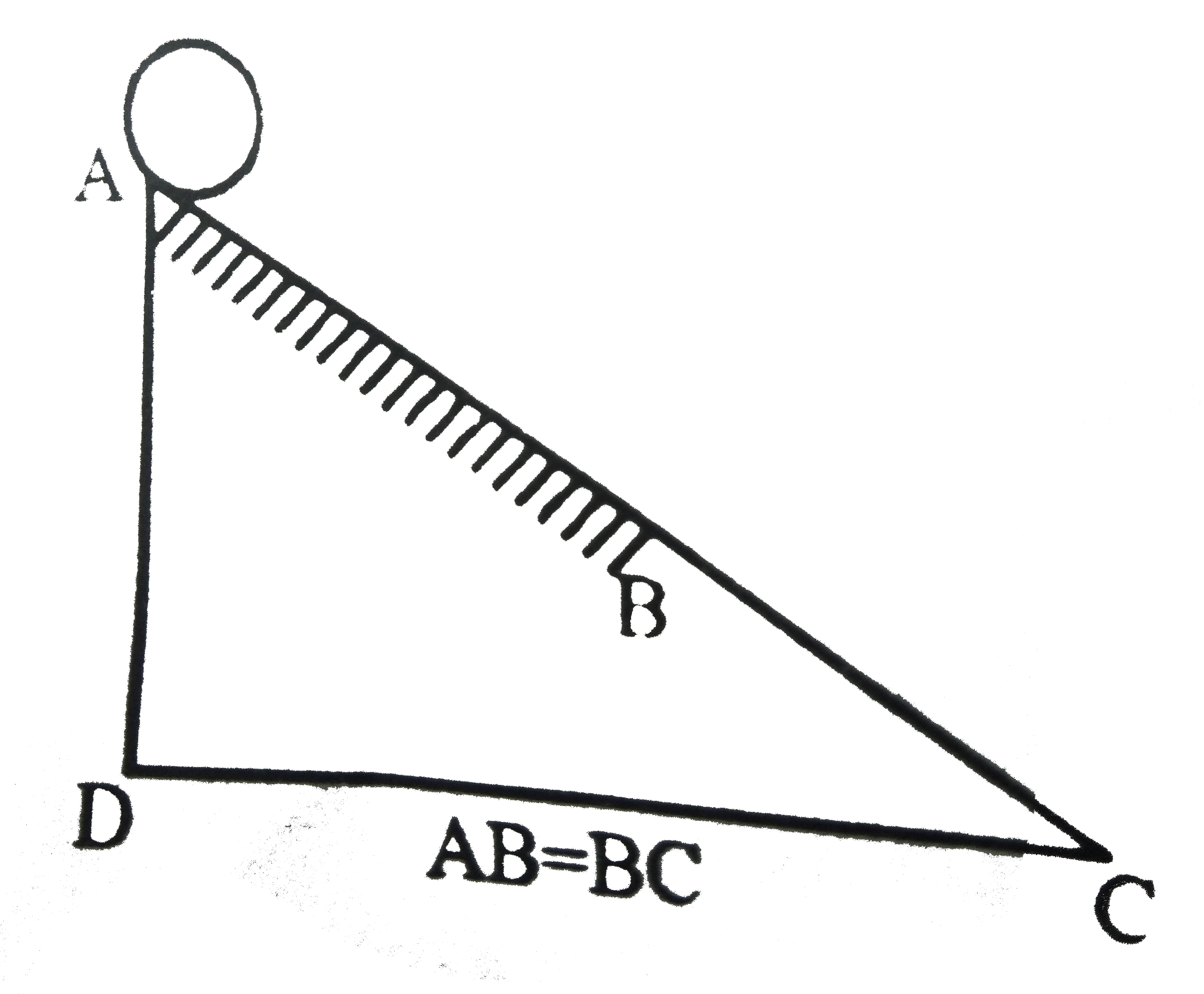 .
.
View SolutionA disc is rolling without slipping. The ratio of its rotational kinetic energy and translational kinetic energy would be -
View SolutionA small steel sphere of mass m and radius r rolls without slipping on the fiictionless surface of a large hemisphere of radius R{R>>r) whose axis of symmetry is vertical. It starts at the top from the rest,
(a) What is the kinetic energy at the bottom ?
(b) What fraction is the rotational kinetic energy of the total kinetic energy ?
(c) What fraction is the rotational kinetic energy of the total kinetic energy?
(d) What fraction is the translational kinetic energy of the total kinetic energy?
View Solution
