A : Two rings of equal mass and radius made of different materials, will have same moment of inertia.
R : Moment of inertia depends on mass as well as distribution of mass in the object.
If both Assertion & Reason are true and the reason is the correct explanation of the assertion,
If both Assertion & Reason are true but the reason is not the correct explanation of the assertion,
If Assertion is true statement but Reason is false,
If both Assertion and Reason are false statements,
The correct Answer is:A
To solve the assertion and reason question, we will analyze both statements step by step.
Step 1: Understanding the Assertion
The assertion states that "Two rings of equal mass and radius made of different materials will have the same moment of inertia."
- Moment of Inertia Formula: The moment of inertia (I) for a ring about its central axis is given by the formula:
I=mr2
where m is the mass of the ring and r is the radius.
- Since both rings have the same mass (M) and the same radius (R), we can substitute these values into the formula:
IA=MR2(for ring A)
IB=MR2(for ring B)
- This shows that both rings have the same moment of inertia regardless of the material they are made from.
Conclusion for Step 1:
The assertion is true.
Step 2: Understanding the Reason
The reason states that "Moment of inertia depends on mass as well as distribution of mass in the object."
- Distribution of Mass: The moment of inertia does not only depend on the total mass but also on how that mass is distributed relative to the axis of rotation.
- For the rings in question, even though they are made of different materials, their mass distribution (which is uniform and at a constant radius from the axis) is the same. Therefore, the moment of inertia is determined solely by the mass and the radius in this case.
Conclusion for Step 2:
The reason is also true and correctly explains the assertion.
Final Conclusion:
Both the assertion and the reason are true, and the reason correctly explains the assertion. Therefore, the correct answer is option A.
---
The assertion states that "Two rings of equal mass and radius made of different materials will have the same moment of inertia."
I=mr2
where m is the mass of the ring and r is the radius.
IA=MR2(for ring A)
IB=MR2(for ring B)
- This shows that both rings have the same moment of inertia regardless of the material they are made from.
The assertion is true.
The reason states that "Moment of inertia depends on mass as well as distribution of mass in the object."
The reason is also true and correctly explains the assertion.
Both the assertion and the reason are true, and the reason correctly explains the assertion. Therefore, the correct answer is option A.
Similar Questions
(A) : Two circular discs of equal masses and thickness made of different material, will have same moment of inertia about their central axes of rotation.
(R ) : Moment of inertia depends upon the distribution of mass in the body.
View SolutionThe moment of inertia of a ring of mass M and radius R about PQ axis will be

View Solution
Knowledge Check
The moment of inertia of a hoop of radius R and mass M, about any tangent
A[3mR2]/2B[mR2]/2CMR2D[MR2]/4
(A) : Moment of inertia plays the same role in rotational motion, as mass plays in linear motion.
(R ) : Moment of inertia depends only on mass of the body.
View Solution(A) : Moment of inertia of a particle changes with axis of rotation.
(R ) : Moment of inertia depends on mass and distance of the particle from the axis of rotation.
View SolutionThe moment of inertia of a cube of mass m and side a about one of its edges is equal to
View SolutionTwo rings having same moment of inertia have their radii in the ratio 1 : 4. Their masses will be in the ratio
View SolutionFour rings each of mass M and radius R are arranged as shown in the figure. The moment of inertia of the system about YY' will be
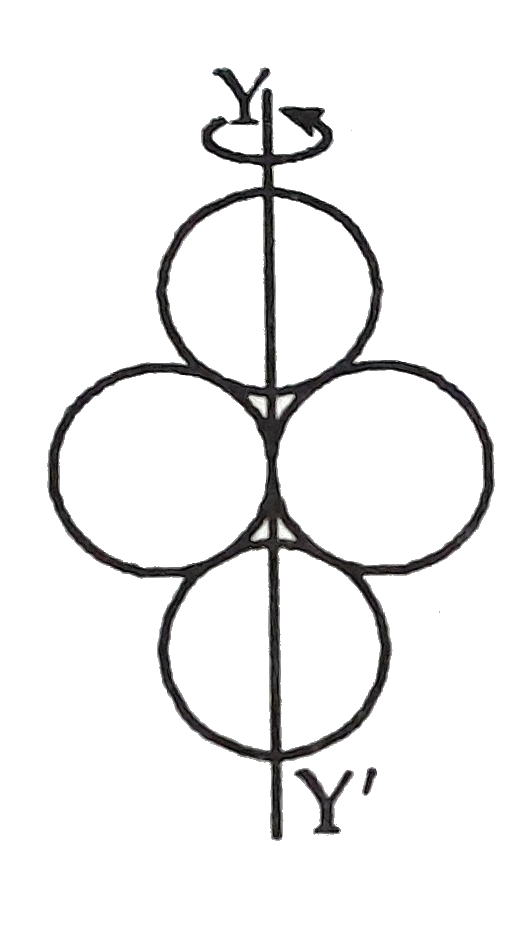
View SolutionTwo rings of radius R and nR made of same material have the ratio of moment of inertia about an axis passing through center is 1:8. The value of n is
View SolutionMoment of inertia of a circular wire of mass M and radius R about its diameter is
View Solution
