For an ideal gas the fractional change in its volume per degree rise in temperature at constant pressure is equal to [T is absolute temperature of gas]
T0
T
T−1
T2
The correct Answer is:C
To solve the problem, we need to determine the fractional change in volume per degree rise in temperature for an ideal gas at constant pressure. We will use the ideal gas law and calculus to derive the required relationship.
1. Start with the Ideal Gas Law:
The ideal gas law is given by the equation:
PV=nRT
where P is the pressure, V is the volume, n is the number of moles, R is the gas constant, and T is the absolute temperature.
2. Differentiate the Equation:
Since we are considering a process at constant pressure, we can differentiate both sides of the ideal gas law with respect to temperature T:
PdVdT=nR
3. Rearranging the Equation:
Rearranging the differentiated equation gives us:
dVdT=nRP
4. Expressing Fractional Change in Volume:
The fractional change in volume dVV can be expressed as:
dVV=dVdT⋅dTV
5. Substituting for dVdT:
From the previous step, we can substitute dVdT:
dVV=nRP⋅dTV
6. Using the Ideal Gas Law to Substitute for V:
From the ideal gas law, we can express V as:
V=nRTP
Substituting this into our equation gives:
dVV=nRP⋅dTnRTP=dTT
7. Finding the Fractional Change in Volume per Degree Rise in Temperature:
To find the fractional change in volume per degree rise in temperature, we can write:
dVV÷dT=1T
8. Final Result:
Thus, we find that the fractional change in volume per degree rise in temperature at constant pressure is:
dVV÷dT=T−1
Conclusion:
The answer is T−1, which corresponds to option 3.
The ideal gas law is given by the equation:
PV=nRT
where P is the pressure, V is the volume, n is the number of moles, R is the gas constant, and T is the absolute temperature.
Since we are considering a process at constant pressure, we can differentiate both sides of the ideal gas law with respect to temperature T:
PdVdT=nR
Rearranging the differentiated equation gives us:
dVdT=nRP
The fractional change in volume dVV can be expressed as:
dVV=dVdT⋅dTV
From the previous step, we can substitute dVdT:
dVV=nRP⋅dTV
From the ideal gas law, we can express V as:
V=nRTP
Substituting this into our equation gives:
dVV=nRP⋅dTnRTP=dTT
To find the fractional change in volume per degree rise in temperature, we can write:
dVV÷dT=1T
Thus, we find that the fractional change in volume per degree rise in temperature at constant pressure is:
dVV÷dT=T−1
The answer is T−1, which corresponds to option 3.
Topper's Solved these Questions
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - B Objective Type Questions
30 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - C Previous Years Questions
21 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE
10 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
15 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-D) (Assertion-Reason Type Questions)
15 Videos
Similar Questions
Knowledge Check
For an ideal gas, number of moles per litre in terms of its pressure P, gas constant R and temperature T is
APT / RBP / RTCPRTDRT / PWhat is the effect on the pressure of a gas if its temperature is increased at constant volume ?
AThe pressure of the gas increases.BThe pressure of the gas decreases .CThe pressure of the gas remains same .DThe pressure of the gas becomes half.
At constant pressure, the ratio of increases in volume of an ideal gas per degree rise in kelvin temperature to its volume is
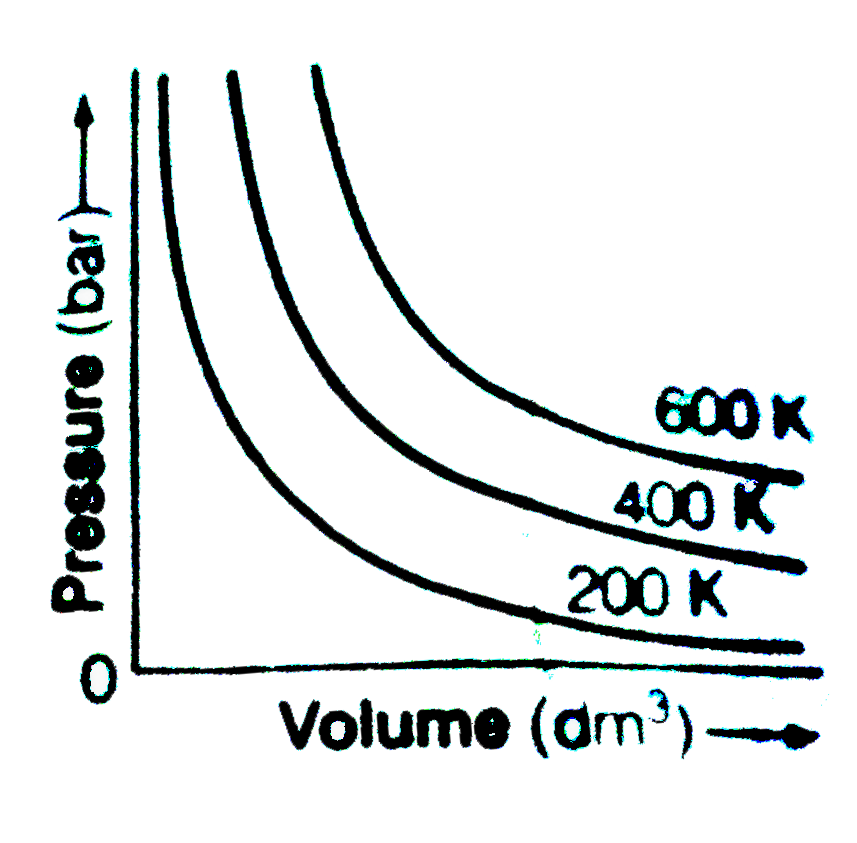
View SolutionThe variation of pressure with volume of the gas at different temperatures can be graphically represented as shown in figure. On the basis of this graph answer the following question.
(i) How will the volume of a gas change if its pressure is increased at constant temperature ?
(ii) At a constant pressure, how will the volume of a gas change if the temperature is increased from 200 K to 400 K ?
View SolutionThe change in the magnitude of the volume of an ideal gas when a small additional pressure ΔP is applied at a constant temperature, is the same as the change when the temperature is reduced by a small quantity ΔT at constant pressure. The initial temperature and pressure of the gas were 300 K and 2 atm. respectively. If |ΔT|=C|ΔP| then value of C in (K/atm) is __________.
View SolutionWhat is the volume of a gas at absolute zero of temperature ?
View SolutionDuring a process, the volume of a gas is found to depend inversely on the square of its absolute temperature. Find how its pressure will depend on the absolute temperature.
View SolutionCp is always greater than Cv for a gas, which of the following statements provide, partly or wholly, the reason for this?
(i) No work is done by a gas at constant volume
(ii) When a gas absorbs heat at constant pressure, its volume must change
(iii) For the same change in temperature, the internal energy of a gas changes by a smaller amount at constant volume that at constant pressure
(iv) The internal energy of an ideal gas is a function only of its temperature
View Solution
