The temperature (T) of one mole of an ideal gas varies with its volume (V) as T=−αV3+βV2, where αandβ are positive constants. The maximum pressure of gas during this process is
αβ2R
β2R4α
(α+β)R2β2
α2R2β
The correct Answer is:B
To find the maximum pressure of one mole of an ideal gas when the temperature varies with volume as given by T=−αV3+βV2, we will follow these steps:
Step 1: Use the Ideal Gas Law
The ideal gas law is given by:
PV=nRT
Since we have one mole of gas (n=1), we can rewrite this as:
P=RTV
Substituting the expression for temperature T:
P=R(−αV3+βV2)V
This simplifies to:
P=R(−αV2+βV)
Step 2: Differentiate Pressure with Respect to Volume
To find the maximum pressure, we need to differentiate P with respect to V:
dPdV=R(−2αV+β)
Setting the derivative equal to zero to find critical points:
−2αV+β=0
From this, we can solve for V:
V=β2α
Step 3: Determine the Nature of the Critical Point
To confirm that this critical point is a maximum, we need to take the second derivative:
d2PdV2=−2Rα
Since α is positive, d2PdV2<0, indicating that P has a maximum at this value of V.
Step 4: Substitute Back to Find Maximum Pressure
Now we substitute V=β2α back into the expression for P:
Pmax=R(−α(β2α)2+β(β2α))
Calculating this gives:
Pmax=R(−αβ24α2+β22α)
=R(−β24α+2β24α)
=R(β24α)
Thus, the maximum pressure is:
Pmax=Rβ24α
Final Answer
The maximum pressure of the gas during this process is:
Pmax=Rβ24α
The ideal gas law is given by:
PV=nRT
Since we have one mole of gas (n=1), we can rewrite this as:
P=RTV
Substituting the expression for temperature T:
P=R(−αV3+βV2)V
This simplifies to:
P=R(−αV2+βV)
To find the maximum pressure, we need to differentiate P with respect to V:
dPdV=R(−2αV+β)
Setting the derivative equal to zero to find critical points:
−2αV+β=0
From this, we can solve for V:
V=β2α
To confirm that this critical point is a maximum, we need to take the second derivative:
d2PdV2=−2Rα
Since α is positive, d2PdV2<0, indicating that P has a maximum at this value of V.
Now we substitute V=β2α back into the expression for P:
Pmax=R(−α(β2α)2+β(β2α))
Calculating this gives:
Pmax=R(−αβ24α2+β22α)
=R(−β24α+2β24α)
=R(β24α)
Thus, the maximum pressure is:
Pmax=Rβ24α
The maximum pressure of the gas during this process is:
Pmax=Rβ24α
Topper's Solved these Questions
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - C Previous Years Questions
21 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - A Objective Type Questions
30 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
15 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-D) (Assertion-Reason Type Questions)
15 Videos
Similar Questions
Find the maximum attainable temperature of ideal gas in each of the following process :
(a) p=p0−αV2 ,
(b) p=p0e−βv,
where p0,α and β are positive constants, and V is the volume of one mole of gas.
View SolutionOne mole of an ideal gas undergoes a process
P=P0−αV2
where α and P0 are positive constant and V is the volume of one mole of gas
Q. When temperature is maximum, volume is
View SolutionOne mole of an ideal gas undergoes a process
P=P0−αV2
where α and P0 are positive constant and V is the volume of one mole of gas
Q. When temperature is maximum, volume is
View SolutionOne mole of an ideal gas undergoes a process
P=P0−αV2
where α and P0 are positive constant and V is the volume of one mole of gas
Q. The maximum attainable temperature is
View SolutionThe displacement x of a particle varies with time t as x=ae−αt+beβt. Where a,b,α and β positive constant.
The velocity of the particle will.
View SolutionThe displacement x of a particle varies with time t as x=ae−αt+beβt. Where a,b,α and β positive constant.
The velocity of the particle will.
View SolutionThe relation between the internal energy (U) versus temeperature (T) for a 3 mole ideal gas in an isochoric process is given as U=α+βT where α and β are constant. If the specific heat capacity of the gas for above process is βm find the value of m.
View SolutionOne mole of an ideal gas undergoes a process in which T=T0+aV3, where T0 and a are positive constants and V is molar volume. The volume for which pressure with be minimum is
View SolutionOne mole of an ideal gas undergoes a process in which T=T0+aV3, where T0 and a are positive constants and V is molar volume. The volume for which pressure with be minimum is
View SolutionOne mole of an ideal monotomic gas undergoes a linear process from A to B in which its pressure p and its volume V change as shown in figure.
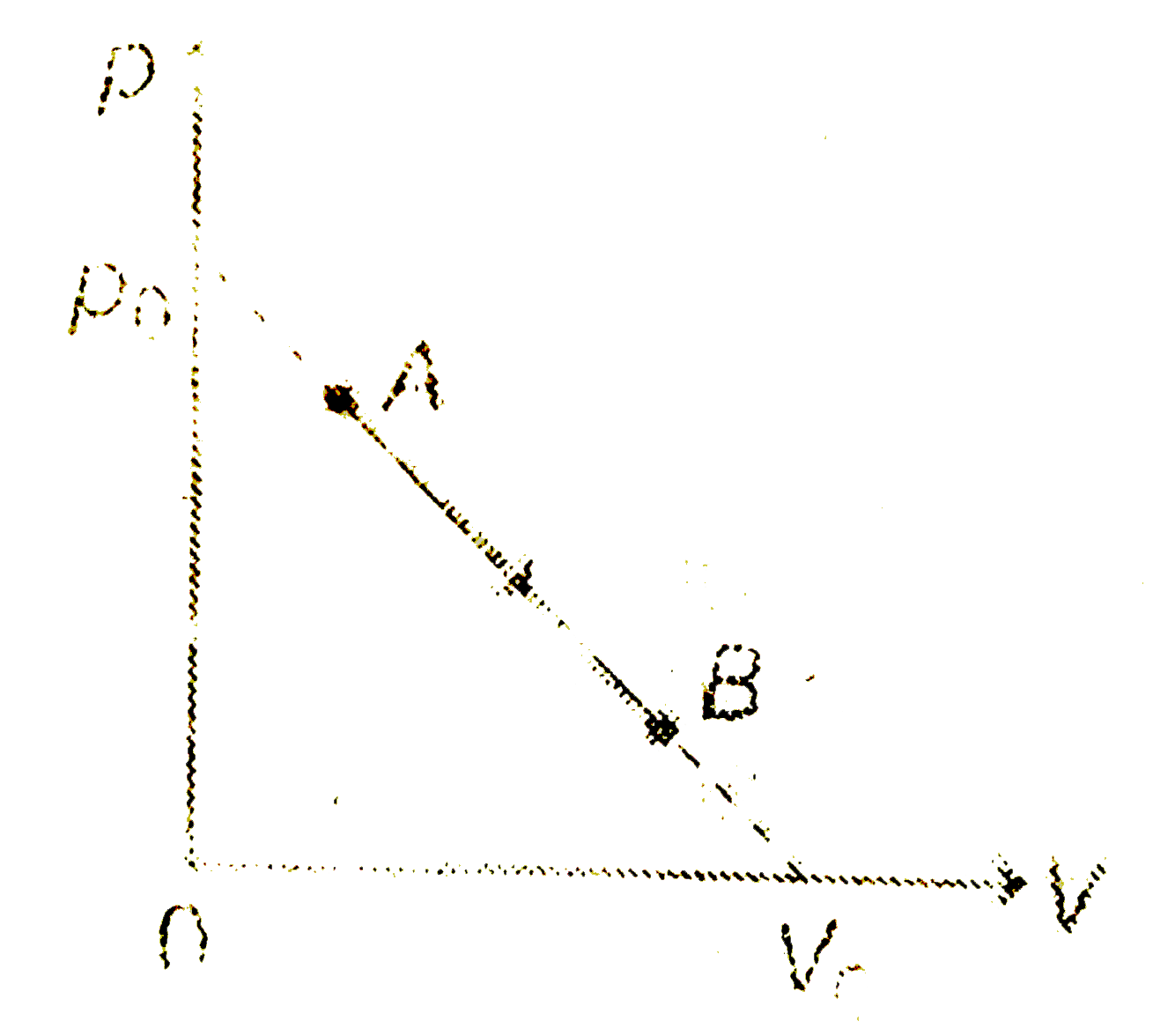
The maximum temperature of the gas during this process is
View Solution
