Two point objects of mass 2x and 3x are separated by a distance r. keeping the distance fixed, how much mass should be transferred from 3x to 2x , so that gravitational force between them becomes maximum ?
x4
x3
x2
2x3
The correct Answer is:C
To solve the problem of how much mass should be transferred from the object with mass 3x to the object with mass 2x in order to maximize the gravitational force between them, we can follow these steps:
Step 1: Define the masses after transfer
Let y be the mass transferred from 3x to 2x. After the transfer:
- The mass of the first object (originally 3x) becomes 3x−y.
- The mass of the second object (originally 2x) becomes 2x+y.
Step 2: Write the formula for gravitational force
The gravitational force F between two masses is given by the formula:
F=G⋅m1⋅m2r2
Substituting the new masses into this equation, we have:
F=G⋅(3x−y)⋅(2x+y)r2
Step 3: Simplify the expression
We can simplify this expression by factoring out the constant terms:
F=Gr2⋅(3x−y)(2x+y)
Let k=Gr2, then:
F=k⋅(3x−y)(2x+y)
Step 4: Expand the expression
Now, we expand the product:
F=k⋅(6x2+3xy−2xy−y2)=k⋅(6x2+xy−y2)
Step 5: Differentiate with respect to y
To find the maximum force, we need to differentiate F with respect to y and set the derivative equal to zero:
dFdy=k⋅(x−2y)=0
Step 6: Solve for y
Setting the derivative to zero gives:
x−2y=0⟹y=x2
Step 7: Verify it's a maximum
To confirm that this value of y gives a maximum, we can take the second derivative:
d2Fdy2=−2k
Since k>0, d2Fdy2<0, indicating that we have a maximum.
Conclusion
Thus, the amount of mass that should be transferred from 3x to 2x to maximize the gravitational force between them is:
x2
Let y be the mass transferred from 3x to 2x. After the transfer:
- The mass of the first object (originally 3x) becomes 3x−y.
- The mass of the second object (originally 2x) becomes 2x+y.
The gravitational force F between two masses is given by the formula:
F=G⋅m1⋅m2r2
Substituting the new masses into this equation, we have:
F=G⋅(3x−y)⋅(2x+y)r2
We can simplify this expression by factoring out the constant terms:
F=Gr2⋅(3x−y)(2x+y)
Let k=Gr2, then:
F=k⋅(3x−y)(2x+y)
Now, we expand the product:
F=k⋅(6x2+3xy−2xy−y2)=k⋅(6x2+xy−y2)
To find the maximum force, we need to differentiate F with respect to y and set the derivative equal to zero:
dFdy=k⋅(x−2y)=0
Setting the derivative to zero gives:
x−2y=0⟹y=x2
To confirm that this value of y gives a maximum, we can take the second derivative:
d2Fdy2=−2k
Since k>0, d2Fdy2<0, indicating that we have a maximum.
Thus, the amount of mass that should be transferred from 3x to 2x to maximize the gravitational force between them is:
x2
Topper's Solved these Questions
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - A (OBJECTIVE TYPE QUESTIONS)
41 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - B (OBJECTIVE TYPE QUESTIONS)
22 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - TRY YOUR SELF
33 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - ASSIGNMENT SECTION - D
9 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
Similar Questions
Two spheres of masses 16 kg and 4 kg are separated by a distance 30 m on a table. Then, the distance from sphere of mass 16 kg at which the net gravitational force becomes zero is
View SolutionTwo celestial bodies are separated by some distance. If the mass of any one of the bodies is doubled while the mass of other is halved then how far should they be taken so that the gravitational force between them becomes one-fourth ?
View SolutionTwo celestial bodies are separated by some distance. If the mass of any one of the bodies is doubled while the mass of other is halved then how far should they be taken so that the gravitational force between them becomes one-fourth ?
View SolutionThe separation between two masses is reduced to half. How is the magnitude of gravitational force between them affected ?
View SolutionTwo point masses m and 4 m are seperated by a distance d on a line . A third point mass m0 is to be placed at a point on the line such that the net gravitational force on it zero .

The distance of that point from the m mass is
View SolutionTwo bodies of masses M1 and M2 are kept separeated by a distance d. The potential at the point where the gravitational field produced by them is zero,the gravitational potential will be :-
View SolutionMass M=1 unit is divided into two parts X and (1−X). For a given separation the value of X for which the gravitational force between them becomes maximum is
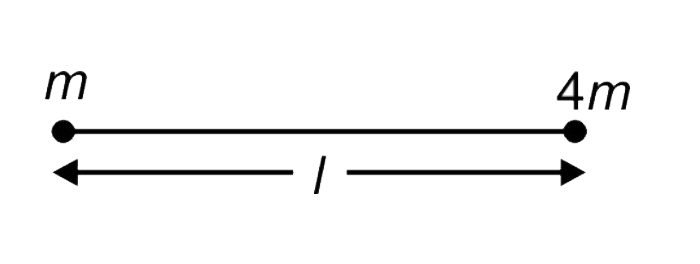
View SolutionTwo particles having masses m and 4m are separated by distance l. The distance of the centre of mass from m is x1andx2 is the distance of point at which gravitational field intensity is zero. Find the value of x1x2
View SolutionTwo points objects of mass 1.5g and 2.5g respectively are a distance of 16cm apart, the center of mass is at distance x from the object of mass 1.5g. Find the value of x
View SolutionTwo point particles of mass m and 2m are initially separated by a distance 4a. They are then released to become free to move. Find the velocities of both the particles when the distance between them reduces to a.
View Solution
