L,L2 and L3 are connected in series. Their radii are r, r2 and r3 respectively. Then, if stream-line flow is to be maintained and the pressure across the first capillary is P, then:
The pressure difference across the ends of second capillary is 8P
The pressure difference across the third capillary is 43P
The pressure difference across the ends of second capillary is 16P
The pressure difference across the third capillary is 59P
To solve the problem, we need to analyze the flow of fluid through three capillary tubes connected in series. The lengths and radii of the tubes are given, and we need to find the pressure difference across the second capillary tube.
1. Identify the Parameters:
- Lengths of the capillaries:
- First capillary: L
- Second capillary: L2
- Third capillary: L3
- Radii of the capillaries:
- First capillary: r
- Second capillary: r2
- Third capillary: r3
2. Use Poiseuille’s Law:
The volumetric flow rate Q through a capillary tube is given by:
Q=πr4P8ηL
where P is the pressure difference, η is the viscosity of the fluid, and L is the length of the tube.
3. Set Up Equations for Each Capillary:
- For the first capillary (length L, radius r):
Q1=πr4P18ηL
- For the second capillary (length L2, radius r2):
Q2=π(r2)4P28η(L2)=πr416P24ηL=πr4P264ηL
- For the third capillary (length L3, radius r3):
Q3=π(r3)4P38η(L3)=πr481P38L3=3πr4P3648ηL=πr4P3216ηL
4. Equate the Flow Rates:
Since the capillaries are in series, the flow rates must be equal:
Q1=Q2=Q3
5. Finding the Pressure Ratios:
From Q1=Q2:
πr4P18ηL=πr4P264ηL
Simplifying gives:
P1=81P2⟹P1=8P2
6. Finding the Pressure Across the Second Capillary:
Rearranging gives:
P2=P18
Thus, the pressure difference across the second capillary is:
P2=8P
Conclusion:
The pressure difference across the ends of the second capillary is 8P.
- Lengths of the capillaries:
- First capillary: L
- Second capillary: L2
- Third capillary: L3
- Radii of the capillaries:
- First capillary: r
- Second capillary: r2
- Third capillary: r3
The volumetric flow rate Q through a capillary tube is given by:
Q=πr4P8ηL
where P is the pressure difference, η is the viscosity of the fluid, and L is the length of the tube.
- For the first capillary (length L, radius r):
Q1=πr4P18ηL
- For the second capillary (length L2, radius r2):
Q2=π(r2)4P28η(L2)=πr416P24ηL=πr4P264ηL
- For the third capillary (length L3, radius r3):
Q3=π(r3)4P38η(L3)=πr481P38L3=3πr4P3648ηL=πr4P3216ηL
Since the capillaries are in series, the flow rates must be equal:
Q1=Q2=Q3
From Q1=Q2:
πr4P18ηL=πr4P264ηL
Simplifying gives:
P1=81P2⟹P1=8P2
Rearranging gives:
P2=P18
Thus, the pressure difference across the second capillary is:
P2=8P
The pressure difference across the ends of the second capillary is 8P.
Similar Questions
Three capillaries of length L,L2andL3 are connected in a series. Their radii are r,r2andr3 respectively. If a streamlined flow is to be maintained and pressure difference across the first capillary is ρ, then the pressure difference across the second capillary will be
View SolutionAt resonance in series R-L-C circuit
_PHY_E06_308_Q01.png)
View Solution
Knowledge Check
Two capillaries of same length and radii in the ratio 1:2 are connected in series. A liquid flows through them in streamlined condition. If the pressure across the two extreme ends of the combination is 1 m of water, the pressure difference across first capillary is
A9.4 mB4.9 mC0.49 mD0.94 mTwo copper wire of length l and 2l have radii, r and 2r respectively. What si the ratio of their specific resistance.?
A1:2B2:1C1:1D1:3
In an LCR series ac circuit the voltage across L,C and R are V1,V2 and V3 respectively The voltage of the source is .
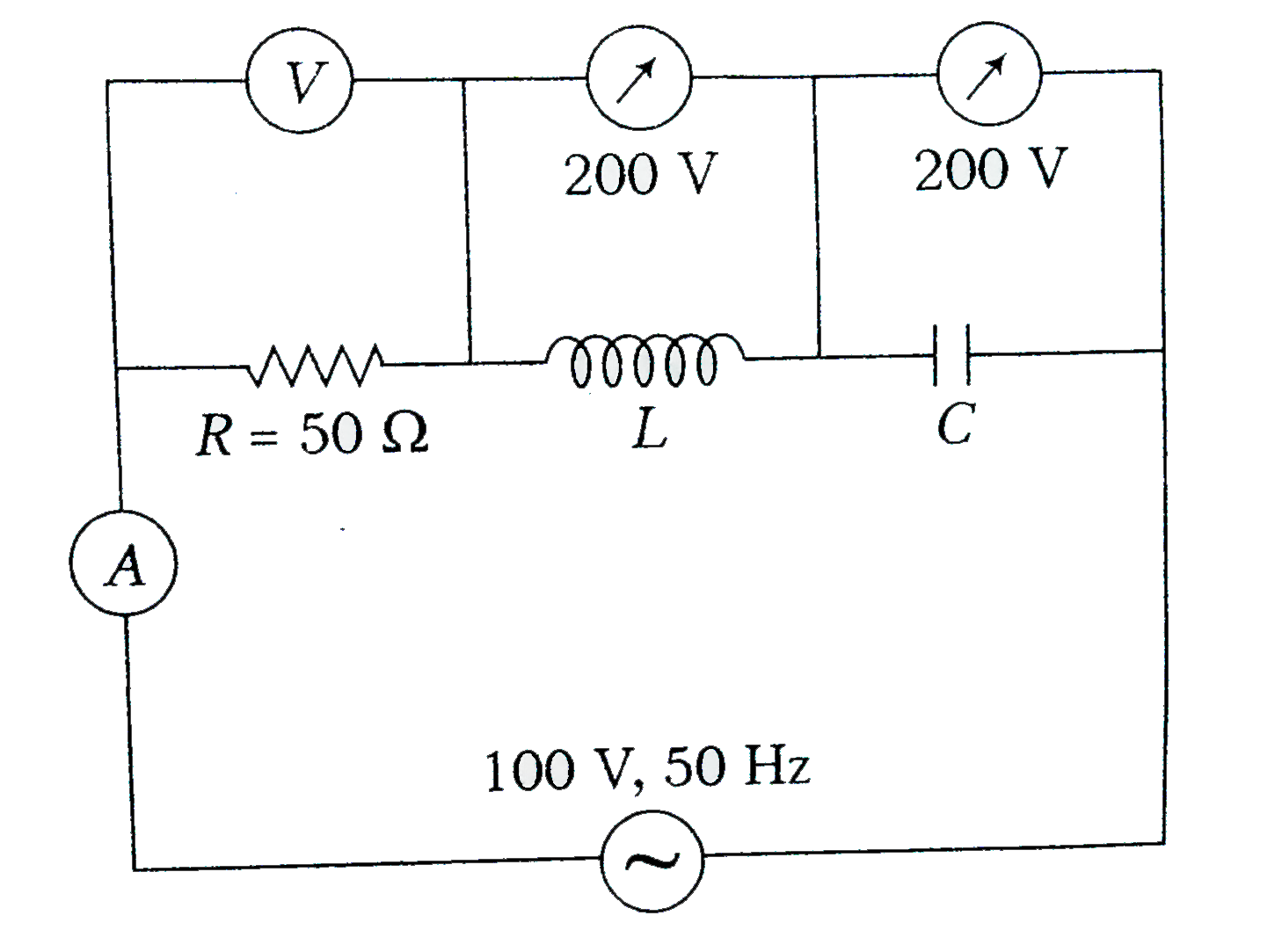
View SolutionIn the series L-C-R circuit , the voltmeter and ammeter readings are respectively
View SolutionThe rate of steady volume flow of water through a capillary tube of length ' l ' and radius ' r ' under a pressure difference of P is V . This tube is connected with another tube of the same length but half the radius in series. Then the rate of steady volume flow through them is (The pressure difference across the combination is P )
View SolutionIn L−C−R series circuit
View SolutionWe have two (narrow) capillary tubes T1 and T2 . Their lengths are l1 and l2 and radii of cross-section are r1 and r2 respectively. The rate of flow of water under a pressure difference P through tube T is 8cm3/sec. If l1=2l2 and r1=r2 what will be the rate of flow when the two tubes are connected in series and pressure difference across the combinatin is same as before (=P)
View SolutionIn a series L-R circuit, connected with a sinusoidal ac source, the maximum potential difference across L and R are respectivaly 3 volts and 4 volts.
At an instant the potemtial difference across resistor is 2 volts. The potential difference in volt, across the inductor at the same instant will be:
View Solution