A spring balance reads 200 gF when carrying a lump of lead in air. If the lead is now immersed with half of its volume in brine solution, what will be the new reading of the spring balance? . specific gravity of lead and brine are 11.4 and 1.1 respectively
190.4 gF .
T80.4 gF
210 gF
170.4 gF
To solve the problem, we need to determine the new reading of the spring balance when a lump of lead is partially immersed in a brine solution. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Initial Conditions
The spring balance reads 200 gF when the lead is in air. This reading represents the weight of the lead in air.
Step 2: Determine the Weight of the Lead
The weight of the lead (W) can be expressed as:
W=mg=200gF
Step 3: Calculate the Volume of the Lead
Using the specific gravity of lead (SG_lead = 11.4), we can find the density of lead:
Density of lead=SGlead×Density of water=11.4×1000kg/m3=11400kg/m3
Now, we can express the weight in terms of volume (V) and density (ρ):
W=ρlead⋅V⋅g
Since we already have W = 200 gF, we can rearrange to find V:
V=Wρlead⋅g
Step 4: Calculate the Volume of Lead
Convert 200 gF to kg:
200gF=0.2kg⋅g
Using g≈9.81m/s2:
V=0.2kg11400kg/m3≈1.754×10−5m3
Step 5: Determine the Buoyant Force When Half Immersed
When half of the lead’s volume is immersed in brine, the volume of lead submerged (V_submerged) is:
Vsubmerged=V2=1.754×10−52≈8.77×10−6m3
The specific gravity of brine (SG_brine = 1.1) gives us its density:
Density of brine=SGbrine×Density of water=1.1×1000kg/m3=1100kg/m3
Now, calculate the buoyant force (F_buoyant):
Fbuoyant=Densitybrine⋅Vsubmerged⋅g
Fbuoyant=1100kg/m3⋅8.77×10−6m3⋅9.81m/s2≈0.095N
Step 6: Calculate the New Reading of the Spring Balance
The new reading of the spring balance (F_new) can be calculated by considering the weight of the lead and the buoyant force:
Fnew=W−Fbuoyant
Convert the buoyant force to gram force:
Fbuoyant≈0.095N×1000g9.81N≈9.67gF
Now, calculate the new reading:
Fnew=200gF−9.67gF≈190.33gF
Final Answer
The new reading of the spring balance when the lead is half immersed in brine is approximately:
New Reading≈190.4gF
The spring balance reads 200 gF when the lead is in air. This reading represents the weight of the lead in air.
The weight of the lead (W) can be expressed as:
W=mg=200gF
Using the specific gravity of lead (SG_lead = 11.4), we can find the density of lead:
Density of lead=SGlead×Density of water=11.4×1000kg/m3=11400kg/m3
W=ρlead⋅V⋅g
Since we already have W = 200 gF, we can rearrange to find V:
V=Wρlead⋅g
Convert 200 gF to kg:
200gF=0.2kg⋅g
Using g≈9.81m/s2:
V=0.2kg11400kg/m3≈1.754×10−5m3
When half of the lead’s volume is immersed in brine, the volume of lead submerged (V_submerged) is:
Vsubmerged=V2=1.754×10−52≈8.77×10−6m3
Density of brine=SGbrine×Density of water=1.1×1000kg/m3=1100kg/m3
Fbuoyant=Densitybrine⋅Vsubmerged⋅g
Fbuoyant=1100kg/m3⋅8.77×10−6m3⋅9.81m/s2≈0.095N
The new reading of the spring balance (F_new) can be calculated by considering the weight of the lead and the buoyant force:
Fnew=W−Fbuoyant
Fbuoyant≈0.095N×1000g9.81N≈9.67gF
Fnew=200gF−9.67gF≈190.33gF
The new reading of the spring balance when the lead is half immersed in brine is approximately:
New Reading≈190.4gF
Similar Questions
The reading of a spring balance when a mass is weighed on it in air is 40 gm. When the mass is immersed in water, its reading is 20 gm. The specific gravity of the mass is
View SolutionA wooden block of volume 1000cm3 is suspended from a spring balance its weight is 12 N in air. It is suspended in water such that half of the block is below the surface of water. The reading of spring balance is
View SolutionA spring balance reads 10kg when a bucket of water is suspened from it . What will be the reading of the balance when an iron piece of mass 7.2kg suspended by a string is immersed with half its volume inside the water in the bucket? Relative density of iron is 7.2 a) 10 kg b) 10.5 kg c) 13.6 kg d) 17.2 kg
View SolutionA spring balance reads W1 when a ball is suspended from it. A weighing machine reads W2 when a tank of liquid is kept on it. When the ball is immersed in the liquid, the spring balance reads W3 and the weighing machine reads W4. Then, which of the following are not correct?
View SolutionA solid body floating in water has 15th of its volume immersed in it. What fraction of its volume will be immersed, if it floats in a liquid of specific gravity 1.2 ?
View SolutionWhen a piece of wood is suspended from the hook of a spring balance, it reads 70 gf. The wood is now lowered into water. What reading do you expect on the scale of spring balance?
View SolutionWhen a piece of wood is suspended from the hook of a spring balance, it reads 70 gf. The wood is now lowered into water.What reading do you expect on the scale of spring balance?
View SolutionA block hangs from a spring balance. The balance reads 40 N in air, 35 N when block is immersed in water and 25 N when block is immersed in another liquid. Find the density of block and other liquid.
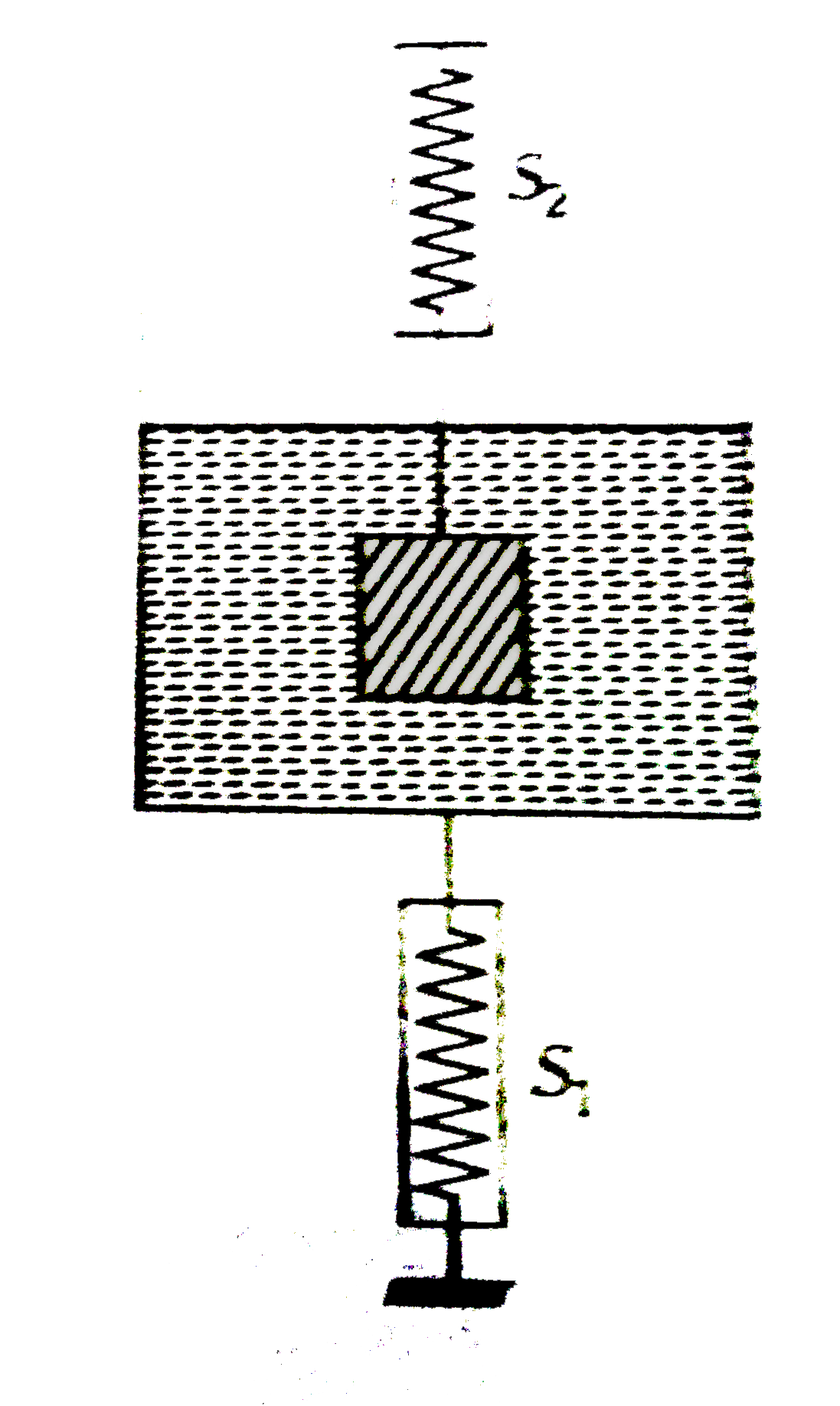
View SolutionA beaker containing water is kept on a spring scale. The mass of water and beaker is 5 kg . A block of mass 2 kg and specific gravity 10 is suspended by means of thread from a spring balance as shown. The readings of scales S1 and S2 are respectively Take, g=10ms−2
View SolutionAs Fig. shows, S1 and S2, are spring balances. Block A is hanging from spring balance S1 and immersed in liquid L which is contained in beaker B. The mass of beaker B is 1kg and mass of liquid L is 1.5kg. Balances S1 and S2 reads 2.5kg and 7.5kg, respectively. What will be the readings of S1 and S2 when block A is pulled up out of the liquid. Find the reading of S1 and S_(2)?
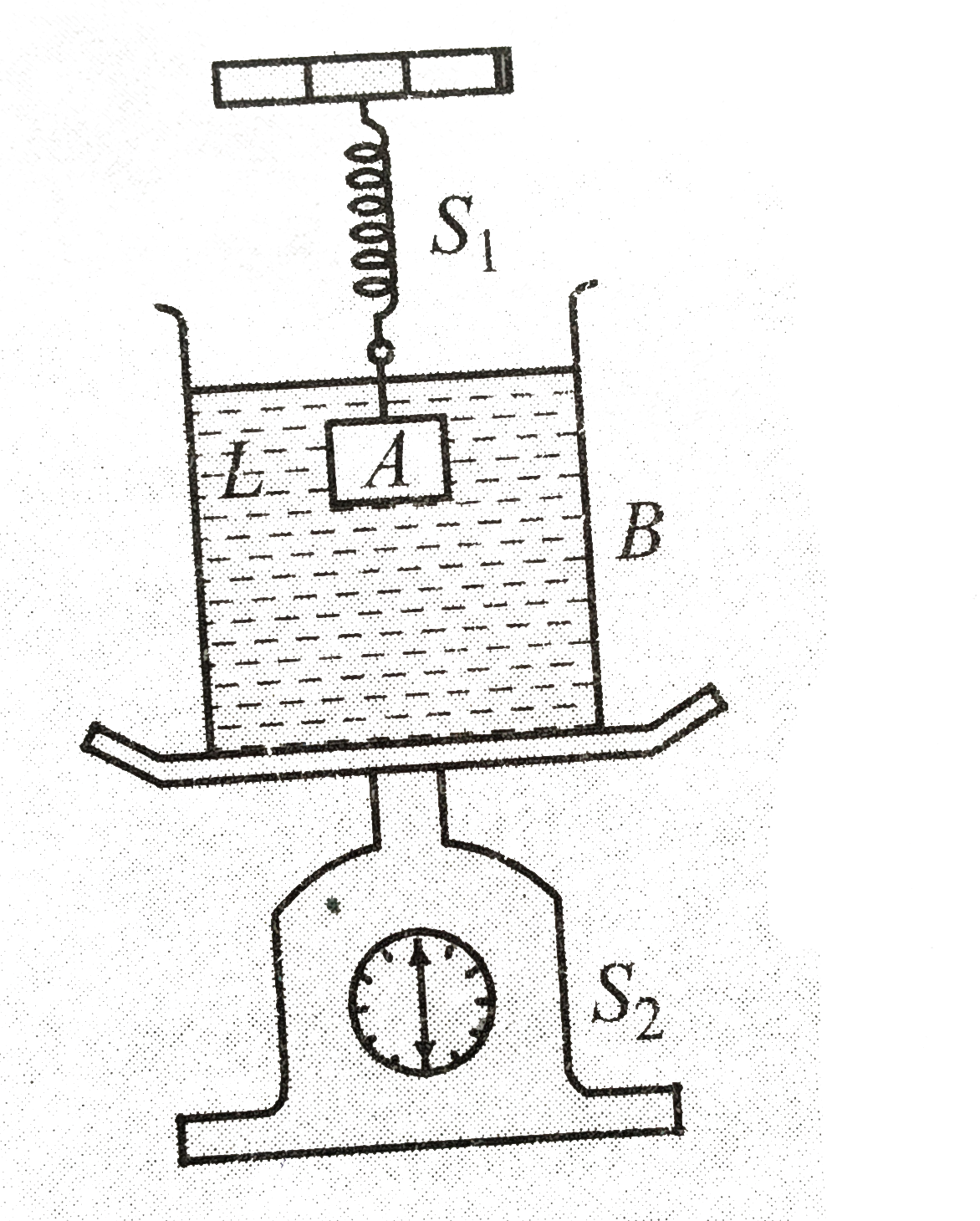
View Solution
