Heat energy at constant rate is given to two substances P and Q. If variation of temperature (T) of substances with time (t) is as shown in figure, then select the correct statement
A. Specific heat of P is greater than Q
B. Specific heat of Q is greater than P
C. Both have same specific heat
D. Data is insufficient to predict it
To solve the problem, we need to analyze the temperature versus time graph for substances P and Q and understand the relationship between the slope of the graph and the specific heat of the substances.
1. Understand the Graph:
- The graph shows the variation of temperature (T) with time (t) for two substances, P and Q.
- The slope of the graph represents the rate of change of temperature with respect to time.
2. Identify the Slope:
- The slope of the temperature vs. time graph can be mathematically expressed as:
slope=ΔTΔt
- Here, ΔT is the change in temperature and Δt is the change in time.
3. Relate Slope to Specific Heat:
- The heat energy (Q) supplied to a substance is related to its mass (m), specific heat (s), and the change in temperature (ΔT) by the formula:
Q=m⋅s⋅ΔT
- If heat is supplied at a constant rate, we can express the rate of heat transfer as:
Qt=m⋅s⋅ΔTt
- Rearranging gives:
ΔTΔt=Qm⋅s
- This indicates that the slope of the graph is inversely proportional to the specific heat capacity (s) of the substance.
4. Compare the Slopes:
- If the slope of substance P is greater than that of substance Q, it implies:
slopeP>slopeQ⇒sP<sQ
- Therefore, the specific heat of substance Q is greater than that of substance P.
5. Conclusion:
- Based on the analysis, we conclude that the correct statement is:
- The specific heat of substance Q is greater than that of substance P.
Final Answer:
- The specific heat of substance Q is greater than that of substance P.
- The graph shows the variation of temperature (T) with time (t) for two substances, P and Q.
- The slope of the graph represents the rate of change of temperature with respect to time.
- The slope of the temperature vs. time graph can be mathematically expressed as:
slope=ΔTΔt
- Here, ΔT is the change in temperature and Δt is the change in time.
- The heat energy (Q) supplied to a substance is related to its mass (m), specific heat (s), and the change in temperature (ΔT) by the formula:
Q=m⋅s⋅ΔT
- If heat is supplied at a constant rate, we can express the rate of heat transfer as:
Qt=m⋅s⋅ΔTt
- Rearranging gives:
ΔTΔt=Qm⋅s
- This indicates that the slope of the graph is inversely proportional to the specific heat capacity (s) of the substance.
- If the slope of substance P is greater than that of substance Q, it implies:
slopeP>slopeQ⇒sP<sQ
- Therefore, the specific heat of substance Q is greater than that of substance P.
- Based on the analysis, we conclude that the correct statement is:
- The specific heat of substance Q is greater than that of substance P.
- The specific heat of substance Q is greater than that of substance P.
Similar Questions
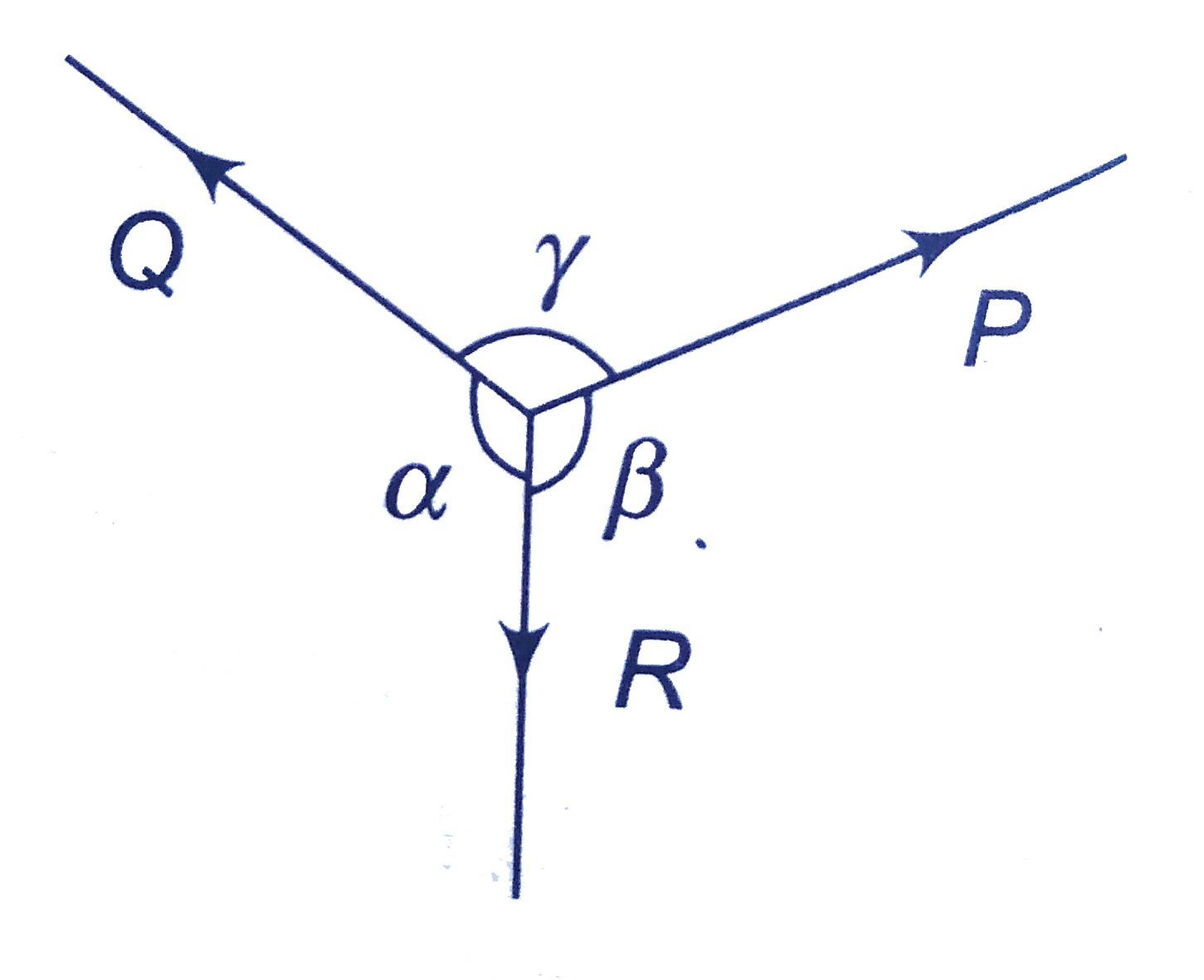
A body is in equilibrium under the action of three coplanar forces P,QandR as shown in figure. Select the correct statement.
View SolutionThe momentum p of an object varies with time (t) as shown in figure. The corresponding force (F)- time (t) graph is -
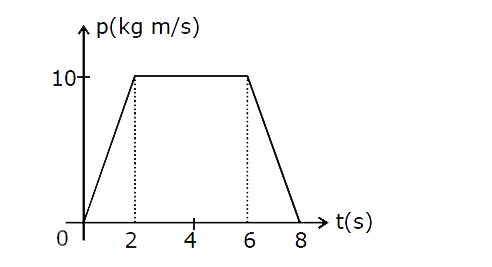
View SolutionThe variation of decay rate of two radioactive samples A and B with time is shown in fig.
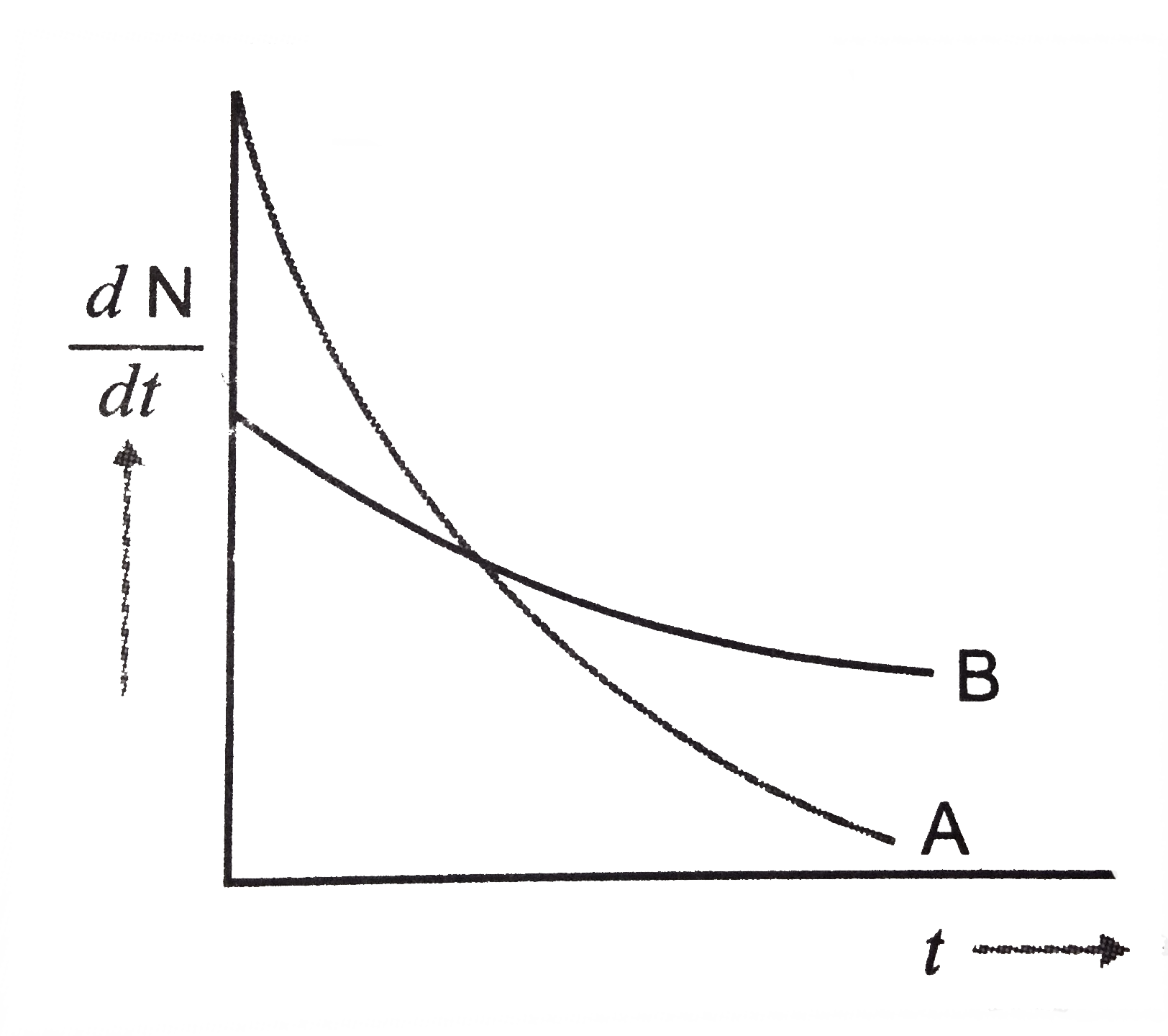
Which of the following statements are true?
View SolutionThe variation of decay rate of two radioactive samples A and B with time is shown in fig.

Which of the following statements are true?
View SolutionIn the circuit shown in the figure, switch S is closed at time t=0. Select the correct statements.
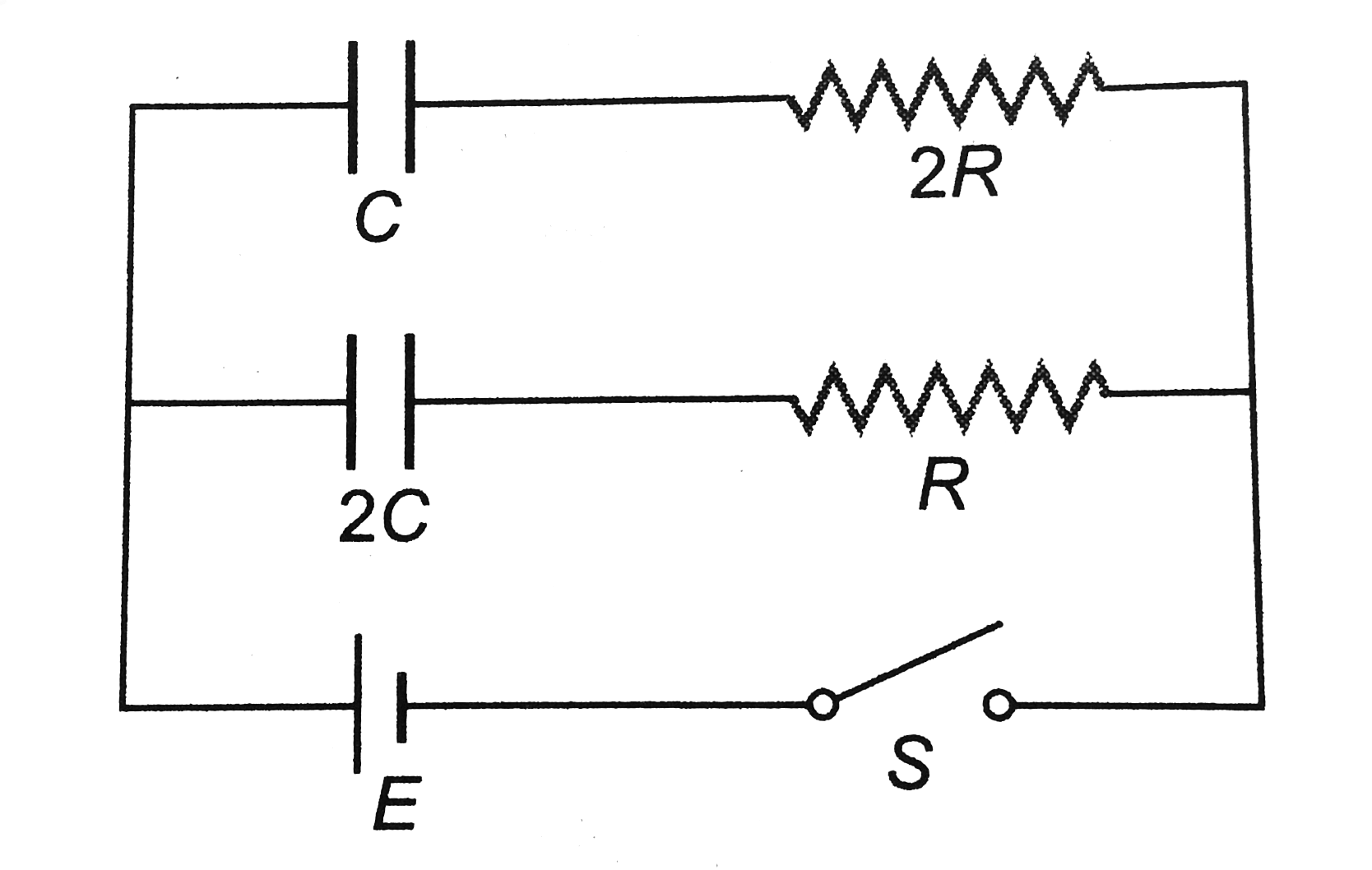
View SolutionIn the circuit shown in the figure, switch S is closed at time t=0. Select the correct statements.

View SolutionA force F is applied to the initially stationary cart. The variation of force with time is shown in the figure. The speed of cart at t = 5 sec is
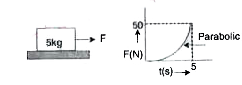
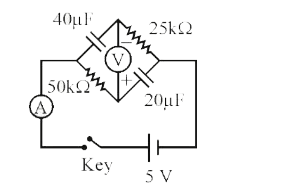
View SolutionIn the circuit shown below, the key is pressed at time t = 0. The correct statement is
View SolutionA heat engine is a system that converts heat into mechanical work. A heat "source" generates thermal energy that brings a working substance to a high temperature. The working substance then generates work in the engine while transferring heat to a sink at a lower temperature . The working of a heat engine is shownn figure 1.
 Heat engines can be modelled using themodynamic cycles. The heat engine given in Figure -2 is of a working substance which is 1.00 mol of a monoatomic ideal gas . The thermodynamic cycle begins at the point designated as '1' and goes clockwise and the values of P and l or V at each point is as given below Fig-2 (P1=1.00atm and V1=24.6L,P2=2.00atm,V3=49.2L,P4=1.00atm)
Heat engines can be modelled using themodynamic cycles. The heat engine given in Figure -2 is of a working substance which is 1.00 mol of a monoatomic ideal gas . The thermodynamic cycle begins at the point designated as '1' and goes clockwise and the values of P and l or V at each point is as given below Fig-2 (P1=1.00atm and V1=24.6L,P2=2.00atm,V3=49.2L,P4=1.00atm)
Calculate T1,T2,T3andT4
View SolutionA body is performing simple harmonic motion with amplitude a and time period T variation of its acceleration (f) with time(t) is shown in figure If at time t velocity of the body is v which of the following graph is correct?
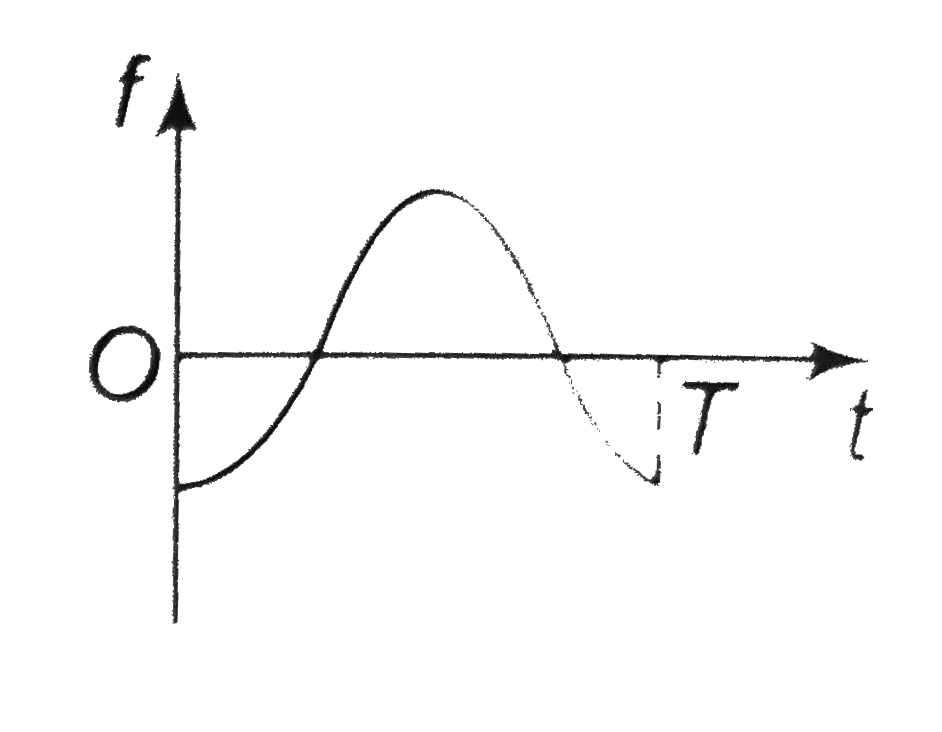
View Solution
