The flux through the hemispherical surface in the figure shwon below is
πR2E
2πR2E
πRE
Zero
To find the electric flux through the hemispherical surface, we can follow these steps:
Step 1: Understand the Concept of Electric Flux
Electric flux (Φ) through a surface is defined as the product of the electric field (E) and the area (A) of the surface, taking into account the angle (θ) between the electric field and the normal to the surface. Mathematically, it is expressed as:
Φ=∫→E⋅d→A=E⋅A⋅cos(θ)
Step 2: Analyze the Given Situation
In this problem, we have a hemispherical surface placed in a uniform electric field →E. The radius of the hemisphere is R. It is also given that there are no charges inside the hemisphere.
Step 3: Apply Gauss's Law
According to Gauss's law, the total electric flux through a closed surface is equal to the charge enclosed divided by the permittivity of free space ϵ0:
Φ=qϵ0
Since there are no charges inside the hemisphere, q=0. Therefore, the total flux through the closed surface (the hemisphere plus its circular base) is:
Φtotal=0
Step 4: Break Down the Total Flux
The total flux can be expressed as the sum of the flux through the hemispherical part and the flux through the circular base:
Φtotal=Φhemisphere+Φcircular base=0
This implies:
Φhemisphere=−Φcircular base
Step 5: Calculate the Flux through the Circular Base
The area A of the circular base is given by:
A=πR2
The flux through the circular base can be calculated as:
Φcircular base=E⋅A⋅cos(180∘)=−E⋅πR2
Here, cos(180∘)=−1 because the electric field is directed opposite to the outward normal of the circular base.
Step 6: Substitute Back to Find the Flux through the Hemisphere
Substituting the value of Φcircular base into the equation from Step 4:
Φhemisphere=−(−E⋅πR2)=E⋅πR2
Conclusion
Thus, the electric flux through the hemispherical surface is:
Φhemisphere=E⋅πR2
Final Answer
The flux through the hemispherical surface is E⋅πR2.
---
Electric flux (Φ) through a surface is defined as the product of the electric field (E) and the area (A) of the surface, taking into account the angle (θ) between the electric field and the normal to the surface. Mathematically, it is expressed as:
Φ=∫→E⋅d→A=E⋅A⋅cos(θ)
In this problem, we have a hemispherical surface placed in a uniform electric field →E. The radius of the hemisphere is R. It is also given that there are no charges inside the hemisphere.
According to Gauss's law, the total electric flux through a closed surface is equal to the charge enclosed divided by the permittivity of free space ϵ0:
Φ=qϵ0
Since there are no charges inside the hemisphere, q=0. Therefore, the total flux through the closed surface (the hemisphere plus its circular base) is:
Φtotal=0
The total flux can be expressed as the sum of the flux through the hemispherical part and the flux through the circular base:
Φtotal=Φhemisphere+Φcircular base=0
This implies:
Φhemisphere=−Φcircular base
The area A of the circular base is given by:
A=πR2
The flux through the circular base can be calculated as:
Φcircular base=E⋅A⋅cos(180∘)=−E⋅πR2
Here, cos(180∘)=−1 because the electric field is directed opposite to the outward normal of the circular base.
Substituting the value of Φcircular base into the equation from Step 4:
Φhemisphere=−(−E⋅πR2)=E⋅πR2
Thus, the electric flux through the hemispherical surface is:
Φhemisphere=E⋅πR2
The flux through the hemispherical surface is E⋅πR2.
Similar Questions
Find flux through the hemispherical surface
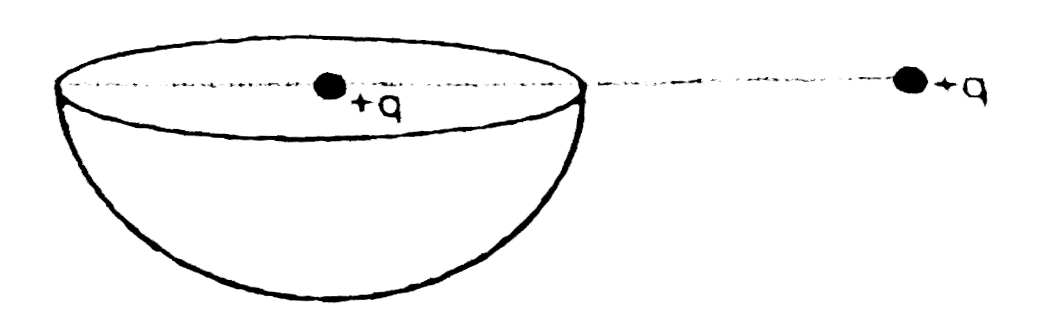
View SolutionElectric flux through a spherical surface shown in the figure is
 _______
_______
View SolutionThe electric flux through the surface

View SolutionThe electric flux through the surface

View SolutionThe electric flux through the surface

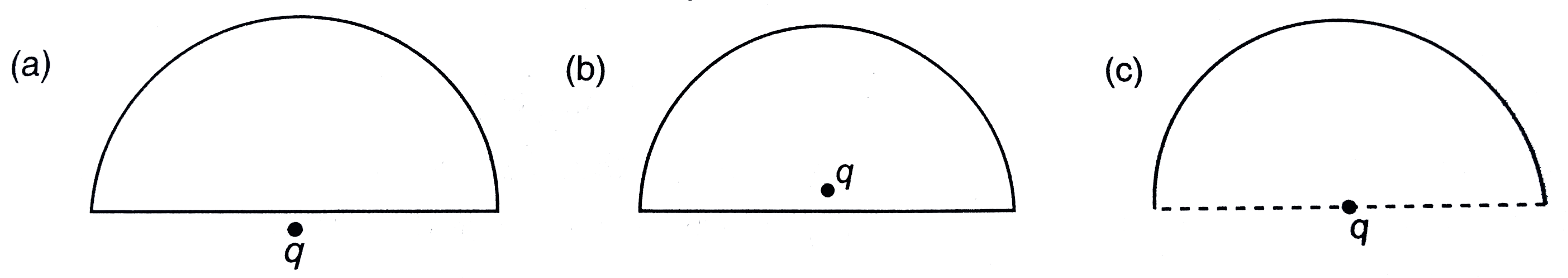
View SolutionIn figure (a), a charge q is placed just outside the centre of a closed hemisphere. In figure (b), the same charge q is placed just inside the centre of the closed hemisphere and in figure (c), the charge is placed at the centre of hemisphere open from the base. Find the electric flux passing through the hemisphere in all the three cases.
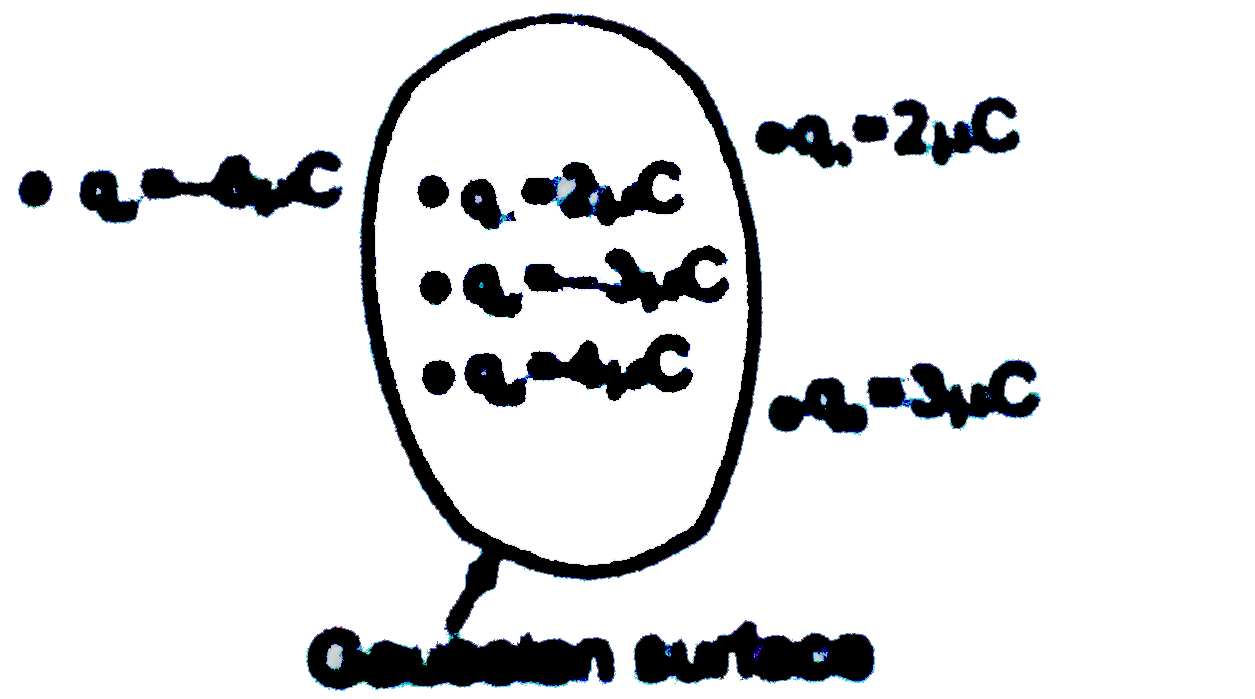
View SolutionFind out flux through the given Gaussian surface.
View SolutionA uniform electric field exists in space. Find the flux of this field through a cylindrical surface with the axis parallel to the field.
View SolutionA charge 'Q' is placed at the centre of a hemispherical surface of radius 'R'. The flux of electric field due to charge 'Q' through the surface of hemisphere is
View SolutionA metallic ring is connected to a rod oscillates freely like a pendulum. If now a magnetic field is applied in horizontal direction so that the pendulum now swings through the field, the pendulum will

View Solution
