Figure shows a long straight wire carrying current , and a square conducting wire loop of side i, at a distance 'a' from current wire. Both the current wire and loop arc in the plane of paper. Find the flux of magnetic field of current wire, passing through the loop.
To find the magnetic flux of the magnetic field produced by a long straight wire carrying current through a square conducting loop, we can follow these steps:
Step 1: Understand the Magnetic Field due to a Long Straight Wire
The magnetic field B at a distance x from a long straight wire carrying current I is given by the formula:
B=μ0I2πx
where μ0 is the permeability of free space.
Step 2: Set Up the Problem
We have a square loop of side l placed at a distance a from the wire. The loop is parallel to the wire, and we need to find the magnetic flux through this loop.
Step 3: Calculate the Magnetic Flux
The magnetic flux Φ through the loop can be calculated using the formula:
Φ=∫B⋅dA
where dA is the differential area element of the loop. Since the magnetic field is not uniform across the loop, we will integrate over the area of the loop.
Step 4: Express the Area Element
For a square loop, the area element dA can be expressed as:
dA=ldx
where dx is an infinitesimal width along the length of the loop.
Step 5: Set Up the Integral for Flux
The total flux through the loop can be expressed as:
Φ=∫l0B⋅ldx
Substituting the expression for B:
Φ=∫l0(μ0I2π(a+x))ldx
Step 6: Evaluate the Integral
Now we can evaluate the integral:
Φ=μ0Il2π∫l01a+xdx
The integral ∫1a+xdx evaluates to ln(a+x). Therefore:
Φ=μ0Il2π(ln(a+x))l0
This results in:
Φ=μ0Il2π(ln(a+l)−ln(a))
Using the properties of logarithms, we can simplify this to:
Φ=μ0Il2πln(a+la)
Final Result
Thus, the magnetic flux Φ through the square loop is:
Φ=μ0Il2πln(a+la)
The magnetic field B at a distance x from a long straight wire carrying current I is given by the formula:
B=μ0I2πx
where μ0 is the permeability of free space.
We have a square loop of side l placed at a distance a from the wire. The loop is parallel to the wire, and we need to find the magnetic flux through this loop.
The magnetic flux Φ through the loop can be calculated using the formula:
Φ=∫B⋅dA
where dA is the differential area element of the loop. Since the magnetic field is not uniform across the loop, we will integrate over the area of the loop.
For a square loop, the area element dA can be expressed as:
dA=ldx
where dx is an infinitesimal width along the length of the loop.
The total flux through the loop can be expressed as:
Φ=∫l0B⋅ldx
Substituting the expression for B:
Φ=∫l0(μ0I2π(a+x))ldx
Now we can evaluate the integral:
Φ=μ0Il2π∫l01a+xdx
The integral ∫1a+xdx evaluates to ln(a+x). Therefore:
Φ=μ0Il2π(ln(a+x))l0
This results in:
Φ=μ0Il2π(ln(a+l)−ln(a))
Using the properties of logarithms, we can simplify this to:
Φ=μ0Il2πln(a+la)
Thus, the magnetic flux Φ through the square loop is:
Φ=μ0Il2πln(a+la)
Similar Questions
Figure shows a long straight wire carrying current I and a square conducting wire loop of side l, at a distance 'a' from current wire. Both the current wire and loop are in the plane of paper. Find the flux of magnetic filed of current wire, passing through the loop.
View SolutionA long wire carrying a steady current I lies in the plane of circular conducting loop placed at a certain distance from the wire. There will be an induced current in the loop if it is
View SolutionA square loop of side a carris a current I. The magnetic field at the centre of the loop is
View SolutionA long straight wire is carrying a current of 25 A. A squre loop of side length 10 cm carrying a current of 15 A, is placed 2 cm from the wire as shown in figure. The force on the loop is
View SolutionA conducting circular loop of radius a is connected to two long, straight wires . The straight wires carry a current I as shown in figure . Find the magnetic field B at the centre of the loop.
View SolutionShows a long current carrying wire and two rectangular loops moving with of velocity v. Find the direction of current in each loop.
View SolutionShows a long current carrying wire and two rectangular loops moving with of velocity v. Find the direction of current in each loop.
View SolutionShows a long current carrying wire and two rectangular loops moving with of velocity v. Find the direction of current in each loop.
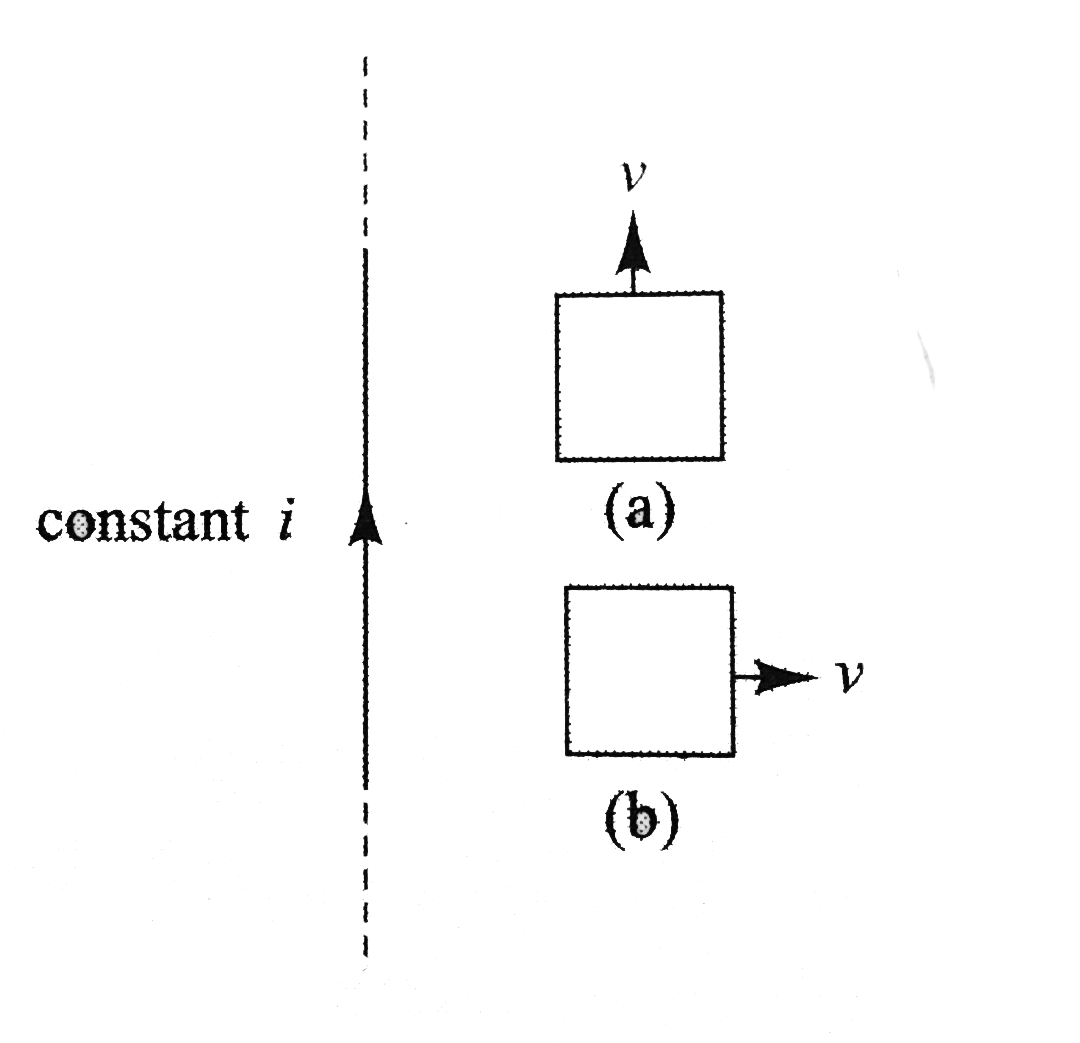View SolutionFigure shows a conducting loop placed near a long straight wire carrying a current i as shown, if the current increases continuously, find the direction of the induced current in the loop.
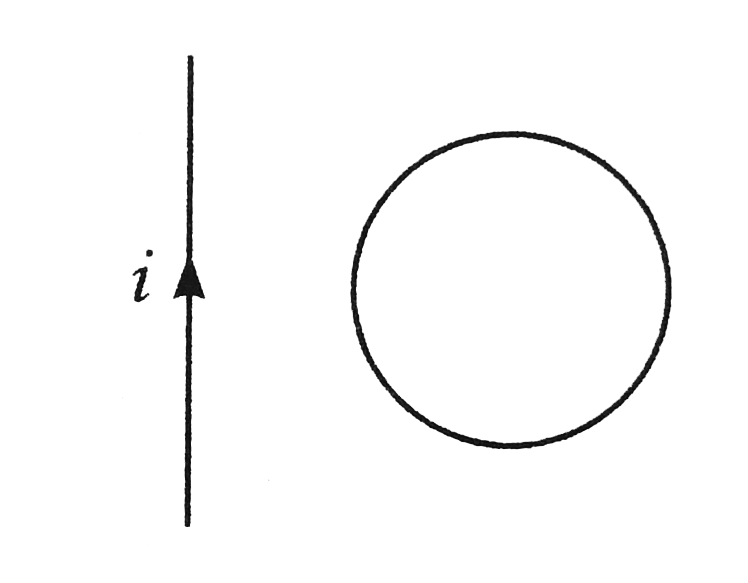View SolutionA triangular loop (ABC) having current i2 and an infinite wire having current i1 are placed in the same plane.Find the magnetic force of interaction between the infinite wire and the loop ABC.
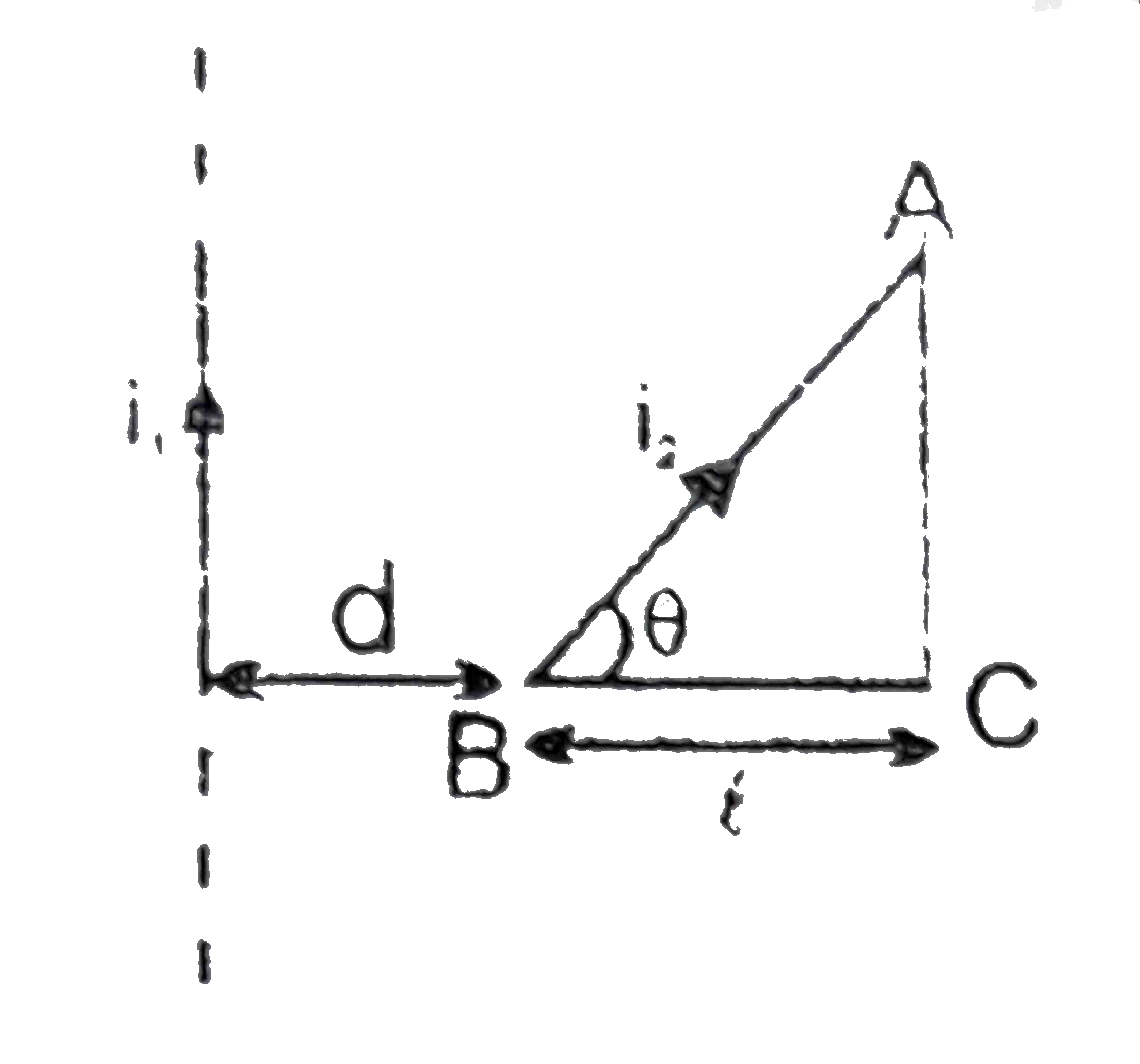View Solution