In a series LCR circuit which is connected to an AC vottage source, choose the incorrect statement
Algebraic sum of instantaneous vottage across L, C, R is a variable
VLinst+VCinst+VRinst=VSourceinst
Vottage across inductor, capacitor, resistance behave as a vector
Current is same in inductor, capacitor and resistance
The correct Answer is:C
To solve the question regarding the incorrect statement in a series LCR circuit connected to an AC voltage source, we will analyze each option provided and determine which one is incorrect.
1. Understand the Circuit:
- A series LCR circuit consists of an inductor (L), a capacitor (C), and a resistor (R) connected in series to an AC voltage source.
2. Analyze Option A:
- Statement: "The algebraic sum of instantaneous voltage across LCR is variable."
- Analysis: In an LCR circuit, the instantaneous voltages across each component (inductor, capacitor, and resistor) change with time due to the alternating current. Therefore, the sum of these instantaneous voltages is indeed variable.
- Conclusion: This statement is true.
3. Analyze Option B:
- Statement: "The instantaneous voltage across inductor plus voltage drop across the capacitor and the voltage drop across the resistor is equal to the voltage source instantaneous."
- Analysis: The correct relationship is given by the equation V=VR+VL−VC (considering the phase differences). Thus, the sum of the voltages across the inductor and capacitor does not equal the source voltage instantaneously due to phase differences.
- Conclusion: This statement is false (incorrect).
4. Analyze Option C:
- Statement: "Voltage across inductor, capacitor, and resistor behaves as a vector."
- Analysis: In AC circuits, voltages are represented as phasors (vectors) because they have both magnitude and phase. Therefore, this statement is true.
5. Analyze Option D:
- Statement: "Current is the same in the inductor, capacitor, and resistance."
- Analysis: In a series circuit, the same current flows through all components. Therefore, this statement is true.
6. Final Conclusion:
- The incorrect statement is Option B: "The instantaneous voltage across inductor plus voltage drop across the capacitor and the voltage drop across the resistor is equal to the voltage source instantaneous."
Summary:
The incorrect statement in the series LCR circuit connected to an AC voltage source is Option B.
---
- A series LCR circuit consists of an inductor (L), a capacitor (C), and a resistor (R) connected in series to an AC voltage source.
- Statement: "The algebraic sum of instantaneous voltage across LCR is variable."
- Analysis: In an LCR circuit, the instantaneous voltages across each component (inductor, capacitor, and resistor) change with time due to the alternating current. Therefore, the sum of these instantaneous voltages is indeed variable.
- Conclusion: This statement is true.
- Statement: "The instantaneous voltage across inductor plus voltage drop across the capacitor and the voltage drop across the resistor is equal to the voltage source instantaneous."
- Analysis: The correct relationship is given by the equation V=VR+VL−VC (considering the phase differences). Thus, the sum of the voltages across the inductor and capacitor does not equal the source voltage instantaneously due to phase differences.
- Conclusion: This statement is false (incorrect).
- Statement: "Voltage across inductor, capacitor, and resistor behaves as a vector."
- Analysis: In AC circuits, voltages are represented as phasors (vectors) because they have both magnitude and phase. Therefore, this statement is true.
- Statement: "Current is the same in the inductor, capacitor, and resistance."
- Analysis: In a series circuit, the same current flows through all components. Therefore, this statement is true.
- The incorrect statement is Option B: "The instantaneous voltage across inductor plus voltage drop across the capacitor and the voltage drop across the resistor is equal to the voltage source instantaneous."
The incorrect statement in the series LCR circuit connected to an AC voltage source is Option B.
Similar Questions
Knowledge Check
In the series LCR circuit shown the impedance is
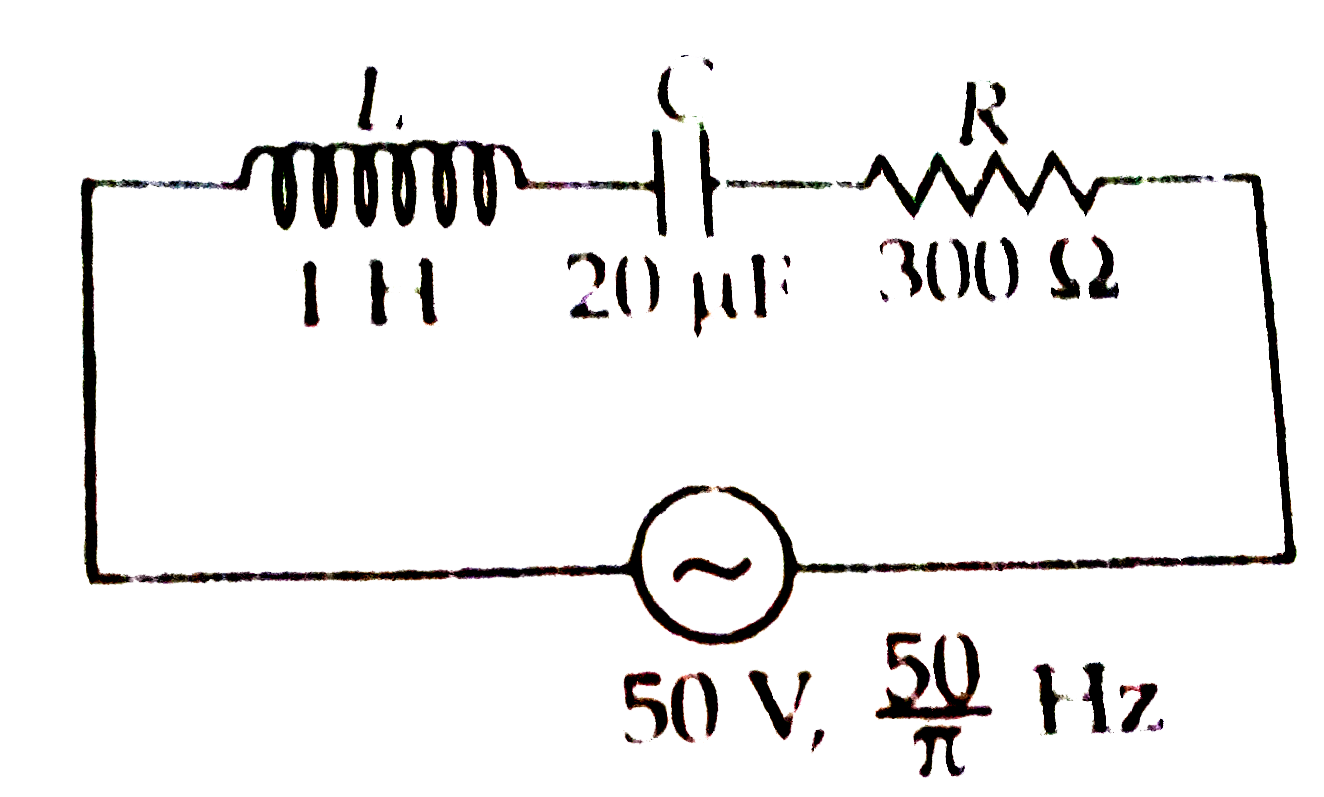 A200ΩB100ΩC300ΩD500Ω
A200ΩB100ΩC300ΩD500Ω
In the series LCR circuit shown the impedance is
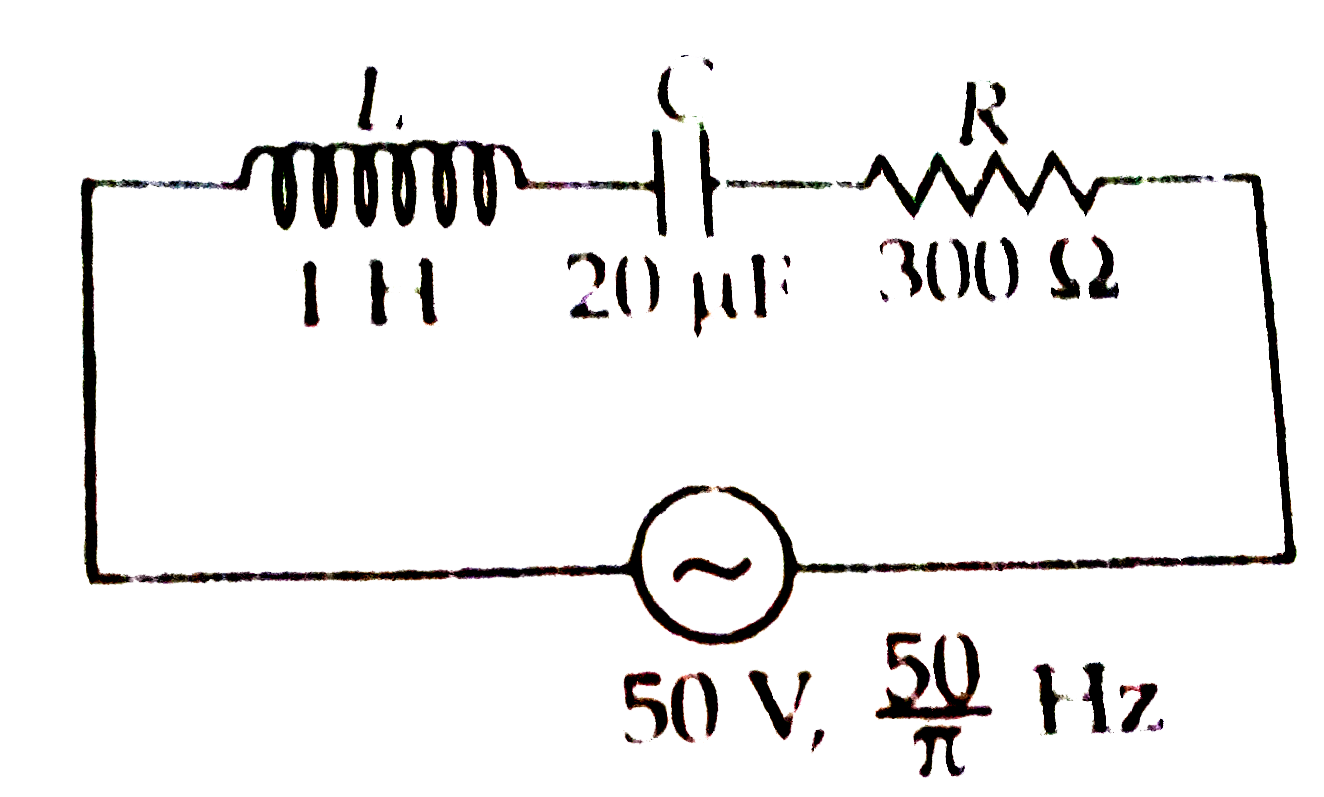
View Solution(a) In a series LCR circuit connected across an AC source of variable frequency, obtain the expression for its impedance and draw a plot showing its variation with frequency of the AC source.
(b) What is the phase difference between the voltages across inductor and the capacitor at resonance in the LCR circuit ?
(c ) When and inductor is connected to a 200 V DC voltage, a current of 1Aflows trough it. when the same inductor is connected to a 200 V, 50 Hz AC source, only 0.5 A current flows. Explain, why ? Also, calculate the self inductance of the inductor.
View Solution(a) Deriver and expression for the average power consumed in a series LCR circuit connected to a.c., source in which the phase different between the voltage and the current in the circuit is ϕ.
(b) Define the quality factor in an a.c. circuit. Why should the quality factor have high value in receiving circuits ? Name the factors on which it depends.
View SolutionIn a series L−R circuit, connected with a sinusoidal ac source, the maximum potential difference acrosssd L and R are respectively 3 volts and 4 volts
At the same instant, the magnitude of the potential difference in volt, across the ac source will be
View SolutionIn a series LCR circuit, at resonance, power factor is …….. .
View SolutionStatement 1: In a series LCR circuit at resonance condition power consumed by ciccuit is maximum.
Statement 2 : At resonance condition, the effective resistance of circuit is maximum.
View SolutionA series RLC circuit is connected to an ac generator. The instant at which current in the circuit is zero, the energy stored in the capacitor and inductor are:
View Solution
