In an oscillating LC circuit the maximum charge on the capacitor is Q. The charges on the capacitor when the energy is stored equally between the electric and magnetic field is
Q2
Q√2
Q√3
Q3
The correct Answer is:B
To solve the problem, we need to determine the charge on the capacitor when the energy is stored equally between the electric field and the magnetic field in an oscillating LC circuit.
1. Understand the Total Energy in the LC Circuit:
The total energy E stored in an LC circuit is given by the formula:
E=12Q2C
where Q is the maximum charge on the capacitor and C is the capacitance.
2. Energy Distribution:
When the energy is equally distributed between the electric field (capacitor) and the magnetic field (inductor), we have:
Electric Energy=Magnetic Energy
Therefore, we can write:
12Q2C=12q2C+12Li2
where q is the charge on the capacitor at that instant, L is the inductance, and i is the current.
3. Equating Energies:
Since the energies are equal, we can set the electric energy equal to the magnetic energy:
12Q2C=12q2C+12Li2
From this, we can simplify:
Q2C=q2C+Li2
4. Relating Current and Charge:
In an LC circuit, the current i can be expressed in terms of charge q as:
i=−dqdt
However, at the moment when the energy is equally divided, we can use the relationship:
i2=(Q2−q2L)
This leads us to the conclusion that:
Q2C=q2C+(Q2−q2)L
5. Finding Charge q:
Since we want the energy to be equally divided, we can set:
Q2C=2⋅q2C
This simplifies to:
Q2=2q2
Solving for q:
q2=Q22
Taking the square root gives:
q=Q√2
Final Answer:
The charge on the capacitor when the energy is stored equally between the electric and magnetic field is:
q=Q√2
The total energy E stored in an LC circuit is given by the formula:
E=12Q2C
where Q is the maximum charge on the capacitor and C is the capacitance.
When the energy is equally distributed between the electric field (capacitor) and the magnetic field (inductor), we have:
Electric Energy=Magnetic Energy
Therefore, we can write:
12Q2C=12q2C+12Li2
where q is the charge on the capacitor at that instant, L is the inductance, and i is the current.
Since the energies are equal, we can set the electric energy equal to the magnetic energy:
12Q2C=12q2C+12Li2
From this, we can simplify:
Q2C=q2C+Li2
In an LC circuit, the current i can be expressed in terms of charge q as:
i=−dqdt
However, at the moment when the energy is equally divided, we can use the relationship:
i2=(Q2−q2L)
This leads us to the conclusion that:
Q2C=q2C+(Q2−q2)L
Since we want the energy to be equally divided, we can set:
Q2C=2⋅q2C
This simplifies to:
Q2=2q2
Solving for q:
q2=Q22
Taking the square root gives:
q=Q√2
The charge on the capacitor when the energy is stored equally between the electric and magnetic field is:
q=Q√2
Similar Questions
Knowledge Check
A fully charged capacitor C with initial charge q0 is connected to a coil of self inductance L at t=0. The time at which the energy is stored equally between the electric and the magnetic fields is
Aπ4√LCB2π√LCC√LCDπ√LC
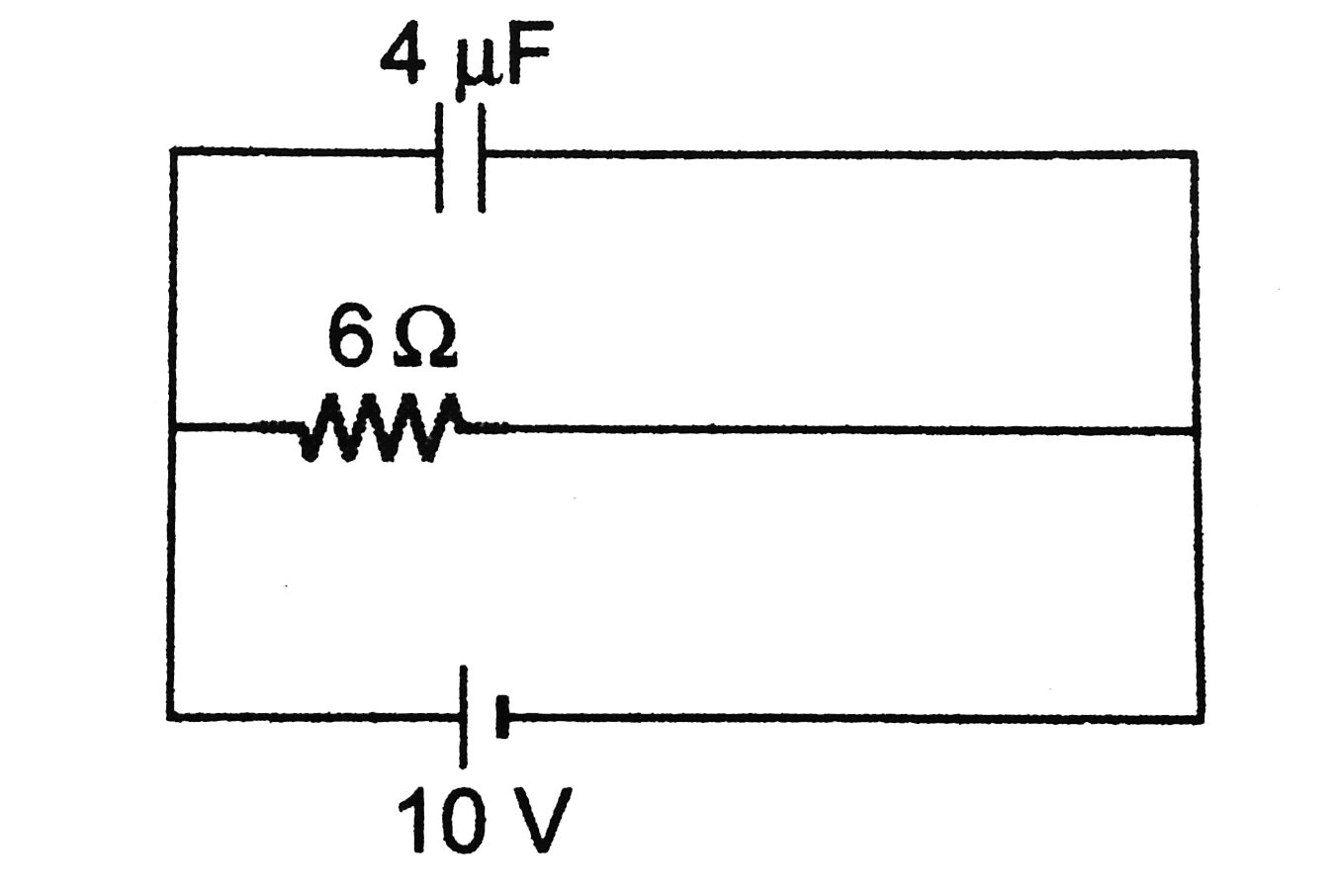
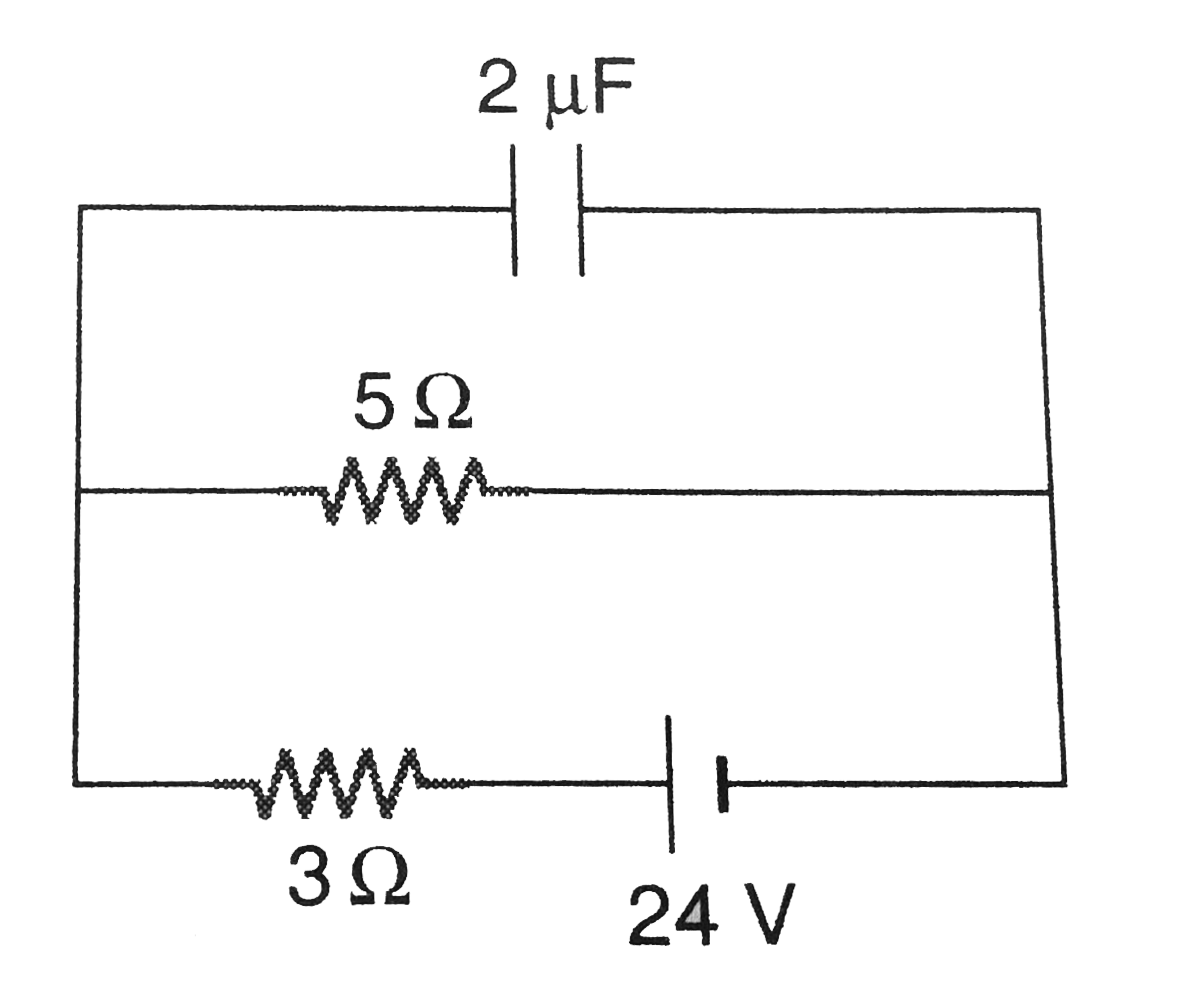
Find the charge stored in the capacitor.
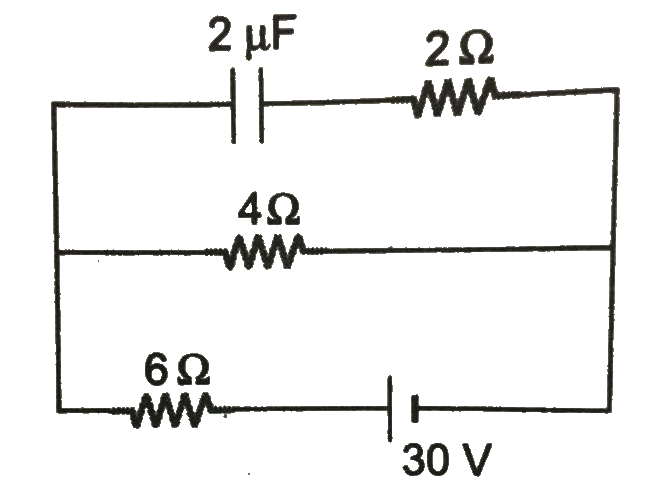
View SolutionIn LC circuit the maximum charge in capacitor is q0. Then maximum value of rate of change of current is
View SolutionDisplacement current is maximum during charging of capacitor. When charge on the capacitor is
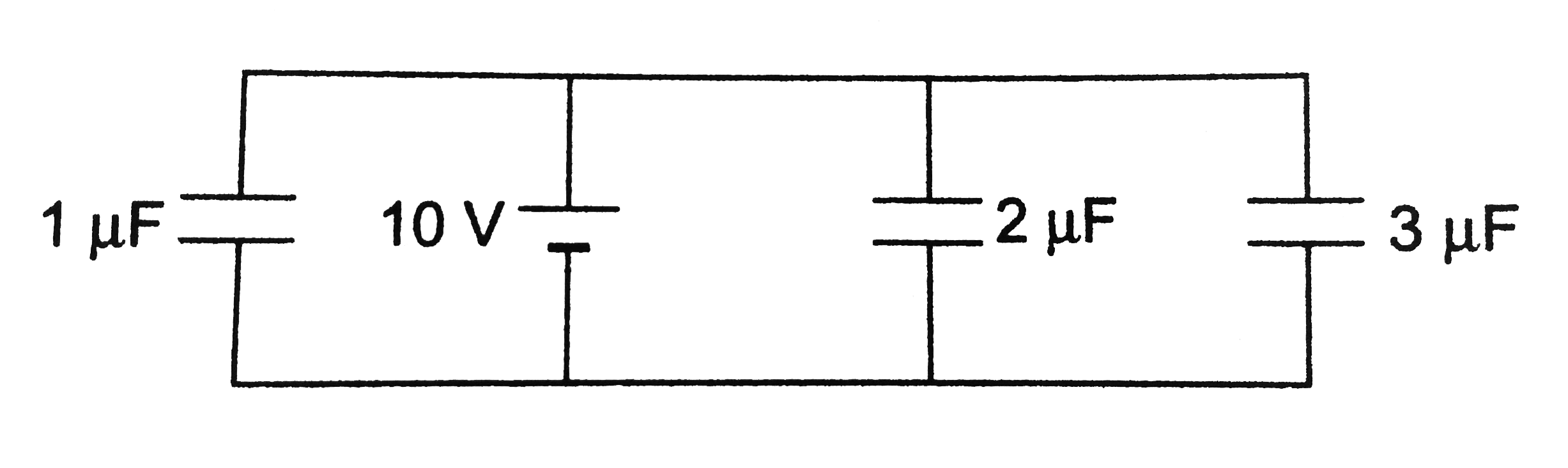
View SolutionFind the charged stored in all the capacitors
View SolutionIn a charged capacitor, the energy resides in
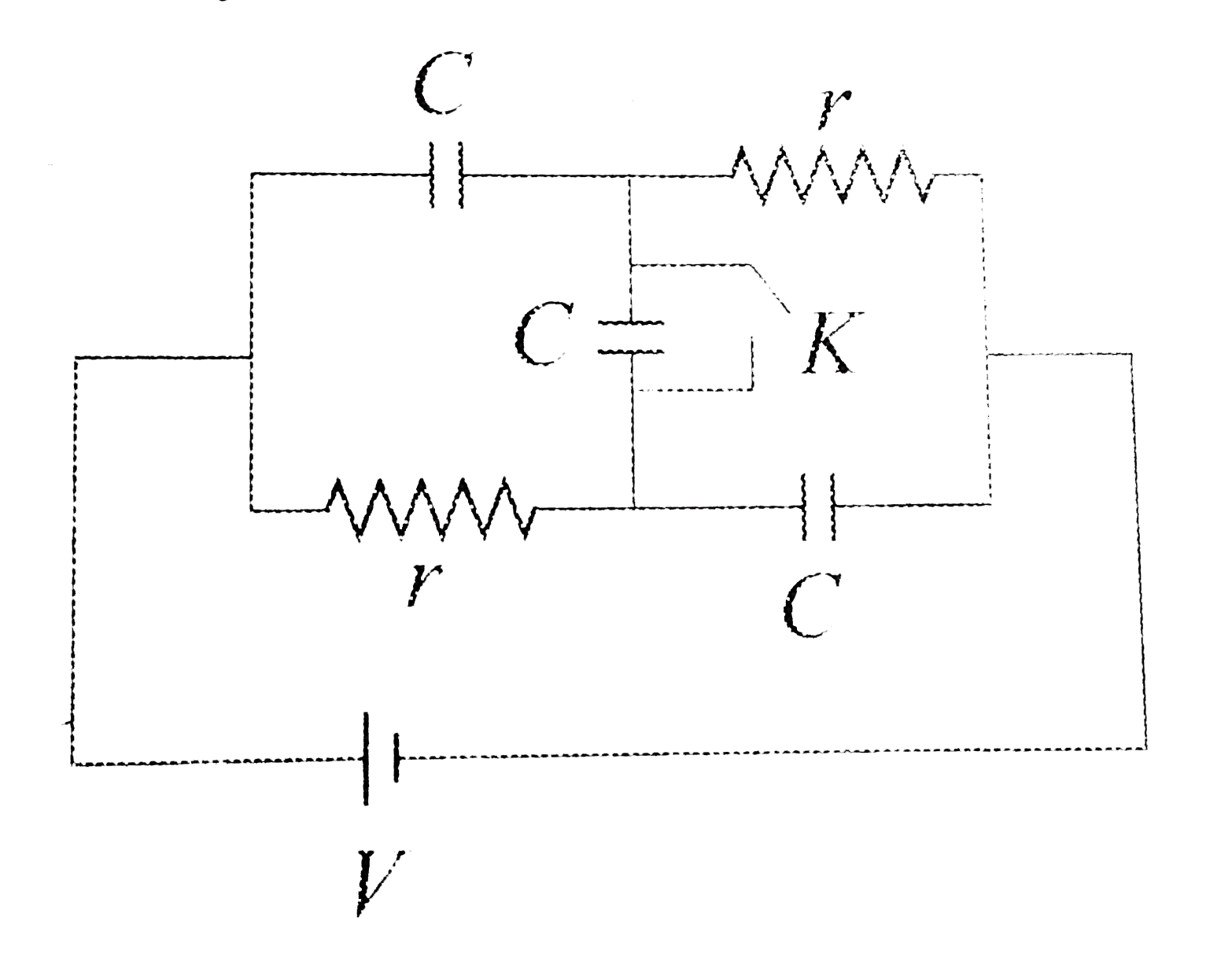
View SolutionIn the circuit what is the change of total electrical energy stored in the capacitors when the key is pressed ?
View SolutionDetermine to charge on the capacitor in the following circuit :
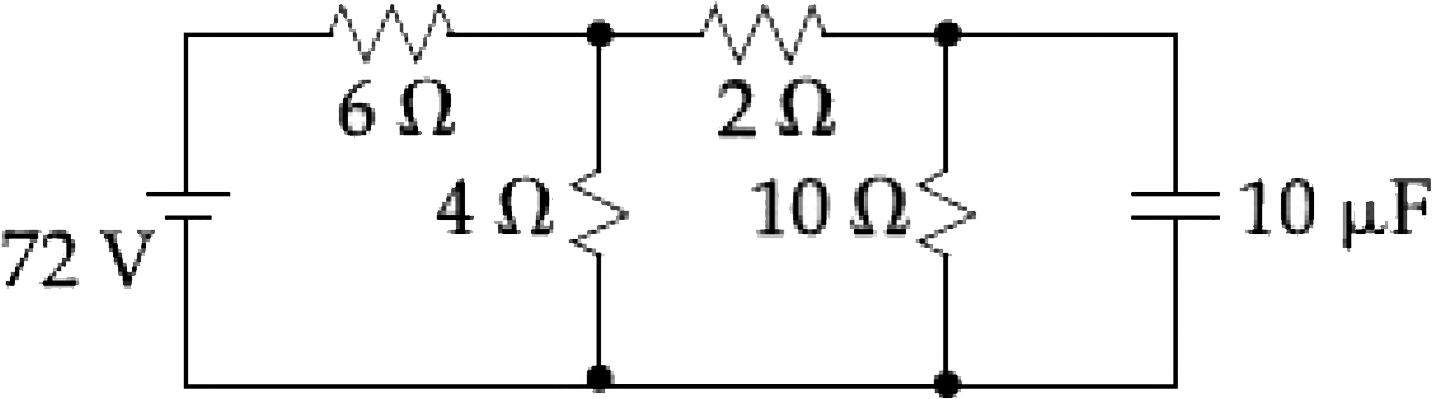
View Solution