For a concave mirror, paraxial rays are focused at distance R2 from pole and marginal rays are focused at distance x from pole, then (R is radius of curvature)
x=R2
x=−f
x>R2
x<R2
The correct Answer is:B
To solve the problem, we need to analyze the behavior of paraxial and marginal rays in a concave mirror.
1. Understanding the Focus of a Concave Mirror:
- The focal point (F) of a concave mirror is located at a distance of R/2 from the pole (P) of the mirror, where R is the radius of curvature.
2. Identifying Paraxial Rays:
- Paraxial rays are rays that are close to the principal axis and make small angles with it. For these rays, the focal point is at F=R/2.
3. Identifying Marginal Rays:
- Marginal rays are rays that are at the edge of the mirror and can be considered as rays that travel parallel to the principal axis. These rays converge at a different point compared to paraxial rays.
4. Finding the Distance for Marginal Rays:
- For a concave mirror, marginal rays focus at the same distance as the focal point but on the opposite side of the mirror. Therefore, if the focal point is at R/2 on the right side of the mirror, the marginal rays will focus at x=−F.
5. Substituting the Value of F:
- Since F=R/2, we can substitute this into the equation for marginal rays:
x=−F=−R2
6. Conclusion:
- Thus, the distance x from the pole for marginal rays is given by:
x=−R2
Final Answer:
The distance x from the pole for marginal rays is x=−R2.
---
- The focal point (F) of a concave mirror is located at a distance of R/2 from the pole (P) of the mirror, where R is the radius of curvature.
- Paraxial rays are rays that are close to the principal axis and make small angles with it. For these rays, the focal point is at F=R/2.
- Marginal rays are rays that are at the edge of the mirror and can be considered as rays that travel parallel to the principal axis. These rays converge at a different point compared to paraxial rays.
- For a concave mirror, marginal rays focus at the same distance as the focal point but on the opposite side of the mirror. Therefore, if the focal point is at R/2 on the right side of the mirror, the marginal rays will focus at x=−F.
- Since F=R/2, we can substitute this into the equation for marginal rays:
x=−F=−R2
- Thus, the distance x from the pole for marginal rays is given by:
x=−R2
The distance x from the pole for marginal rays is x=−R2.
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSIGNMENT (SECTION - A)39videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSIGNMENT (SECTION - B)35videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - EXAMPLE75videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)4videos
PHYSICAL WORLD
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )EXERCISE - Assignment SECTION - D (Assertion & reason type Question)10videos
SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )
Similar Questions
A concave mirror forms an image of the sun at a distance of 12 cm from it.
View SolutionA transparent thin film of uniform thickness and refractive index n1=1.4 is coated on the convex spherical surface of radius R at one end of a long solid glass cylinder of refractive index n2=1.5, as shown in the figure. Rays of light parallel to the axis of the cylinder traversing through the film from air to glass get focused at distance f1 from the film, while rays of light traversing from glass to air get focused at distance f2 from the film, Then
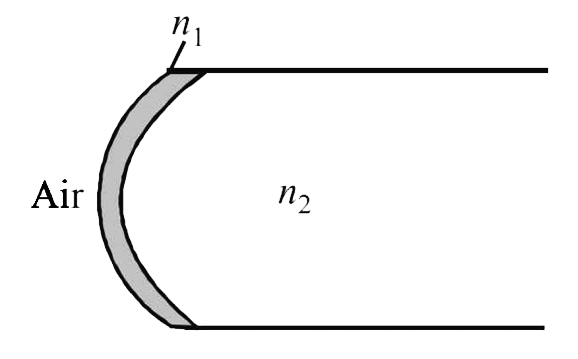 View Solution
View SolutionA convex mirror of radius of curvature 1.6m has an object placed at a distance of 1m from it. The image is formed at a distance of
View SolutionA source of light is at 10 cm from a convex mirror and is then moved to a distance of 2 cm from the mirror. How much does the image move if the radius of curvature of the mirror is 4.8 cm?
View SolutionThe figure ,shows a transparent sphere of radiusRand refractive indexμ.An object Ois placed at a distance x from the pole of the first surface so that a real image is formed at the pole of the exactly opposite surface.
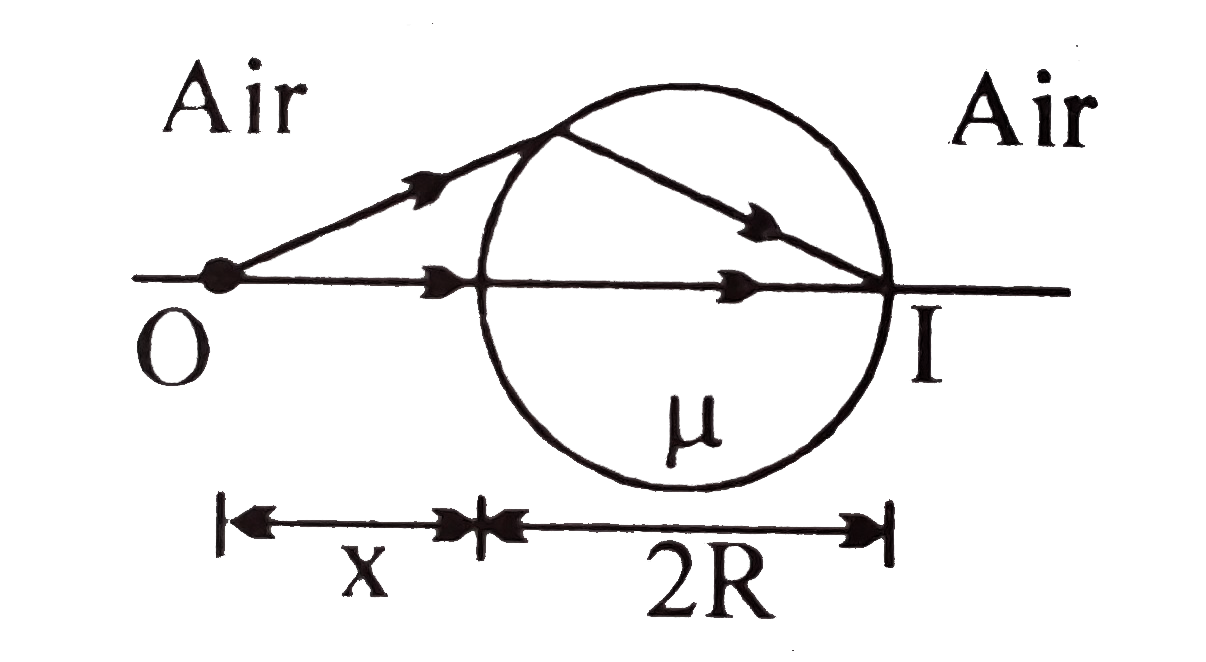 if an object is Placed at a distanceR from the pole of first surface ,then the real image is formed at a distance Rfrom thepole of the second surface.The refractive indexμ of the spher is given byView Solution
if an object is Placed at a distanceR from the pole of first surface ,then the real image is formed at a distance Rfrom thepole of the second surface.The refractive indexμ of the spher is given byView SolutionIn the given figure a parallel beam of ligth is incident on the upper part of a prism of angle 1.8∘ and R.I.3/2 . The light coming out of the prism falls on a concave mirror of radius of curvature 20cm. The distance of the point (where the rays are focused after reflrction from the mirror) from the pricipal axis is: [use π=3.14]
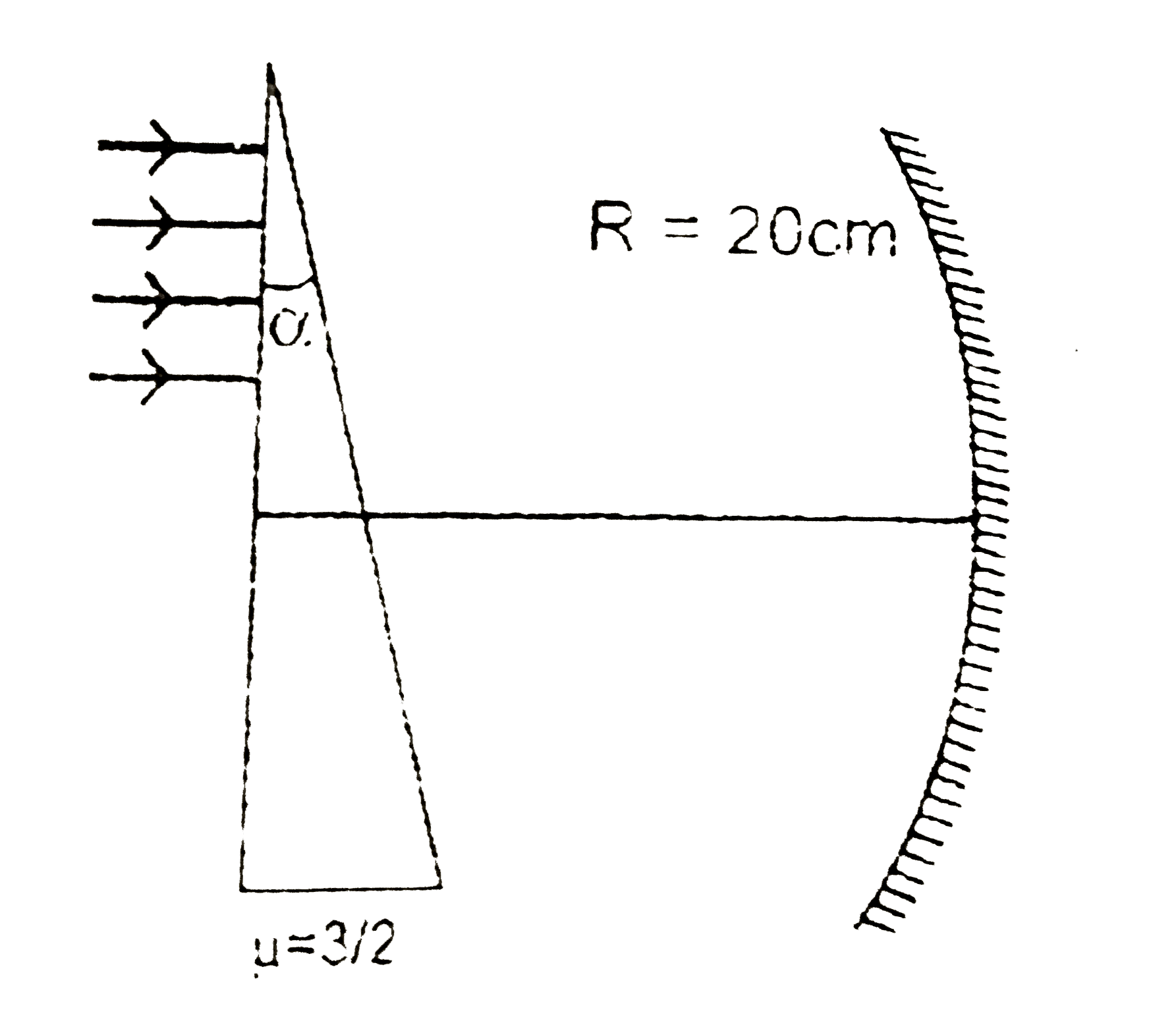 View Solution
View SolutionA beam of light coming from infinty is passing through a biconvex lens having radius of curvature R=20cm of each surface, if focused at a certain distance from lens. Find the radius of curvature of emergent wave front from lens : (μ≤ns=1.5)
View SolutionA real point object is kept on the pricipal axis of a concave mirror (radius of curvature =20cm) at a distance 60 cm from the pole. Take pole as origin and direction of incident light as positive direction of X axis. Y axis is perpendicular to the principal axis. Select correct statement(s).
View SolutionThe minimum sum of the distances of a real object and a real image from a concave mirror of radius of curvature 20cm is:
View SolutionA concave mirror having a radius of curvature 40 cm is placed in front of an illuminated point source at a distance of 30 cm from it. Find the location of the image.
View Solution

