A convex lens forms a real image 16 mm long on a screen. If the lens is shifted to a new position without disturbing the object or the screen then again a real image of length 81 mm is formed. The length of the object must be
48.5 mm
36 mm
6 mm
72 mm
The correct Answer is:B
To solve the problem step by step, we will use the concept of magnification in optics.
Step 1: Understand the problem
We have a convex lens that forms two real images of different lengths on a screen when the lens is shifted. The lengths of the images are given as:
- Length of the first image (l1) = 16 mm
- Length of the second image (l2) = 81 mm
We need to find the length of the object (L).
Step 2: Write the magnification formulas
The magnification (m) produced by a lens is given by the formula:
m=Height of ImageHeight of Object
For the first image:
m1=l1L=16L
For the second image:
m2=l2L=81L
Step 3: Use the displacement law
According to the displacement law of lenses, when the lens is shifted, the product of the magnifications of the two images formed remains equal to 1:
m1×m2=1
Substituting the expressions for m1 and m2:
(16L)×(81L)=1
Step 4: Simplify the equation
This simplifies to:
16×81L2=1
Step 5: Solve for L
Now, we can solve for L2:
L2=16×81
Calculating 16×81:
16×81=1296
Thus,
L2=1296
Taking the square root of both sides:
L=√1296=36mm
Final Answer
The length of the object is L=36mm.
---
We have a convex lens that forms two real images of different lengths on a screen when the lens is shifted. The lengths of the images are given as:
- Length of the first image (l1) = 16 mm
- Length of the second image (l2) = 81 mm
The magnification (m) produced by a lens is given by the formula:
m=Height of ImageHeight of Object
m1=l1L=16L
m2=l2L=81L
According to the displacement law of lenses, when the lens is shifted, the product of the magnifications of the two images formed remains equal to 1:
m1×m2=1
(16L)×(81L)=1
This simplifies to:
16×81L2=1
Now, we can solve for L2:
L2=16×81
16×81=1296
L2=1296
L=√1296=36mm
The length of the object is L=36mm.
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSIGNMENT (SECTION - D)16videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSIGNMENT (SECTION - B)35videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)4videos
PHYSICAL WORLD
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )EXERCISE - Assignment SECTION - D (Assertion & reason type Question)10videos
SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )
Similar Questions
A convex lens forms an image of an object on a screen 30 cm from the lens. When the lens is moved 90 cm towards the object, then the image is again formed on the screen. Then, the focal length of the lens is
View SolutionA lens placed between a candle and a fixed screen forms a real triply magnified image of the candle on the screen. When the lens is moved away form the candle by 0.8m without changing the position of the candle, a real image one-third the size of the candle is formed on the screen. Determine the focal length of the lens.
View SolutionA convex lens forms an image of an object on a screen. The height of the image is 9 cm . The lens is now displaced until an image is again obtained on the screen. Then height of this image is 4 cm . The distance between the object and the screen is 90 cm.
View SolutionA convex lens produces an image of a real object on a screen with a magnification of 1/2. When the lens is moved 30 cm away from the object, the magnification of the image on the screen is 2. The focal length of the lens is
View SolutionFormation of real image using a biconves lens is shown below:
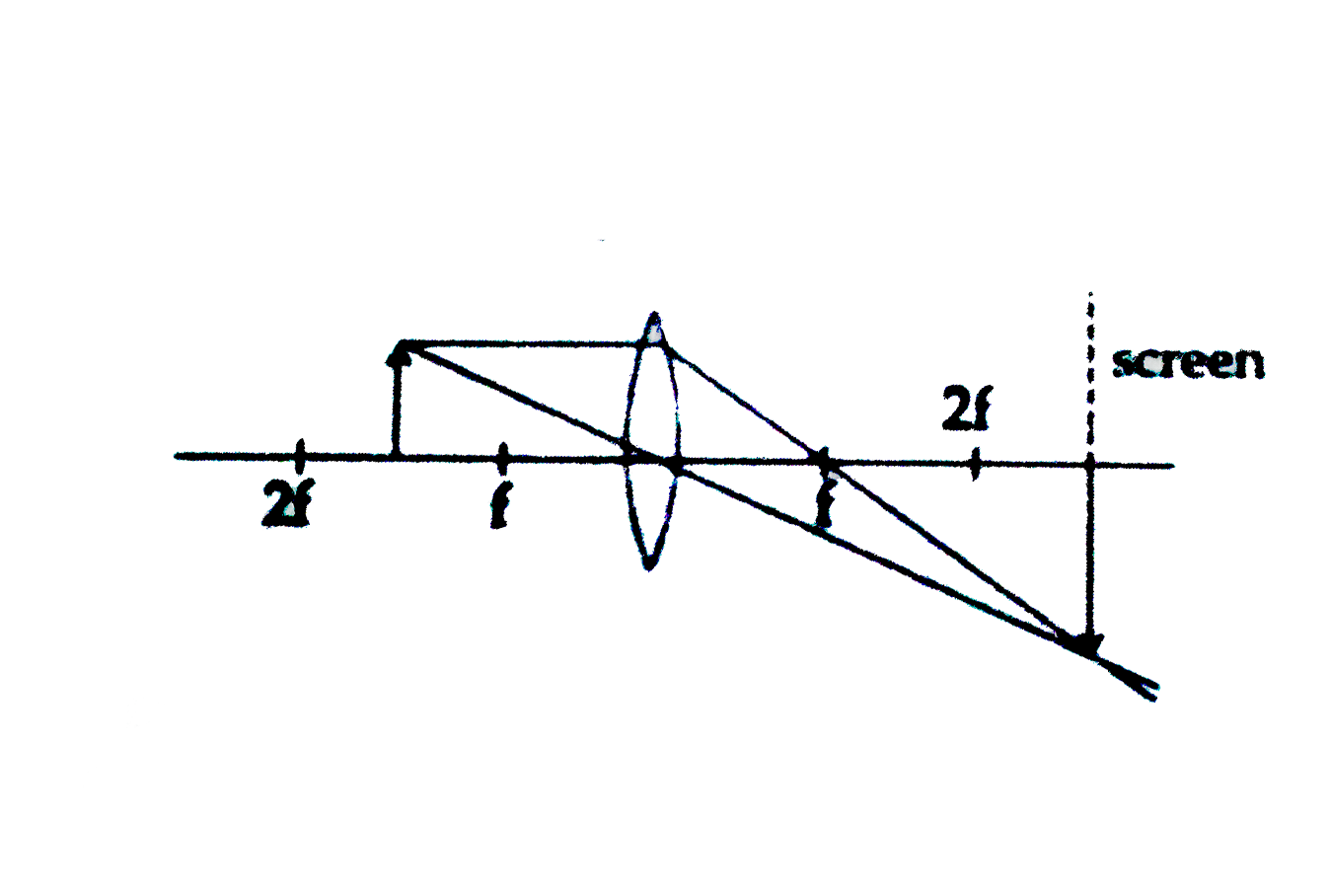
If the whole set up is immersed in water without disturbing the object and the screen positions, what will one observe on the secreen ?View SolutionA double convex lens forms a real image of an objectt on a screen which is fixed. Now lens is given a constant velocity v=1m/s along its axis and away from the screen for the purpose of forming image always on the screen the object is also required to be given appropriate velocity . Find the velocity (in m/s) of the object at the instant its size is doubled the size of the image.
View SolutionA convex lens forms a real image of an object for its two different positions on a screen. If height of the image in both the cases be 8 cm and 2 cm , then height of the object is
View SolutionA convex lens produces a real image m times the size of the object. What will be the distance of the object from the lens ?
View SolutionA convex forms a real image of a poinnt object placed on its principal axis. If the upper half of the lens is painted black
View SolutionA convex lens of focal length 30 cm forms a real image three times larger than the object on a screen. Object and screen are moved until the image becomes twice the size of the object. If the shift of the object is 6 cm. the shift of screen is (7x) cm. find value of x
View Solution

