The focal length of a thin lens in vacuum is . If the material of the lens has , its focal length when immersed in water of refractive index will be.
f
f
2f
4f
The correct Answer is:D
To find the focal length of a thin lens when it is immersed in water, we can use the lens maker's formula. The formula relates the focal length of a lens to the refractive indices of the lens material and the surrounding medium.
1. Understanding the Lens Maker's Formula:
The lens maker's formula is given by:
1F=(μlμs−1)(1R1−1R2)
where:
- F is the focal length of the lens,
- μl is the refractive index of the lens material,
- μs is the refractive index of the surrounding medium,
- R1 and R2 are the radii of curvature of the lens surfaces.
2. Case 1: Lens in Vacuum:
For the lens in vacuum, the refractive index of the surrounding medium μs=1 and the refractive index of the lens material μl=32. Thus, we can write:
1F=(3/21−1)(1R1−1R2)
Simplifying this gives:
1F=(12)(1R1−1R2)
3. Case 2: Lens Immersed in Water:
Now, when the lens is immersed in water, the refractive index of the surrounding medium μs=43. The formula for the new focal length F′ becomes:
1F′=(3/24/3−1)(1R1−1R2)
Simplifying 3/24/3:
3/24/3=3×32×4=98
Thus, we have:
1F′=(98−1)(1R1−1R2)=(98−88)(1R1−1R2)=18(1R1−1R2)
4. Relating Focal Lengths:
Now, we can relate 1F and 1F′:
1F′=18(1R1−1R2)=14(12(1R1−1R2))=14⋅1F
Therefore, we have:
F′=4F
Final Answer:
The focal length of the lens when immersed in water is:
F′=4F
The lens maker's formula is given by:
1F=(μlμs−1)(1R1−1R2)
where:
- F is the focal length of the lens,
- μl is the refractive index of the lens material,
- μs is the refractive index of the surrounding medium,
- R1 and R2 are the radii of curvature of the lens surfaces.
For the lens in vacuum, the refractive index of the surrounding medium μs=1 and the refractive index of the lens material μl=32. Thus, we can write:
1F=(3/21−1)(1R1−1R2)
Simplifying this gives:
1F=(12)(1R1−1R2)
Now, when the lens is immersed in water, the refractive index of the surrounding medium μs=43. The formula for the new focal length F′ becomes:
1F′=(3/24/3−1)(1R1−1R2)
Simplifying 3/24/3:
3/24/3=3×32×4=98
Thus, we have:
1F′=(98−1)(1R1−1R2)=(98−88)(1R1−1R2)=18(1R1−1R2)
Now, we can relate 1F and 1F′:
1F′=18(1R1−1R2)=14(12(1R1−1R2))=14⋅1F
Therefore, we have:
F′=4F
The focal length of the lens when immersed in water is:
F′=4F
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSIGNMENT (SECTION - D)
16 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSIGNMENT (SECTION - B)
35 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
4 Videos
- SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )EXERCISE - Assignment SECTION - D (Assertion & reason type Question)
10 Videos
Similar Questions
Focal length of a lens in air is f. Refractive index of the lens is μ. Focal length changes to f1 if lens is immersed in a liquid of refractive index μ2 and it becomes f2 if the lens is immersed in a liquid of refractive index 2μ. Then
View SolutionA convex lens of refractive index 1.5 has a focal length of 20 cm in air. Calculate the change in its focal length when it is immersed in water of refractive index 4/3.
View SolutionThe focal length of a thin convex-lens is 30cm. At a distance of 10 cm from the lens there is a plane refracting surface fo refractive inidex 3/2 Where will parallel rays incident on lens converge?
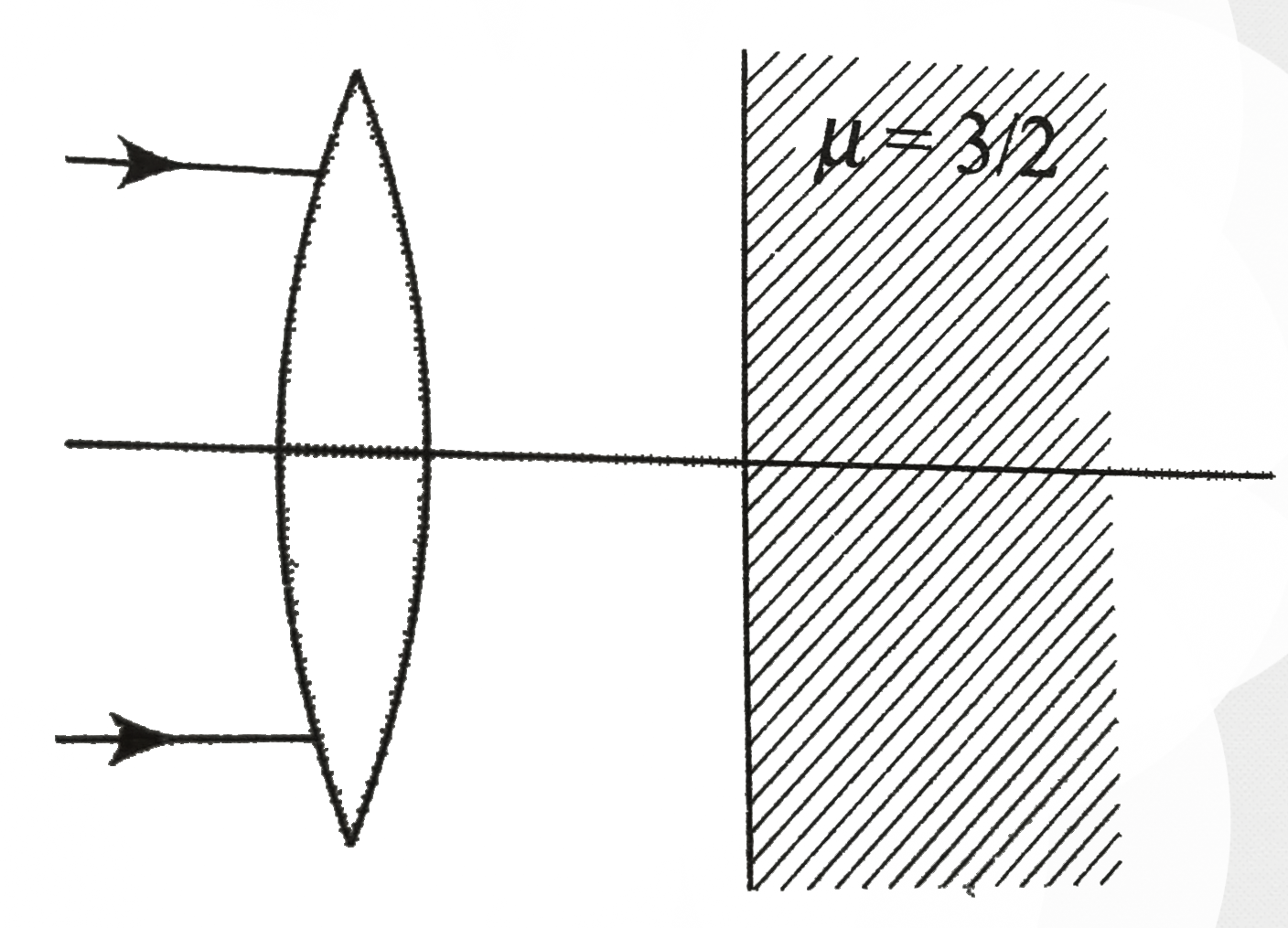
View SolutionFocal length of a convex lens of refractive index 1.5 is 2 cm. Focal length of lens when immersed in a liquid of refractive index 1.25 will be
View SolutionA converging lens has a focal length of 20cm in air. It is made of a material of refractive index 1.6. If it is immersed in a liquid of refractive index 1.3, what will be its new foacl length ?
View SolutionA convex lens of focal length 12cm is made of glass of μ=32. What will be its focal length when immersed in liquid of μ=54 ?
View SolutionIf a thin lens is dipped in water, does its focal length change ?
View SolutionThe focal length of lens of refractive index 1.5 in air is 30cm When it is immersed in water of refractive index 43 ,then its focal length will be
View SolutionFocal length of a thin convex lens is 30 cm. At distance of 10 cm from the lens there is a plan refracting surface of refractive index 3/2. Where w the parallel rays incident on lens converge ?
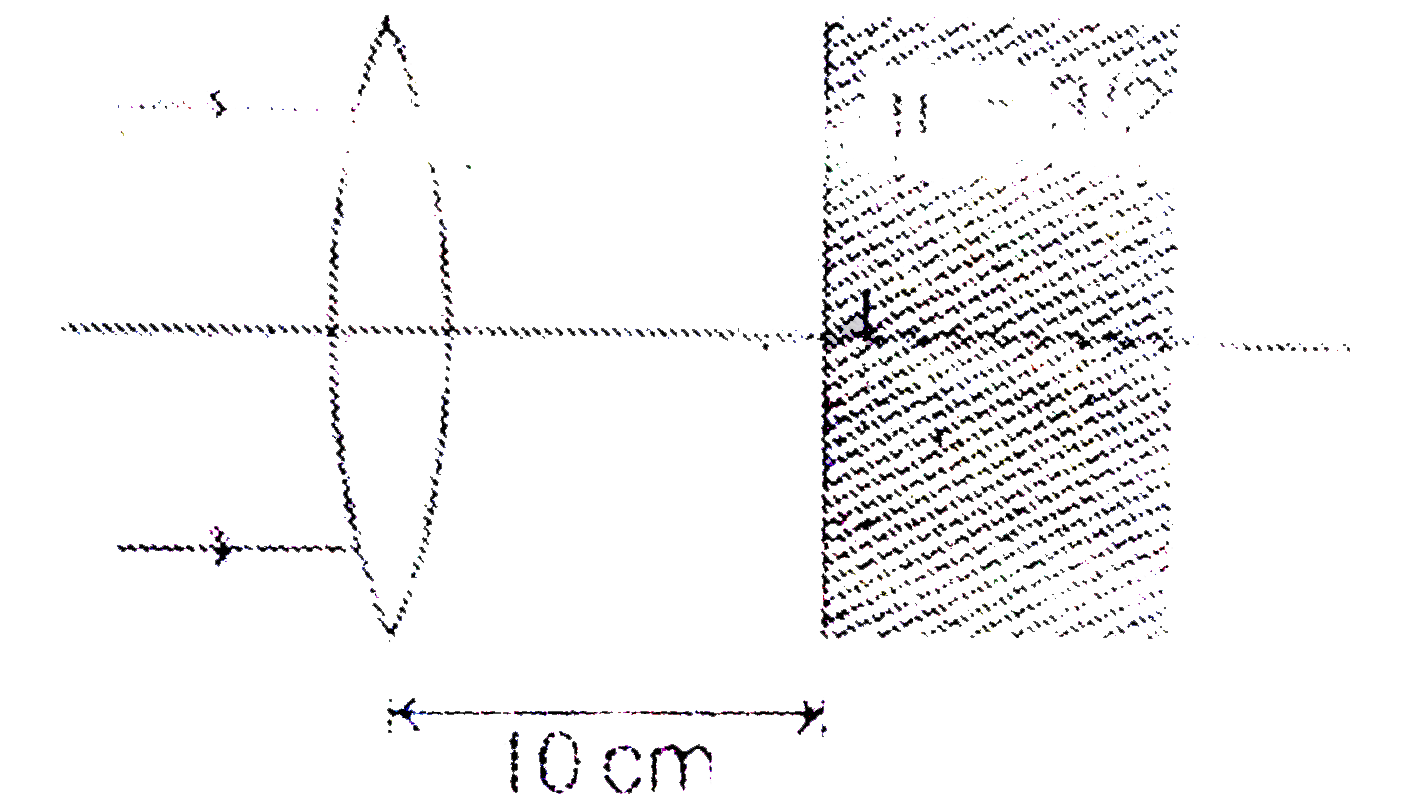
View SolutionA concave lens with unequal radii of curvature made of glass (μg=1.5) has a focal length of 40cm. If it is immersed in a liquid of refractive index μ1=2, then
View Solution
