Which of the following may be representing graph between number of scattered particles detected (N) and scattering angle(θ) in Rutherford's experiment?




The correct Answer is:B
To determine the graph representing the relationship between the number of scattered particles detected (N) and the scattering angle (θ) in Rutherford's experiment, we can follow these steps:
Step 1: Understand the Relationship
In Rutherford's gold foil experiment, the number of particles scattered at an angle θ is inversely related to the sine of the angle raised to the fourth power. Mathematically, this can be expressed as:
N∝1sin4(θ)
Step 2: Analyze the Behavior of the Function
As θ approaches 0, sin(θ) approaches 0, which means that sin^4(θ) also approaches 0. Consequently, 1sin4(θ) approaches infinity. Therefore, at θ = 0, the number of detected particles (N) becomes infinitely large.
Step 3: Examine the Range of θ
The angle θ varies from 0 to π (0 to 180 degrees). As θ increases from 0 to π, sin(θ) increases from 0 to 1 and then decreases back to 0. This means that sin4(θ) will initially increase, leading to a decrease in N until it reaches a maximum point, after which N will decrease again as θ approaches π.
Step 4: Sketch the Graph
1. At θ = 0, N approaches infinity.
2. As θ increases from 0 to π/2, N decreases.
3. At θ = π/2, N reaches a minimum value.
4. As θ continues to increase towards π, N increases again but remains finite.
The graph will start from infinity at θ = 0, decrease to a minimum at θ = π/2, and then increase again as θ approaches π, but will not reach infinity again.
Step 5: Conclusion
The graph representing the relationship between the number of scattered particles detected (N) and the scattering angle (θ) will show a trend starting from infinity, decreasing to a minimum, and then increasing again, resembling a curve that approaches the axes but never touches them.
In Rutherford's gold foil experiment, the number of particles scattered at an angle θ is inversely related to the sine of the angle raised to the fourth power. Mathematically, this can be expressed as:
N∝1sin4(θ)
As θ approaches 0, sin(θ) approaches 0, which means that sin^4(θ) also approaches 0. Consequently, 1sin4(θ) approaches infinity. Therefore, at θ = 0, the number of detected particles (N) becomes infinitely large.
The angle θ varies from 0 to π (0 to 180 degrees). As θ increases from 0 to π, sin(θ) increases from 0 to 1 and then decreases back to 0. This means that sin4(θ) will initially increase, leading to a decrease in N until it reaches a maximum point, after which N will decrease again as θ approaches π.
1. At θ = 0, N approaches infinity.
2. As θ increases from 0 to π/2, N decreases.
3. At θ = π/2, N reaches a minimum value.
4. As θ continues to increase towards π, N increases again but remains finite.
The graph representing the relationship between the number of scattered particles detected (N) and the scattering angle (θ) will show a trend starting from infinity, decreasing to a minimum, and then increasing again, resembling a curve that approaches the axes but never touches them.
Similar Questions
Knowledge Check
Which of the following inequalities represents the graph shown below on the real number line ?
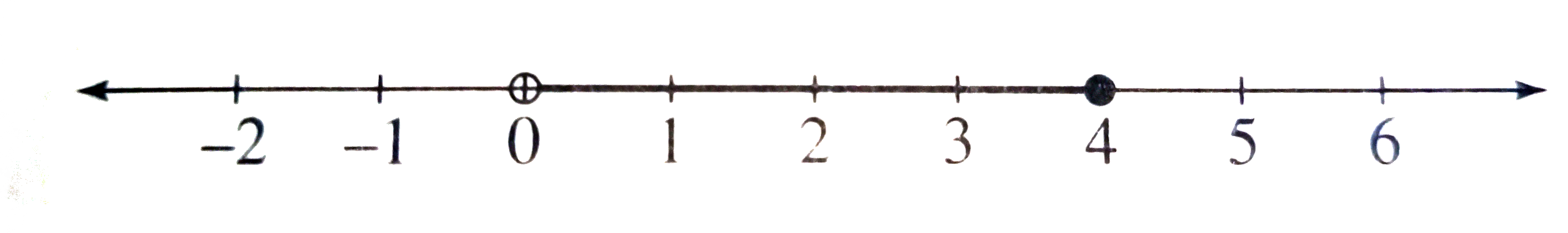 A0<x<5B0<x≤4C−2<x≤4D1≤x≤4
A0<x<5B0<x≤4C−2<x≤4D1≤x≤4Which of the following graph represents correct graph for SN1 reaction :
A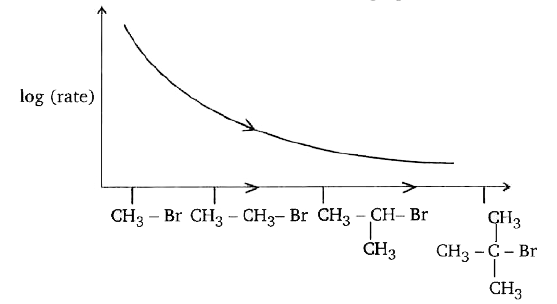 B
B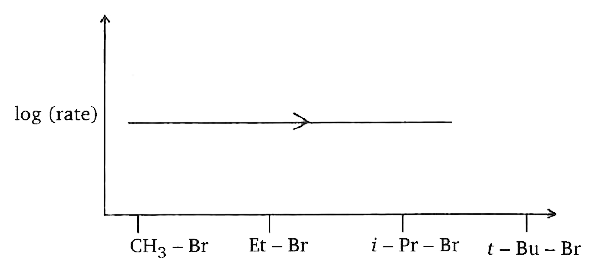 C
C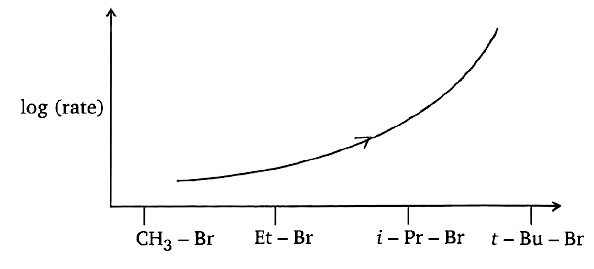 D
D
Which of the following graphs cannot possibly represent one dimensional motion of a particle?
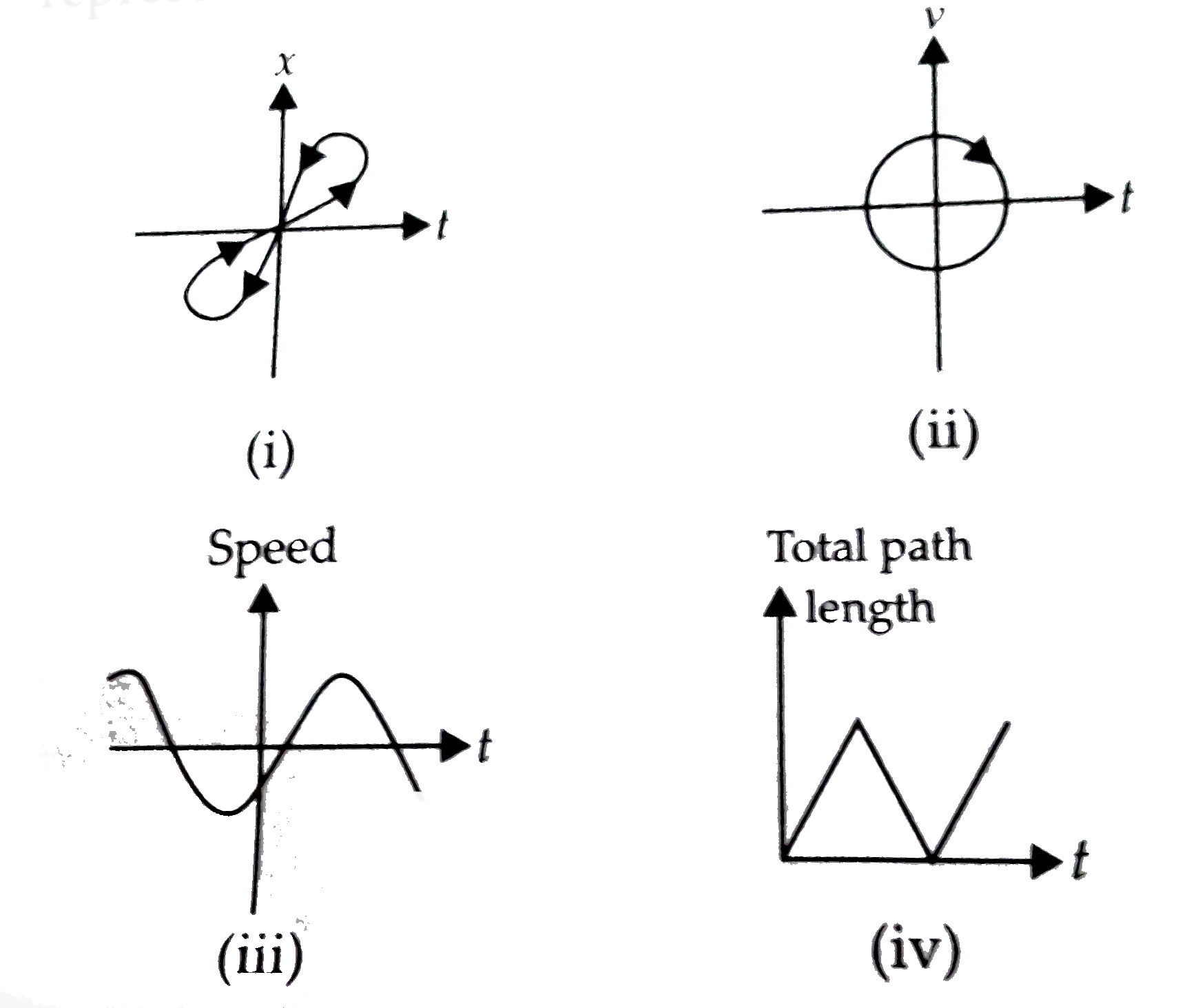 A(i) and (ii)B(ii) and (iii)C(i), (ii) and (iii)DAll four
A(i) and (ii)B(ii) and (iii)C(i), (ii) and (iii)DAll four
Which of the following colour is scattered most is atmosphere?
View SolutionWhich of the following graphs represents the motion of a particle moving with constant velocity?
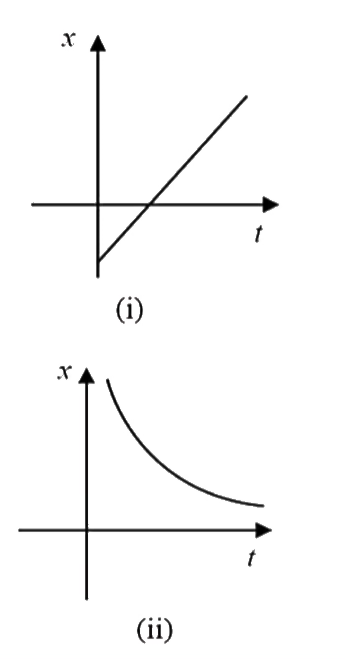
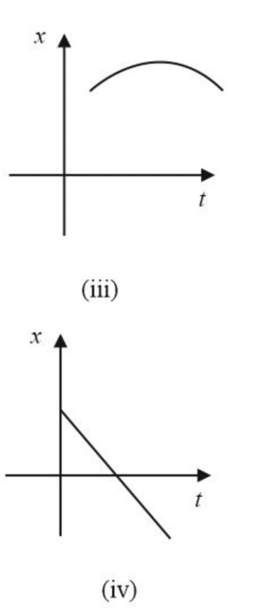
View SolutionWhich of the following graphs cannot possibly represent one dimensional motion of a particle?

View SolutionThe number of α-particless scattered per unit area N (θ) at scattering angle θ varies inversely as
View SolutionWhich of the following graphs represents the position time-graph of a particle moving with negative velocity?
View Solution

